5 Key Measures of Center: Worksheet Answers

The concept of measures of center plays a crucial role in descriptive statistics, offering insightful summaries that help us understand the general tendency or typical value within a dataset. This understanding can significantly enhance our ability to interpret data effectively across various domains like business analytics, health sciences, and social research.
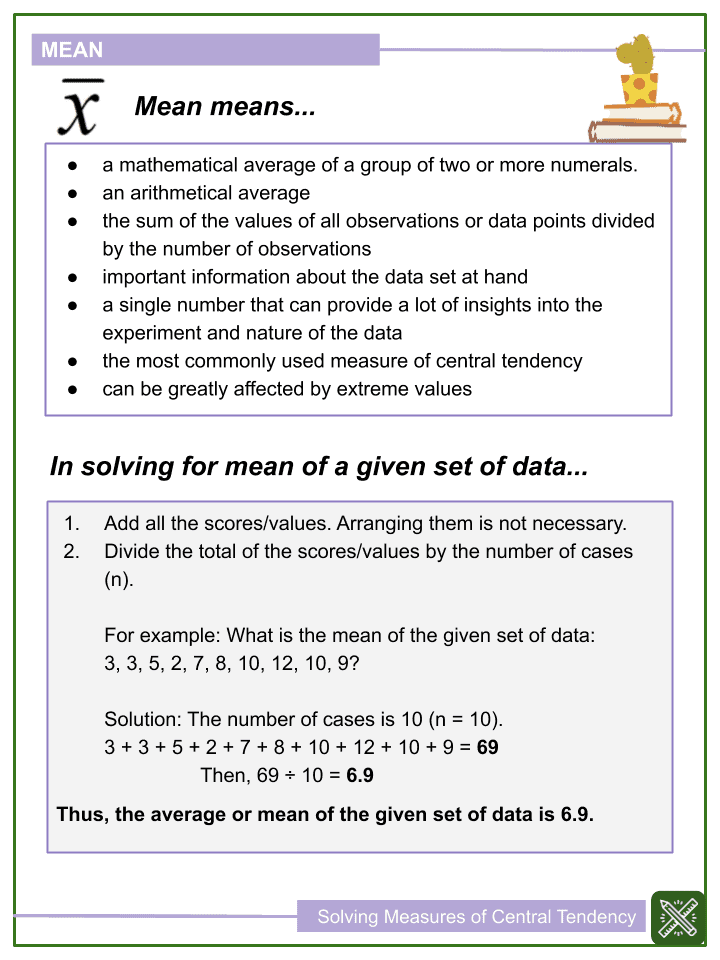
1. Mean

The mean, commonly known as the average, is calculated by adding all data values and dividing by the total number of observations. It represents the overall balance point of the dataset:
- Formula:
Mean = (Sum of all values) / (Number of observations) - Use Case: Ideal for normally distributed data without significant outliers. For instance, calculating the average score of students in a class.
💡 Note: The mean is highly sensitive to outliers; a single extreme value can skew the result.
2. Median

The median is the middle value of a dataset when arranged in ascending order:
- If the dataset has an odd number of observations, the median is the middle number.
- If even, it’s the average of the two central numbers.
- Use Case: Excellent for skewed distributions or when there are outliers. Example: Median house prices in a neighborhood.
3. Mode
The mode is the value that appears most frequently in the dataset:
- Dataset can have no mode, one mode (unimodal), or multiple modes (multimodal).
- Use Case: Suitable for categorical data or when identifying the most common value is relevant, e.g., the favorite flavor of ice cream in a survey.
🌟 Note: Unlike mean and median, mode doesn’t require numerical data, making it versatile for both qualitative and quantitative datasets.
4. Midrange

The midrange is the arithmetic mean of the minimum and maximum values in the dataset:
- Formula:
Midrange = (Min + Max) / 2 - Use Case: Quick estimation of data spread. Best for understanding extremes, not the central tendency, like the midrange salary in a company.
5. Trimmed Mean

The trimmed mean is similar to the mean but excludes a specified percentage of the lowest and highest values before calculation:
- Formula: Remove 5%, 10%, or any other agreed percentage from both ends, then calculate the mean.
- Use Case: Useful when dealing with datasets that have outliers that might significantly affect the regular mean, e.g., trim 10% of test scores from both ends.
Each measure of center provides unique insights into data, and choosing the right one depends on several factors:
- The distribution of your data.
- The presence of outliers.
- The goal of your analysis.
Wrapping up, understanding measures of center is essential for descriptive statistics. Each measure—mean, median, mode, midrange, and trimmed mean—offers a different lens through which we can view and interpret data. Their application helps analysts make informed decisions, predict outcomes, and summarize complex datasets succinctly. Remember, the choice between these measures often depends on the nature of the data and the specific needs of your analysis. No single measure can tell the whole story by itself, making it crucial to use them in conjunction with other statistical tools to gain a comprehensive understanding of data trends and distributions.
Why is the median less affected by outliers than the mean?

+
The median is the middle value in a sorted dataset. Outliers, which are extreme values, do not affect the position of the middle value. The mean, however, is calculated by summing all values, making it more sensitive to these extreme values.
When should I use the mode instead of the mean?

+
Use the mode when dealing with categorical data or when you want to identify the most common value in your dataset. The mean is less informative in such cases, as it requires numerical values to make sense.
What’s the benefit of using a trimmed mean?

+
The trimmed mean reduces the impact of outliers by excluding the most extreme values. This makes it a more robust measure of central tendency, particularly in datasets with a significant number of outliers.
Can I have a midrange in datasets with an even number of observations?

+
Yes, the midrange can be calculated for any dataset by taking the average of the minimum and maximum values, regardless of whether the number of observations is odd or even.
How do you decide which measure of center to use?

+
The choice depends on the data’s distribution, the presence of outliers, and your analysis’s objectives. For symmetric distributions without outliers, the mean works well. With skewness or outliers, the median might be more appropriate. The mode is good for categorical data or when finding the most common value is essential.



