Free Printable Worksheets for Fractions to Decimals Conversion
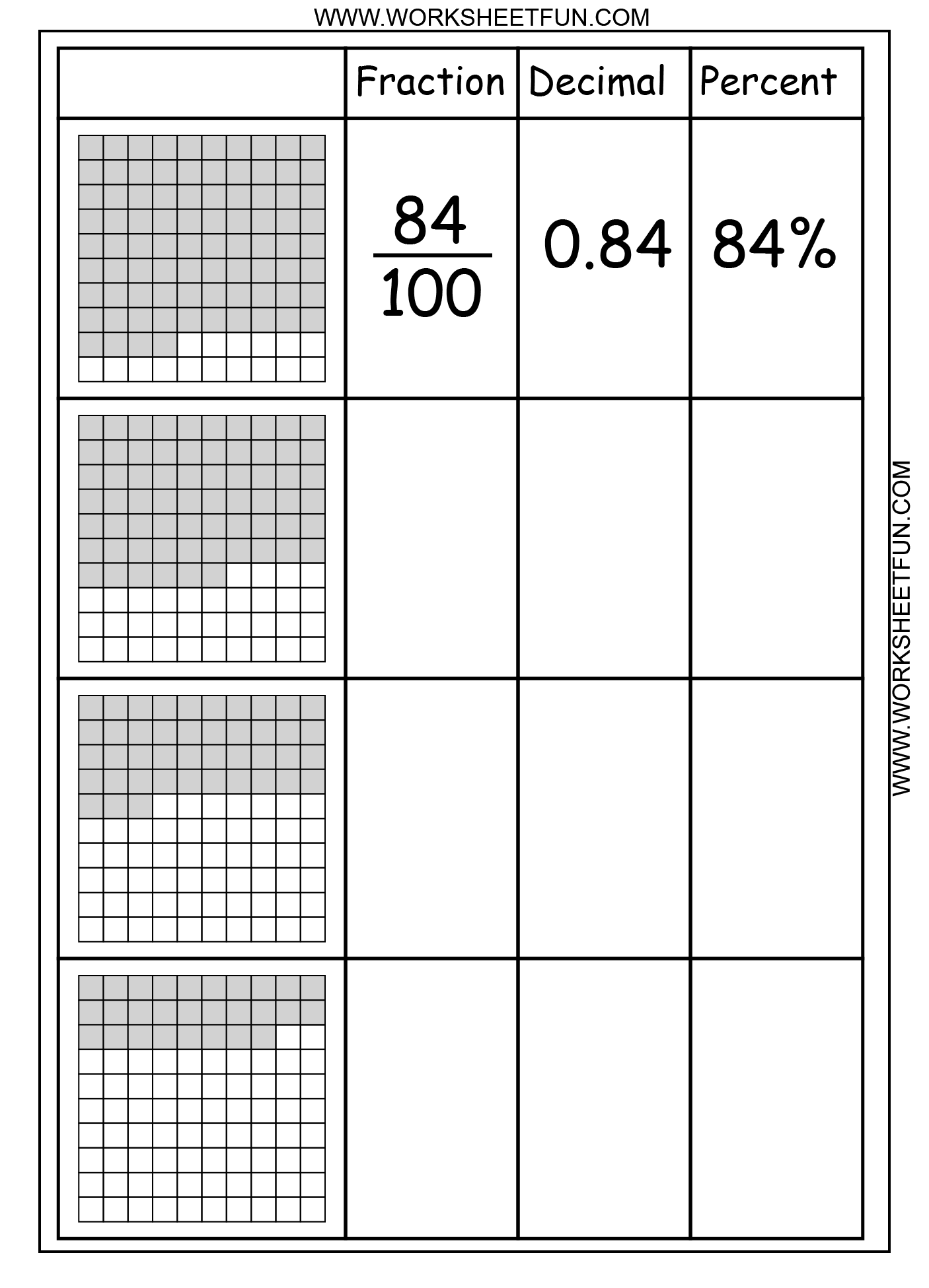
Welcome to our comprehensive guide on converting fractions to decimals, a crucial skill for both students and those wanting to brush up on their math. Whether you're a parent looking for educational resources, a teacher seeking classroom materials, or someone keen on understanding the mathematical process, this post is tailored for you. Here, we will explore various techniques, provide free printable worksheets, and share tips to make this transition from fractions to decimals as smooth as possible.
Theoretical Foundation: Understanding Fractions and Decimals

Fractions are portions of whole numbers, expressed as ratios like 1/4 or 3/7. On the other hand, decimals are the numerical system where numbers are placed after the decimal point to indicate fractions of a whole. Here are key points to understand:
- Fractions: Represent division of a whole into equal parts (numerator/denominator).
- Decimals: Express fractions in a base-10 system where each digit after the decimal point represents a fraction of 10, 100, 1000, etc.
Examples:
- 1/2 = 0.5
- 3/5 = 0.6
Conversion Techniques

Long Division

Long division is a common method for converting fractions to decimals. Here's how you do it:
- Set up the division problem with the numerator as the dividend and the denominator as the divisor.
- Divide the numerator by the denominator, appending zeros to the numerator if necessary until you achieve the desired decimal accuracy or if the division terminates.
Example:
- 1/4: Divide 1 by 4. You get 0.25.
- 5/6: Divide 5 by 6. You get 0.8333333..., which can be rounded to three decimal places as 0.833.
Using Calculators

For quick conversions or verifying your work:
- Input the fraction into a scientific or graphing calculator.
- Convert the display to a decimal using the function keys.
💡 Note: Using a calculator will give you a precise result up to the calculator's display limit, but remember that not all fractions convert to exact decimal values.
Converting Repeating Decimals
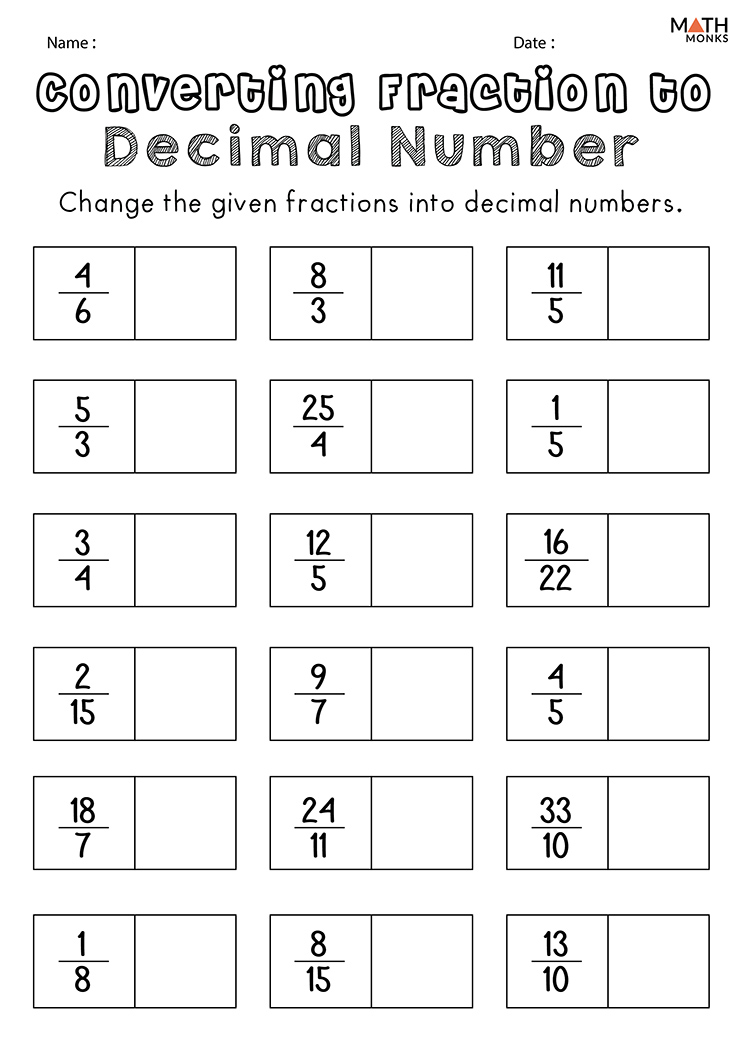
Sometimes, the decimal representation of a fraction can be repeating or infinite. Here are two scenarios:
- Repeating Decimals: The decimal has a repeating sequence (e.g., 1/3 = 0.333...). These are often represented with a vinculum or a bar over the repeating digits (e.g., 0.3).
- Infinite Non-Repeating Decimals: These occur with irrational numbers like π or √2, which cannot be represented exactly by any finite decimal.
Free Printable Worksheets

We've prepared a set of printable worksheets to help you practice fraction to decimal conversion:
| Worksheet Name | Description |
|---|---|
| Fraction to Decimal - Basic | Convert simple fractions like 1/2 or 3/4 to decimals. |
| Long Division Practice | Focus on mastering long division for fraction conversion. |
| Repeating Decimals | Work with repeating decimals and understand their properties. |
| Advanced Fractions | Includes mixed numbers and more complex fractions. |

Each worksheet comes with instructions, exercises, and an answer key for self-assessment.
Practical Applications
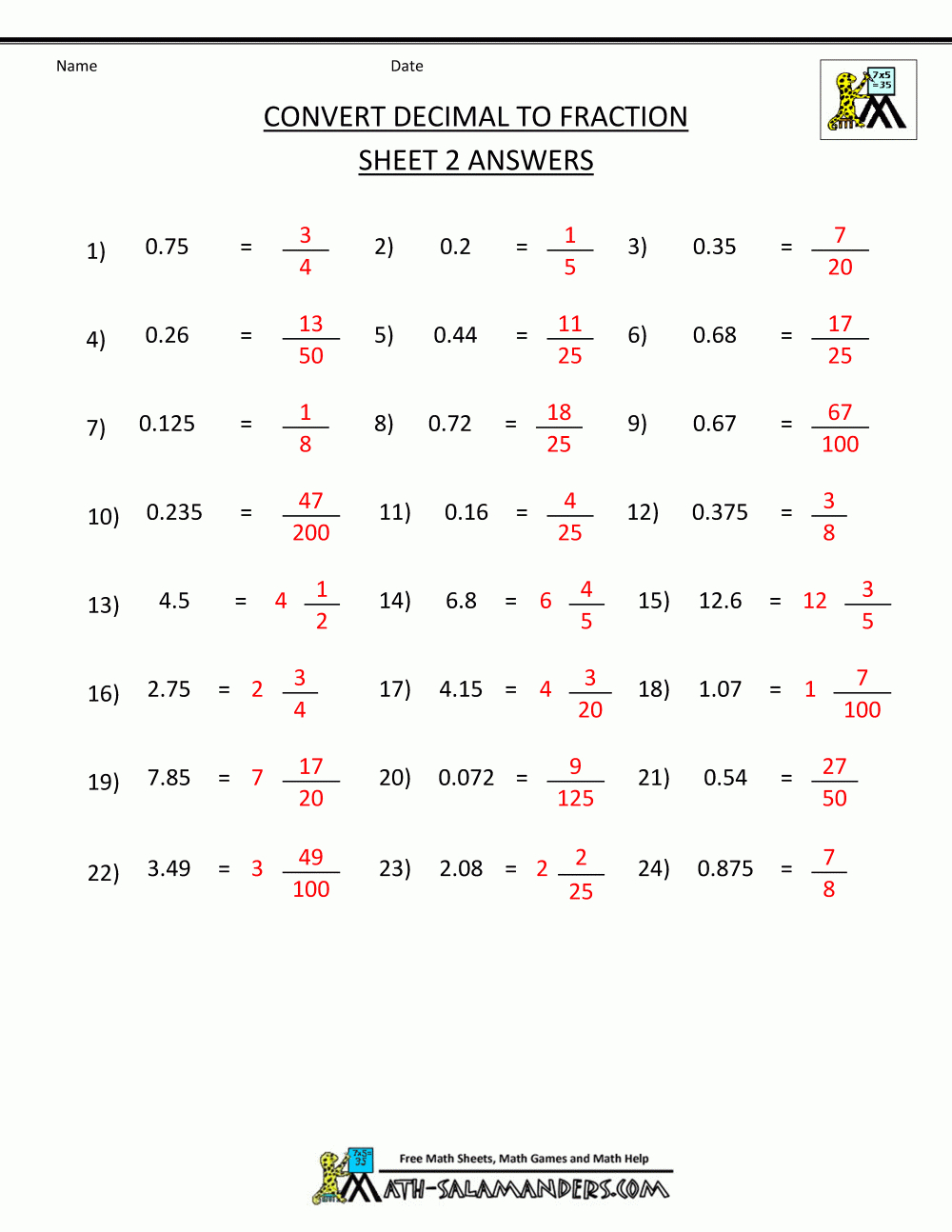
Why learn to convert fractions to decimals?
- Mathematics: For solving equations, understanding graphs, and applying algebra.
- Cooking: Precise measurements often require decimal conversion for accurate proportions.
- Science and Engineering: Measurements and calculations often require decimals for precision.
- Finance: Understanding percentages and interest rates in decimal form is essential.
In Conclusion

Mastering the conversion from fractions to decimals opens up a realm of mathematical applications and problem-solving. With the techniques outlined and the free printable worksheets provided, you're well-equipped to tackle this task. Remember to practice, use the provided resources, and don't shy away from calculators when needed for efficiency and accuracy. Keep in mind that not all fractions convert neatly, but with the knowledge of repeating decimals and the techniques for handling them, you can approach any conversion with confidence.
How can I convert fractions to decimals without using a calculator?

+
Long division is the traditional method. Set up the fraction as a division problem where the numerator is the dividend and the denominator is the divisor. Continue the division, appending zeros to the right of the dividend as needed to achieve the desired decimal accuracy or until the division terminates or repeats.
What does a repeating decimal represent mathematically?

+
A repeating decimal represents a fraction where the division does not terminate. It signifies that the fraction can’t be expressed exactly as a decimal in a finite number of digits. These are commonly encountered in rational numbers like 1⁄3 or 1⁄7.
Are all fractions capable of being converted into exact decimal forms?

+
No. Some fractions, particularly those with denominators not factors of 10, result in infinite, non-repeating decimals. These fractions are linked to irrational numbers like π or the square root of 2.