5 Steps to Master Linear Inequalities Worksheet
Mathematics often presents us with a myriad of challenges, from algebra to geometry, and one of the common areas that can puzzle learners is linear inequalities. Whether you're a high school student looking to solidify your understanding or an adult returning to education, linear inequalities provide a foundation for logical reasoning and problem-solving skills. Here are five steps to master linear inequalities through worksheet practice.
Step 1: Understand the Basics

The first step in mastering linear inequalities is to get a firm grasp of the fundamentals:
- Linear Inequality Definition: A linear inequality compares two expressions using inequality signs (<, ≤, >, ≥).
- Graphical Representation: Linear inequalities can be graphed on a coordinate plane.
- Types of Inequalities: Understand the difference between strict inequalities (x < y) and non-strict inequalities (x ≤ y).

📝 Note: Understanding when to use an open or closed circle on number lines can help clarify the solution sets for linear inequalities.
Step 2: Solve Single-Variable Inequalities
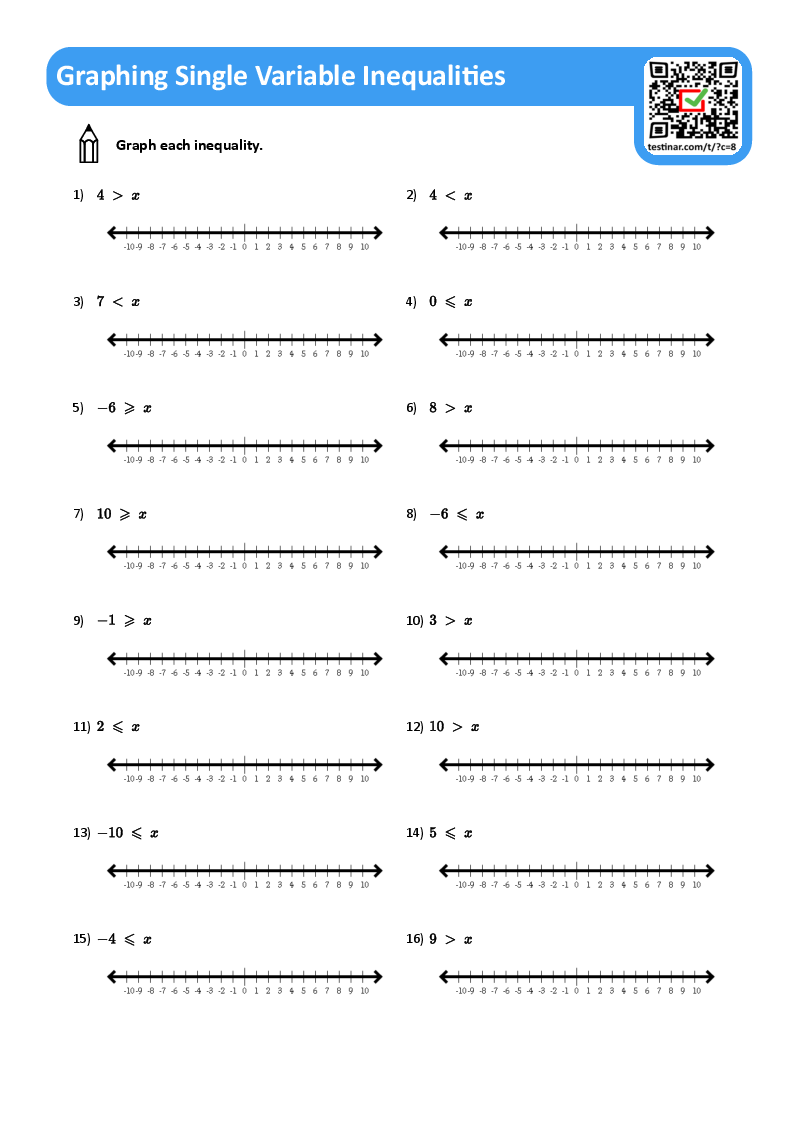
Once you understand the basics, move on to solving inequalities with one variable:
- Isolate the variable on one side of the inequality.
- Remember to reverse the inequality sign when multiplying or dividing by a negative number.
- When solving, you can add or subtract the same value to both sides without changing the inequality.
- Recognize that inequalities have an infinite number of solutions, often represented on a number line.
Step 3: Work on Multi-Step Inequalities

Multi-step inequalities involve more complex operations:
- Distribution: Use the distributive property if necessary.
- Variable Combinations: Combine like terms before solving for the variable.
- Order of Operations: Follow the order of operations when simplifying inequalities.
📝 Note: Practice verifying solutions by substituting them back into the original inequality to ensure accuracy.
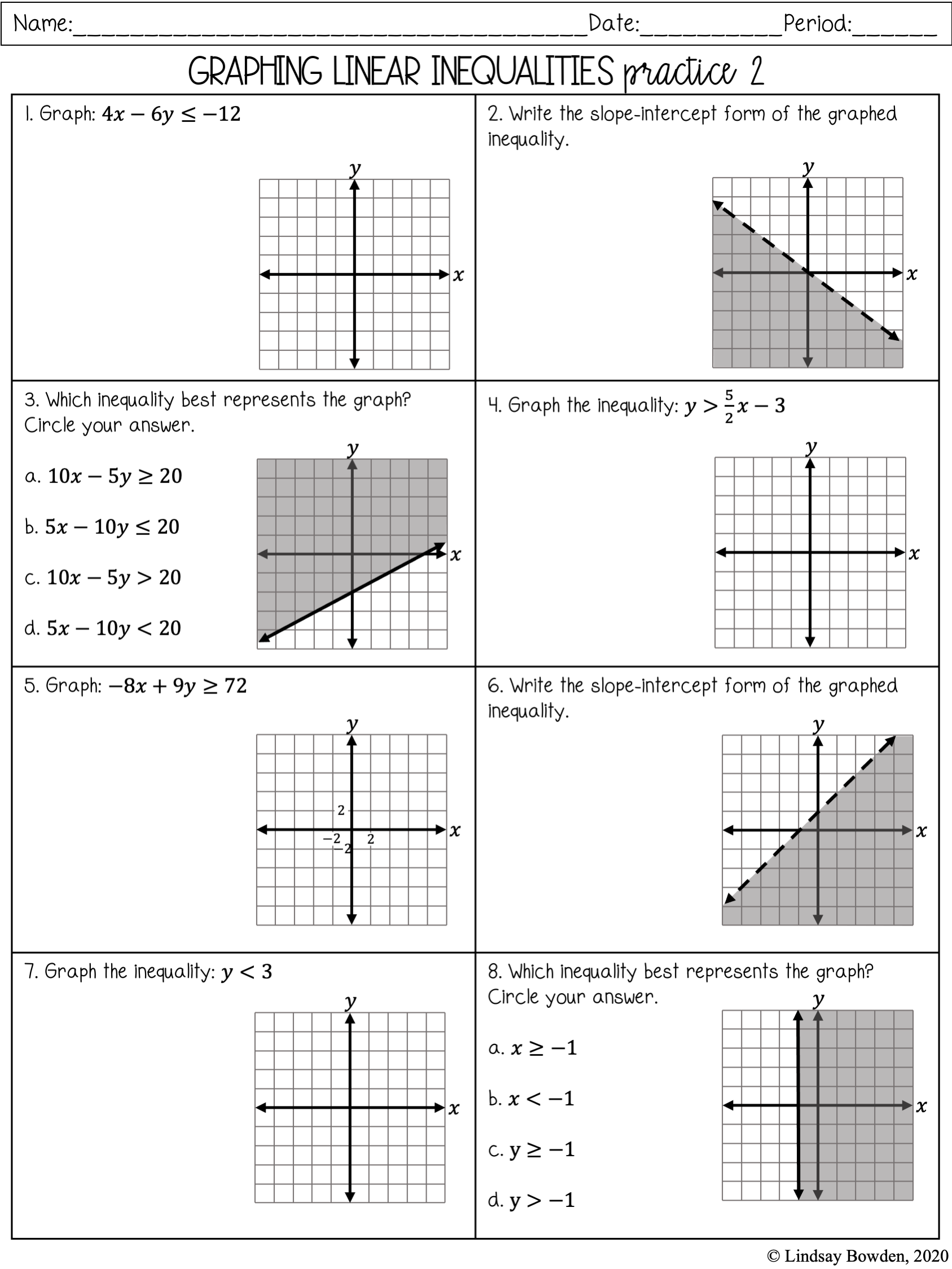
Step 4: Graph and Interpret Two-Variable Inequalities

Linear inequalities can also be expressed in two variables, which requires:
- Graphing: Plot the inequality line and shade the appropriate region on the coordinate plane.
- Understanding Boundary Lines: Solid lines indicate inclusive inequalities (≤, ≥), while dashed lines show exclusive inequalities (<, >).
- Testing Points: Use test points to determine which side of the line to shade.
| Graphical Representation | Description |
|---|---|
| Solid Line | The boundary line is part of the solution set (≤, ≥). |
| Dashed Line | The boundary line is not part of the solution set (<, >). |
| Shading | Represents the region that satisfies the inequality. |

Step 5: Tackle Systems of Inequalities

Systems of inequalities involve solving and graphing multiple inequalities simultaneously:
- Graph each inequality on the same coordinate plane.
- The solution region is where all the shaded areas overlap.
- Identify vertices (if any) where the boundary lines intersect.
- Check the solution region with a test point.
📝 Note: Each additional inequality can significantly reduce the solution set, making this step particularly complex.
Understanding and mastering linear inequalities not only reinforces algebra but also develops critical thinking and analytical skills. Through practice with worksheets, students can transition from simple to complex inequalities, honing their ability to interpret and solve mathematical problems. This journey through linear inequalities involves much more than memorizing steps; it's about understanding the reasoning behind each operation, which can be applied in various real-world scenarios, from business models to game development and beyond.
Why are linear inequalities important?

+
Linear inequalities provide a mathematical framework for understanding constraints, optimizing resources, and modeling real-world problems with variable conditions. They are crucial in fields like economics, engineering, and computer science.
How do you know which side to shade when graphing linear inequalities?

+
Choose a test point not on the boundary line, typically (0, 0), and substitute it into the inequality. If the inequality holds true, shade that side of the line. If not, shade the opposite side.
What are some common mistakes when solving linear inequalities?

+
Common mistakes include forgetting to reverse the inequality sign when dividing or multiplying by a negative number, misinterpreting the boundary line in graphing, and incorrectly simplifying multi-step inequalities.