Arithmetic & Geometric Sequences: Free Worksheet Answers

Understanding arithmetic and geometric sequences is fundamental in mathematics, particularly in algebra. These sequences are not only fascinating patterns but are also used in financial calculations, growth models, and various real-world applications. In this extensive blog post, we'll dive deep into these two types of sequences, explore their characteristics, applications, and provide you with comprehensive worksheets complete with answers to help solidify your understanding.
What Are Sequences?

Before we delve into arithmetic and geometric sequences, let's clarify what a sequence in mathematics means:
- A sequence is an ordered list of numbers where each number is called a term.
- Each term in the sequence has a unique position known as its index.
Sequences are important because they help predict the behavior of numbers in a specific pattern. Now, let's explore the two most common types.
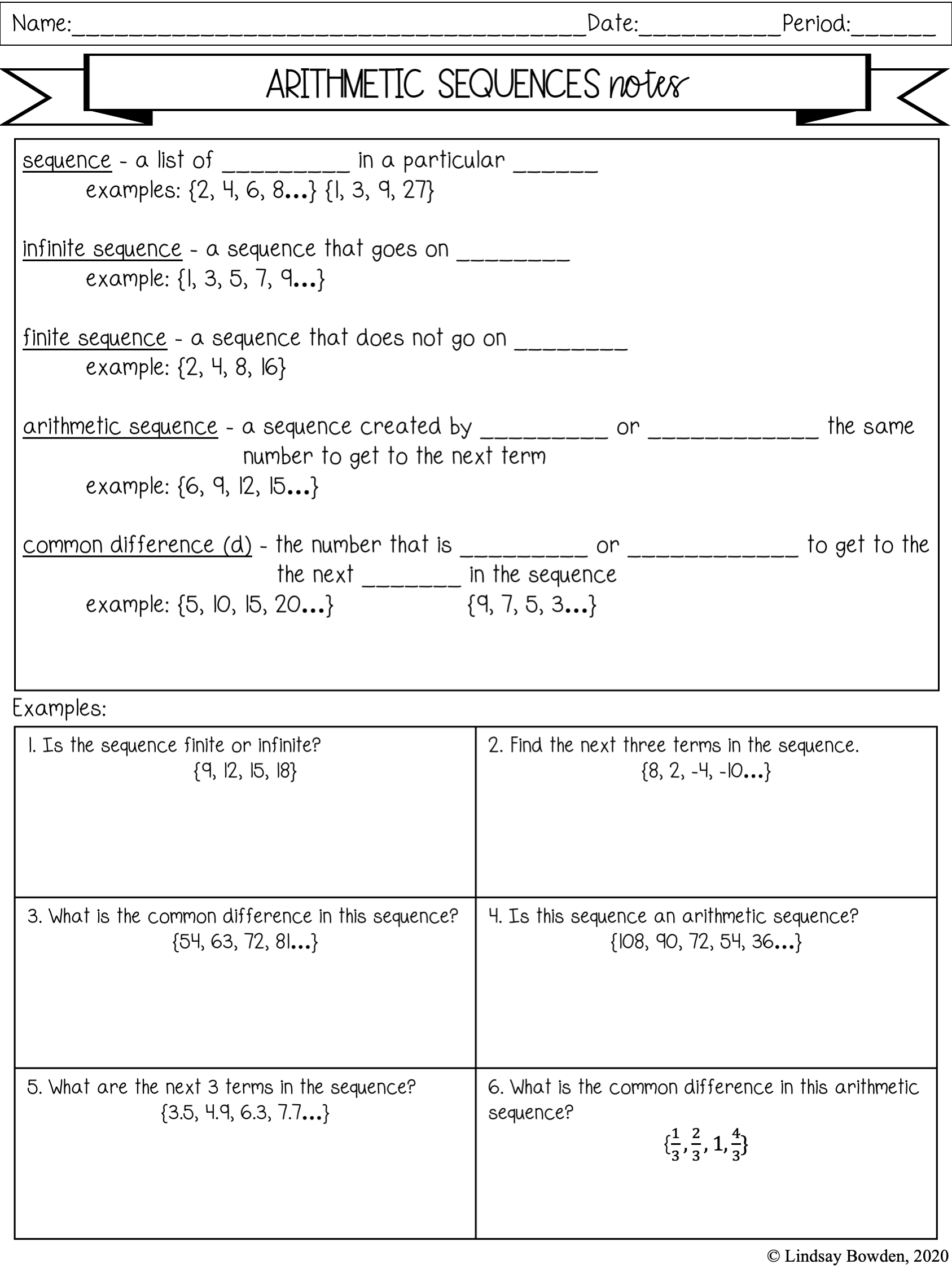
Arithmetic Sequences

An arithmetic sequence is a sequence of numbers where the difference between consecutive terms is constant. This constant difference is referred to as the common difference (d).
- The general form of an arithmetic sequence is: an = a1 + (n - 1)d, where:
- an is the n-th term of the sequence.
- a1 is the first term.
- n is the position of the term.
- d is the common difference.
Examples of Arithmetic Sequences

Here are a few examples to illustrate arithmetic sequences:
- Sequence: 2, 5, 8, 11, 14…
- First term (a1) = 2, common difference (d) = 3
- Sequence: -10, -6, -2, 2, 6…
- First term (a1) = -10, common difference (d) = 4
Calculating Specific Terms

If you know the first term and the common difference, you can find any term in the sequence:
- For the sequence 3, 8, 13, 18… with d = 5, the 7th term is calculated as follows:
- a7 = 3 + (7-1) * 5 = 3 + 30 = 33
⚠️ Note: Don't forget that the common difference (d) can be negative if the sequence is decreasing.
Geometric Sequences
A geometric sequence, on the other hand, is where each term after the first is found by multiplying the previous term by a constant, called the common ratio (r).
- The general form of a geometric sequence is: an = a1 * r(n - 1), where:
- an is the n-th term of the sequence.
- a1 is the first term.
- n is the position of the term.
- r is the common ratio.
Examples of Geometric Sequences

Here are some examples to showcase geometric sequences:
- Sequence: 2, 6, 18, 54…
- First term (a1) = 2, common ratio ® = 3
- Sequence: 162, 54, 18, 6…
- First term (a1) = 162, common ratio ® = 1⁄3
Finding Specific Terms

Using the first term and common ratio, you can find any term in the sequence:
- For the sequence 5, 10, 20, 40… with r = 2, the 6th term is:
- a6 = 5 * 2^(6 - 1) = 5 * 32 = 160
Worksheet: Practicing Arithmetic and Geometric Sequences
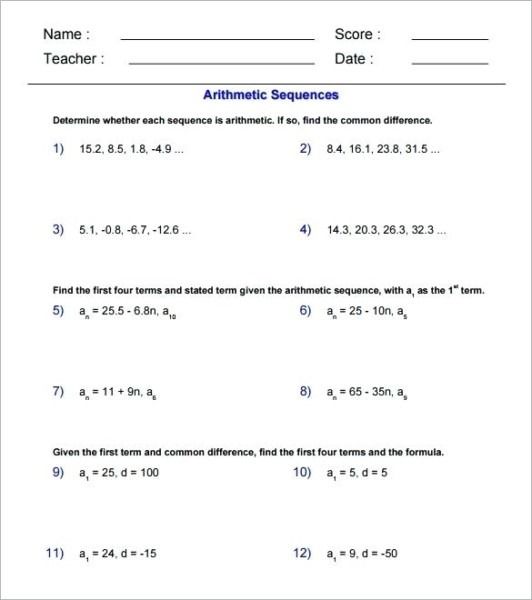
Below is a worksheet designed to help you practice and understand arithmetic and geometric sequences:
| Question | Type | Answer |
|---|---|---|
| 1. Find the 10th term of the sequence 2, 5, 8, 11... | Arithmetic | a10 = 2 + (10 - 1) * 3 = 29 |
| 2. What is the 8th term of the geometric sequence 3, 6, 12, 24... | Geometric | a8 = 3 * 2^(8 - 1) = 384 |
| 3. Determine the common difference of the sequence -4, -2, 0, 2... | Arithmetic | d = 2 - 0 = 2 |
| 4. Find the common ratio of the sequence 1, 1/3, 1/9, 1/27... | Geometric | r = (1/3) / 1 = 1/3 |

📝 Note: When dealing with negative numbers or fractions in sequences, always double-check the calculations to ensure accuracy.
The key to mastering arithmetic and geometric sequences lies in understanding their patterns. Here are some final thoughts:
- Recognize the constant difference in arithmetic sequences.
- Identify the common ratio in geometric sequences.
- Use the general formulas provided to find any term in either type of sequence.
In summary, arithmetic sequences are characterized by their consistent difference between consecutive terms, which makes them useful in modeling linear growth or decline. On the other hand, geometric sequences with their multiplicative property are ideal for modeling exponential growth or decay. These patterns are not just a part of mathematical curiosity but are vital in understanding the world around us through finance, science, engineering, and beyond.
What is the difference between arithmetic and geometric sequences?

+
The difference between these sequences lies in how the terms relate to each other. In an arithmetic sequence, each term increases or decreases by a common difference. In a geometric sequence, each term is found by multiplying the previous term by a common ratio.
How can you tell if a sequence is arithmetic or geometric?

+
To determine if a sequence is arithmetic, check if the difference between consecutive terms is constant. For a geometric sequence, check if the ratio of consecutive terms is constant.
What are real-life applications of these sequences?

+
Arithmetic sequences are used in financial calculations like installment payments or simple interest rates. Geometric sequences model exponential growth or decay, commonly seen in population studies, investments with compound interest, or even the spread of diseases.
Can a sequence be both arithmetic and geometric?

+
No, a sequence cannot be both arithmetic and geometric at the same time, except for very specific cases like a constant sequence where every term is the same, e.g., 3, 3, 3…