5 Key Answers for Linear Inequalities Worksheet

In the realm of algebra, understanding linear inequalities is crucial for tackling more complex mathematical concepts. Linear inequalities are like a set of scales where one side needs to be lighter or heavier than the other, not just equal. Here's a deep dive into key answers for a linear inequalities worksheet, aiming to demystify the process and ensure you grasp the fundamentals with ease.

Understanding Linear Inequalities

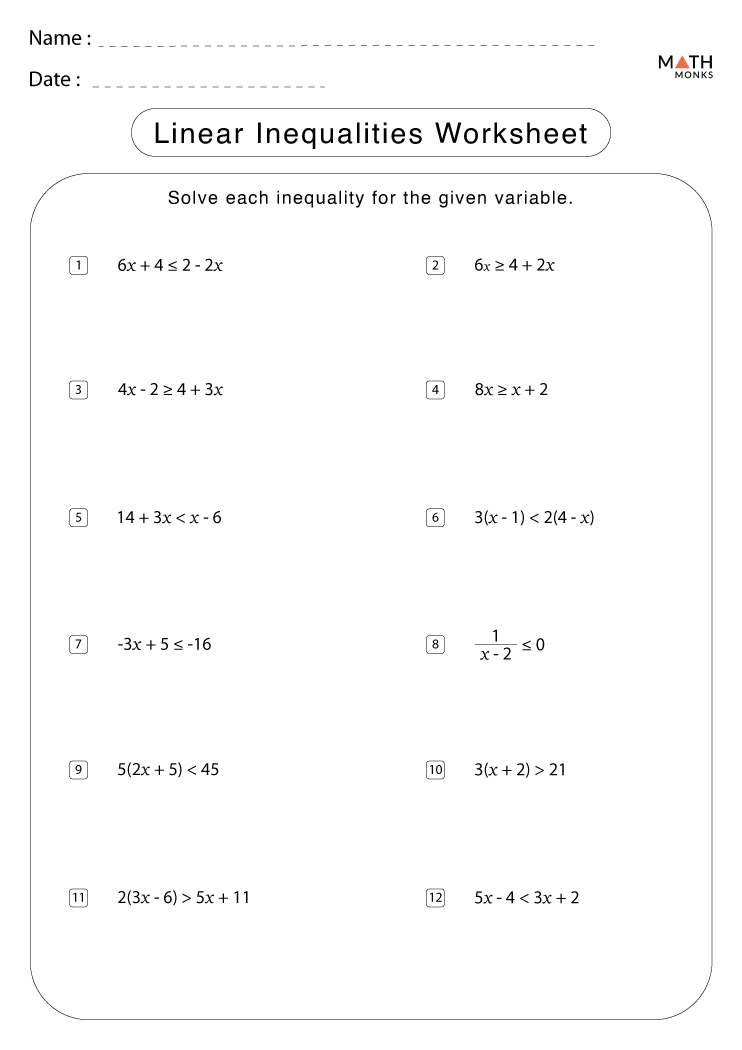
Linear inequalities involve expressions like x + 2 > 7 or y ≤ 4. Unlike equations where you seek an exact balance, inequalities let us explore the boundaries of possible solutions:

• Greater than (>): The solution includes all values to the right of the critical point on a number line.
• Less than (<): The solution includes values to the left of the critical point.
• Greater than or equal to (≥): Includes the critical point itself.
• Less than or equal to (≤): Also includes the critical point.

Solving Linear Inequalities

The goal in solving inequalities is to isolate the variable on one side, similar to solving equations. Here’s the step-by-step process:

1. Isolate the variable: Move terms with the variable to one side and constants to the other. Remember the following rules:
   • Add or subtract the same value to both sides.
   • Multiply or divide both sides by a positive number.
   • If you multiply or divide by a negative number, flip the inequality sign.
2. Check the direction: Ensure the inequality symbol points the right way after any operations.
3. Express the solution: Write the solution in interval notation or on a number line.

📝 Note: Always keep in mind that when you multiply or divide by a negative number, the inequality sign flips to maintain the inequality's meaning.

Key Answers for Linear Inequalities

Let’s delve into common questions you might encounter on a linear inequalities worksheet:

Question 1: Solving a Basic Inequality

Solve the inequality 3x - 5 ≥ 10:

1. Add 5 to both sides: 3x - 5 + 5 ≥ 10 + 5 → 3x ≥ 15
2. Divide both sides by 3: x ≥ 5

The solution is x ≥ 5.

Question 2: Compound Inequalities

Solve -3 < x + 1 < 2:

1. Subtract 1 from all parts: -3 - 1 < x + 1 - 1 < 2 - 1 → -4 < x < 1

The solution is -4 < x < 1.

Question 3: Inequalities with Fractions

Solve frac{2x - 3}{5} + 4 > 1:

1. Subtract 4 from both sides: frac{2x - 3}{5} + 4 - 4 > 1 - 4 → frac{2x - 3}{5} > -3
2. Multiply both sides by 5 to clear the fraction: 2x - 3 > -15
3. Add 3 to both sides: 2x > -12
4. Divide by 2: x > -6

The solution is x > -6.

Question 4: Linear Inequalities with Variables on Both Sides

Solve 5x - 2 < 3x + 8:

1. Subtract 3x from both sides: 5x - 3x - 2 < 3x - 3x + 8 → 2x - 2 < 8
2. Add 2 to both sides: 2x - 2 + 2 < 8 + 2 → 2x < 10
3. Divide by 2: x < 5

The solution is x < 5.

Question 5: Solving Word Problems with Inequalities

Rachel earns 15 per hour working part-time. How many hours must she work to earn at least 225?

• Let h represent the number of hours worked.
• Formulate the inequality: 15h ≥ 225
• Solve for h: h ≥ 15

Rachel needs to work at least 15 hours to earn $225.

Understanding linear inequalities involves learning how to work with these symbols, balance, and interpret solutions:

In conclusion, linear inequalities provide a tool to model real-world problems where equality might not be practical or desired. They offer solutions in ranges or intervals, which is often more useful for real-life applications. By mastering these key answers, you'll be better equipped to handle more advanced topics in algebra and beyond.

What are the symbols used in linear inequalities?

+

The symbols used are > (greater than), < (less than), ≥ (greater than or equal to), and ≤ (less than or equal to).

Why do we sometimes flip the inequality sign?

+

Flipping the inequality sign happens when you multiply or divide both sides of the inequality by a negative number to maintain its validity.

How do you represent the solutions to linear inequalities?

+

Solutions are typically represented on a number line or in interval notation, showing a range of values where the inequality holds true.

What’s the difference between solving a linear equation and a linear inequality?

+

The main difference is that linear equations have exact solutions (points), while inequalities have a range of solutions. Also, with inequalities, you must pay attention to changing the sign when necessary.

Can you have solutions to linear inequalities on a number line?

+

Yes, solutions to linear inequalities can be represented graphically on a number line, indicating where the inequality holds true.