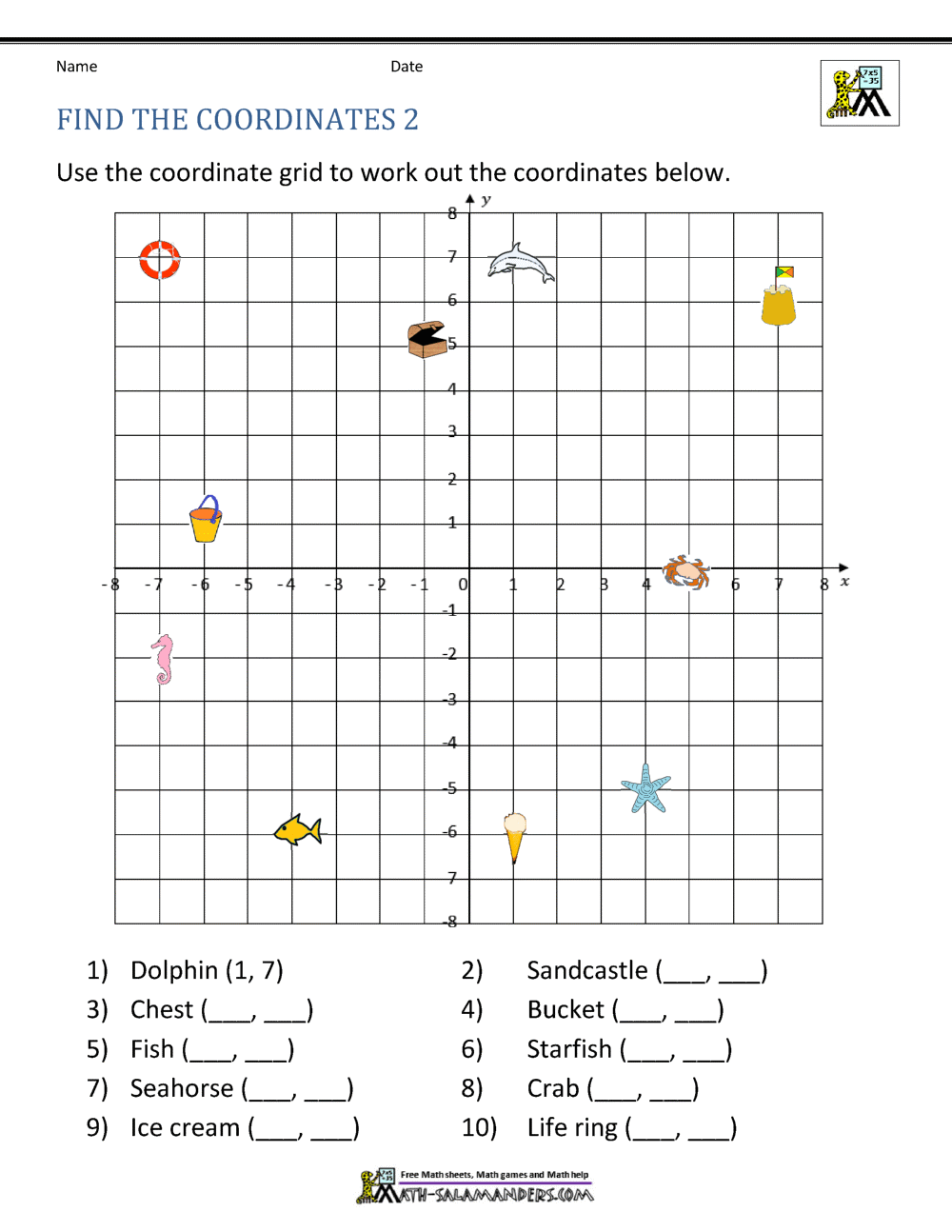
5 Easy Steps to Simplify Linear Expressions

The journey into algebra often starts with mastering the art of simplifying linear expressions. This fundamental skill forms the bedrock for more complex mathematical problems. Simplifying linear expressions is not just a mathematical exercise; it's a way to clarify and organize thought processes in logical problem solving. Here's a step-by-step guide to tackle this task with ease, making algebra a less intimidating field for learners and enthusiasts alike.
Step 1: Understanding the Basics
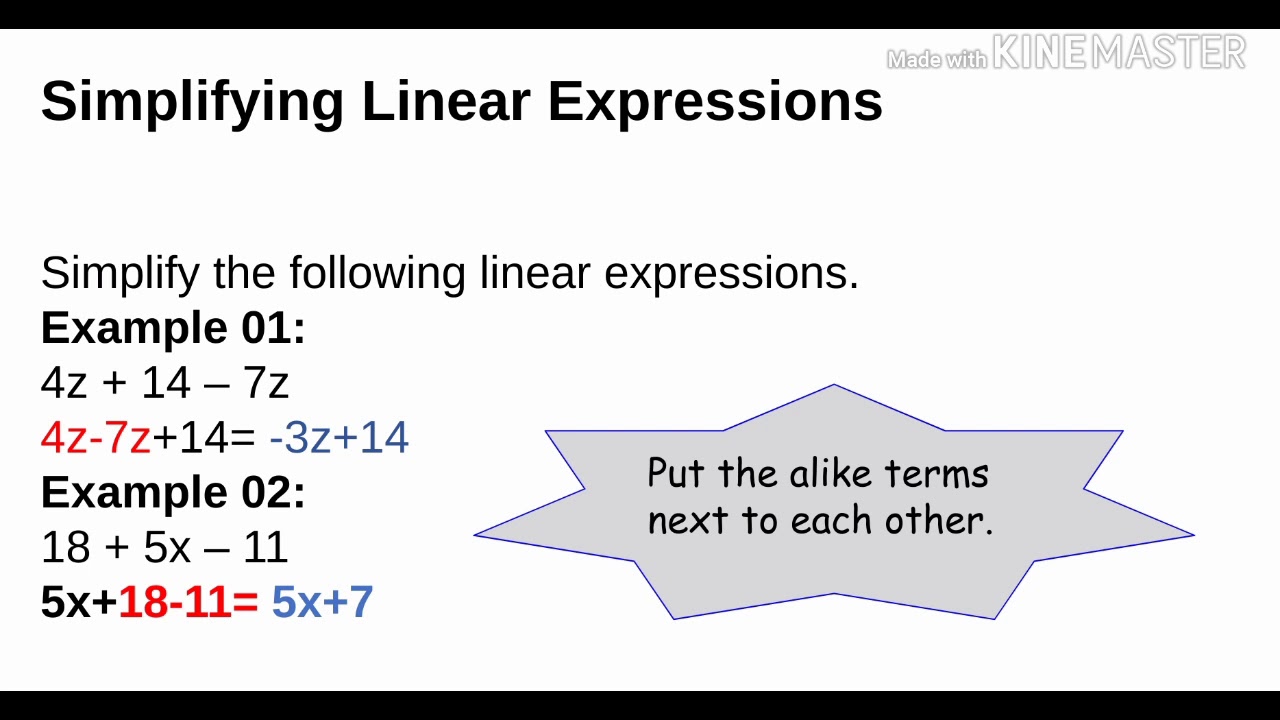
Before diving into the simplification process, it’s crucial to grasp the basic concepts:
- Terms: In algebra, a term is a single number, variable, or a product of numbers and variables. For example, in the expression ( 3x + 2y - 1 ), ( 3x ), ( 2y ), and ( -1 ) are terms.
- Like Terms: These are terms that have the same variables with identical exponents. For instance, ( 3x ) and ( -4x ) are like terms.
- Coefficient: The numerical factor of a term; in ( 3x ), the coefficient is ( 3 ).

Step 2: Combining Like Terms

This step involves:
- Identifying like terms: Look for terms with the same variables and exponents. In ( 4a + 7a + 3 ), ( 4a ) and ( 7a ) are like terms, while ( 3 ) stands alone.
- Adding or Subtracting Coefficients: Once you’ve identified like terms, you can combine them by adding or subtracting their coefficients. Continuing with the example, ( 4a + 7a + 3 ) simplifies to ( (4 + 7)a + 3 ), which equals ( 11a + 3 ).
💡 Note: When there are no like terms, no simplification by combining can be done; the expression remains as is.
Step 3: Dealing with Negative Signs

Here are the key points to remember:
- Distribute the Negative Sign: If there’s a negative sign before a term, distribute it to every part of the term. For instance, ( -(5x - 7) ) becomes ( -5x + 7 ).
- Subtracting a Negative: Keep in mind that subtracting a negative is equivalent to adding a positive. So, ( 8 - (-3) ) becomes ( 8 + 3 ), which equals ( 11 ).
Step 4: Simplifying Brackets and Parentheses

The process involves:
- Distribute the term outside the bracket: Multiply the term outside the parentheses by every term inside. E.g., ( 3(2x - 4) ) simplifies to ( 6x - 12 ).
- Removing Parentheses: Parentheses are removed by performing the distribution process mentioned above.

Step 5: Collecting All Simplified Terms

Once you’ve simplified individual components, collect them:
- Combine the results: Add all the terms together, remembering to combine like terms as necessary. If you’ve worked through ( 4x + (6x - 2) + 3 ), you’ll simplify to ( 4x + 6x - 2 + 3 = 10x + 1 ).
In simplifying linear expressions, the key is to understand the structure of the expression and apply basic algebraic rules systematically. This approach not only simplifies expressions but also clarifies complex mathematical problems by breaking them down into manageable parts. Remember, algebra is about organization and logical sequencing, making problem solving less daunting through a step-by-step method.
As we end this guide, let’s summarize the key steps for simplifying linear expressions:
- Understand the basic components of algebraic expressions.
- Combine like terms efficiently.
- Navigate negative signs with care.
- Simplify brackets and parentheses accurately.
- Collect all simplified terms to get the final result.
What’s the importance of identifying like terms?

+
Identifying like terms is essential for simplifying expressions because it allows you to combine terms, reducing the complexity of the expression. This step makes it easier to solve equations or analyze functions.
How can I ensure I’ve distributed a negative sign correctly?

+
Always distribute the negative sign to every part of the term inside the parentheses. If you have ( -(3x + 5) ), you’ll get ( -3x - 5 ). Remember, a negative sign changes the sign of all terms inside.
Can I apply these steps to non-linear expressions?

+
Yes, you can apply many of these steps to simplify non-linear expressions as well, but the process can be more complex due to additional terms or exponents. The foundational steps remain the same, though.
Embarking on your algebraic journey with a firm grasp of how to simplify linear expressions is an invaluable skill. Whether you’re tackling homework, preparing for an exam, or simply enhancing your problem-solving abilities, these steps will guide you through the process of breaking down complex mathematical structures into understandable and manageable parts.