Mastering Multiplication with Exponents: Free Worksheet Guide

Understanding Multiplication with Exponents

Multiplication with exponents can often seem complex at first glance, but it's an essential concept in algebra, simplifying expressions and solving equations. Exponents, or powers, are a shorthand way to express repeated multiplication of the same number.
Basic Rules of Exponents

Before delving into multiplication with exponents, understanding the basic rules is crucial:
- Product of Powers: a^m \times a^n = a^{m+n}
- Quotient of Powers: \frac{a^m}{a^n} = a^{m-n}
- Power of a Power: (a^m)^n = a^{mn}
- Zero Rule: Any non-zero number raised to the power of zero is 1: a^0 = 1
- Negative Exponents: a^{-n} = \frac{1}{a^n}
These rules are fundamental when simplifying expressions with exponents.
The Power of Multiplication with Exponents

When multiplying two exponential expressions with the same base, you simply add the exponents. Here's how it works:
| Expression | Exponent Rule | Result |
|---|---|---|
| 2^3 \times 2^2 | Product of Powers | 2^{3+2} = 2^5 = 32 |
| x^4 \times x^3 | Product of Powers | x^{4+3} = x^7 |
| 3^2 \times 3^{-1} | Product of Powers | 3^{2+(-1)} = 3^1 = 3 |

⚠️ Note: Ensure that the bases are the same before applying the rules of exponents!
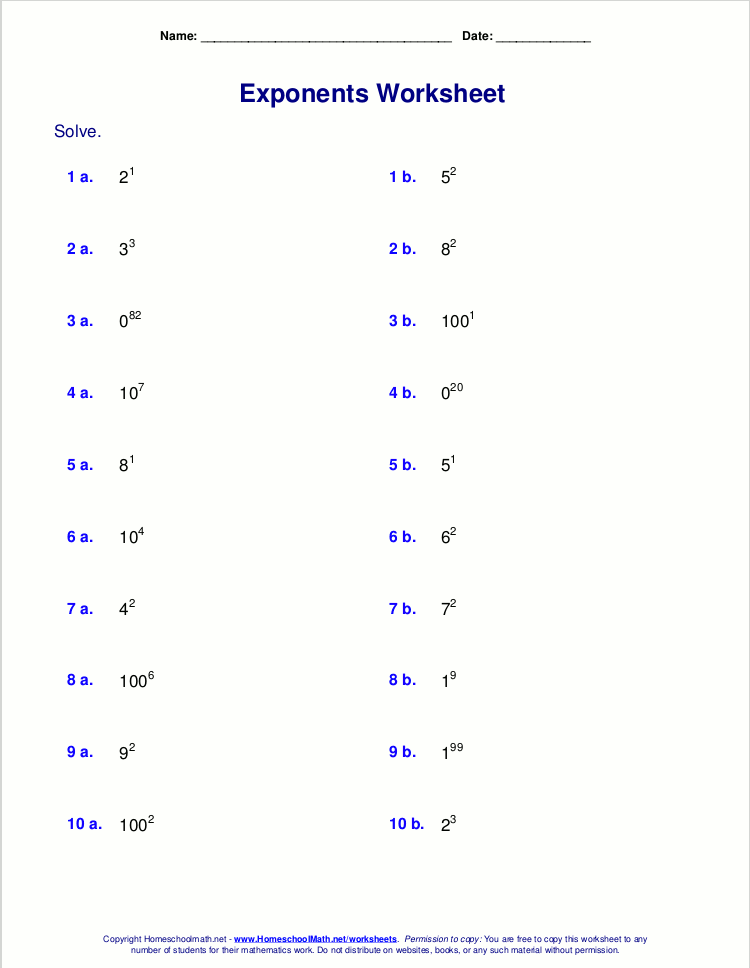
Worksheet Exercises for Practicing Multiplication with Exponents

Here are some problems to help you practice:
- Simplify: 5^2 \times 5^3
- Simplify: x \times x^5
- Simplify: 7^{-2} \times 7^4
- Express 4 \times 4^3 as a power of 4.
- Find the value of 2^0 \times 2^5.
🔍 Note: Remember, when the bases are different, you can't simply add exponents. You need to compute each exponential expression first before multiplying the results.
Applying Exponents in Real-Life Scenarios

Exponents aren't just abstract algebra; they're used in:
- Finance: Compound interest involves exponential growth.
- Physics: For modeling phenomena like radioactive decay or population growth.
- Computer Science: Analyzing algorithms' time complexity often uses exponents.
Common Mistakes and How to Avoid Them

Here are some pitfalls to watch out for:
- Mistaking bases - always ensure the bases are the same before combining exponents.
- Confusing the rules, like multiplying exponents when the bases are the same rather than adding them.
- Ignoring negative exponents or not understanding that they represent reciprocals.
💡 Note: Practicing regularly with worksheets can help avoid these common mistakes.
Putting it All Together

In summary, mastering multiplication with exponents revolves around understanding and correctly applying the basic rules. Use these rules to simplify expressions and solve problems efficiently. Remember, consistent practice with worksheets can lead to mastery, ensuring you can tackle more complex algebraic challenges with confidence.
What is the Product of Powers rule?
+
The Product of Powers rule states that when multiplying two or more exponential expressions with the same base, you simply add their exponents. For example, (a^m \times a^n = a^{m+n}).
How do negative exponents work in multiplication?

+
Negative exponents represent the reciprocal of the base raised to the positive exponent. When multiplying, you still add the exponents, resulting in: (a^{-m} \times a^n = a^{-m+n}), which can be simplified using rules of negative exponents.
Why is it important to understand exponents in math?

+
Understanding exponents is crucial for simplifying expressions, solving equations, and modeling real-life phenomena in fields like finance, physics, and computer science. Exponents allow for concise mathematical expression and help in grasping concepts like exponential growth and decay.
Can I multiply exponents with different bases?

+
No, you cannot simply multiply exponents when the bases are different. You must compute each exponential expression to get the numerical values and then multiply those results.