Limiting Reactant and Percent Yield Worksheet Solutions
Limiting reactants, also known as limiting reagents, are substances in a chemical reaction that dictate how much of the product will be formed. Understanding how to identify the limiting reactant and calculate the theoretical yield, as well as the percent yield, is essential in various fields, particularly chemistry and chemical engineering. This blog post delves into how to solve problems related to these concepts through practical examples, providing you with a clear understanding.
Understanding Limiting Reactants

In any chemical reaction, reactants are combined to form products. However, if one reactant is used up before the others, it becomes the limiting reactant, thereby controlling the amount of product formed. Here's how to determine the limiting reactant:
- Calculate the moles of each reactant present.
- Using the balanced chemical equation, determine how many moles of product each reactant would produce if it were the limiting one.
- The reactant that produces the least amount of product is the limiting reactant.
Worksheet Problem: Identifying the Limiting Reactant
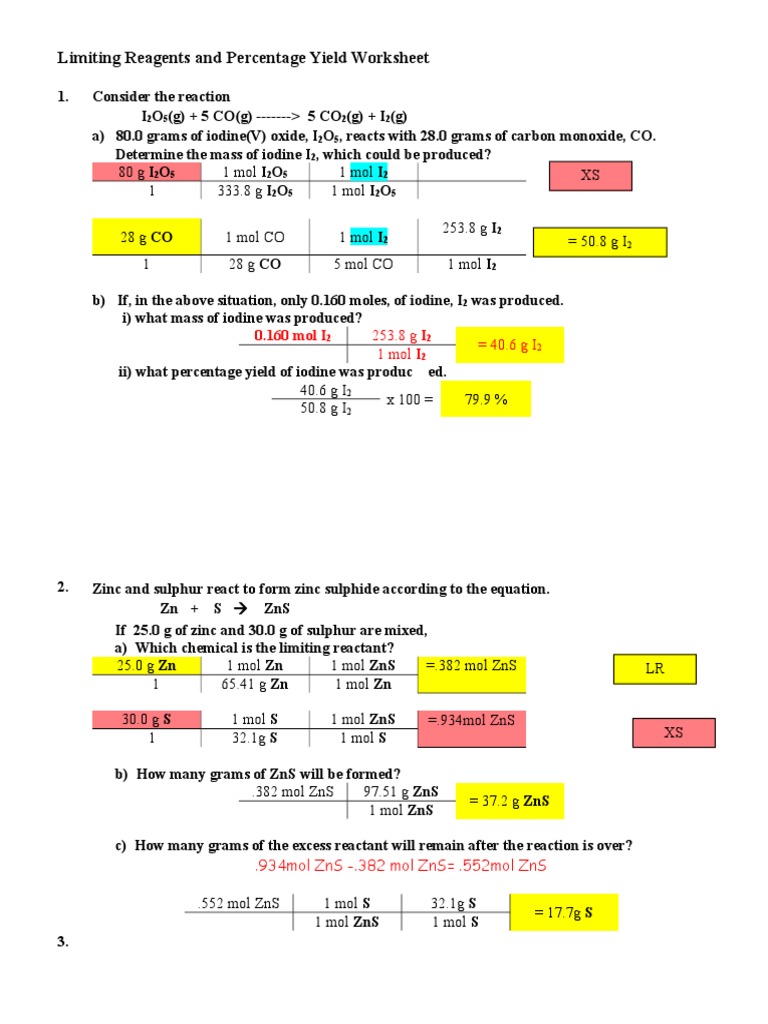
Consider the reaction:
N2 + 3 H2 → 2 NH3
If you have 1.00 mol of N2 and 3.50 mol of H2, which is the limiting reactant?
Solution:

| Reactant | Moles Given | Stoichiometric Ratio | Product Possible |
|---|---|---|---|
| N2 | 1.00 mol | 1 mol N2 : 2 mol NH3 | 2.00 mol NH3 |
| H2 | 3.50 mol | 3 mol H2 : 2 mol NH3 | 2.33 mol NH3 |

Since N2 can only produce 2.00 moles of NH3, it is the limiting reactant.
💡 Note: Remember to account for the stoichiometry of the reaction when determining moles of product.
Calculating Percent Yield

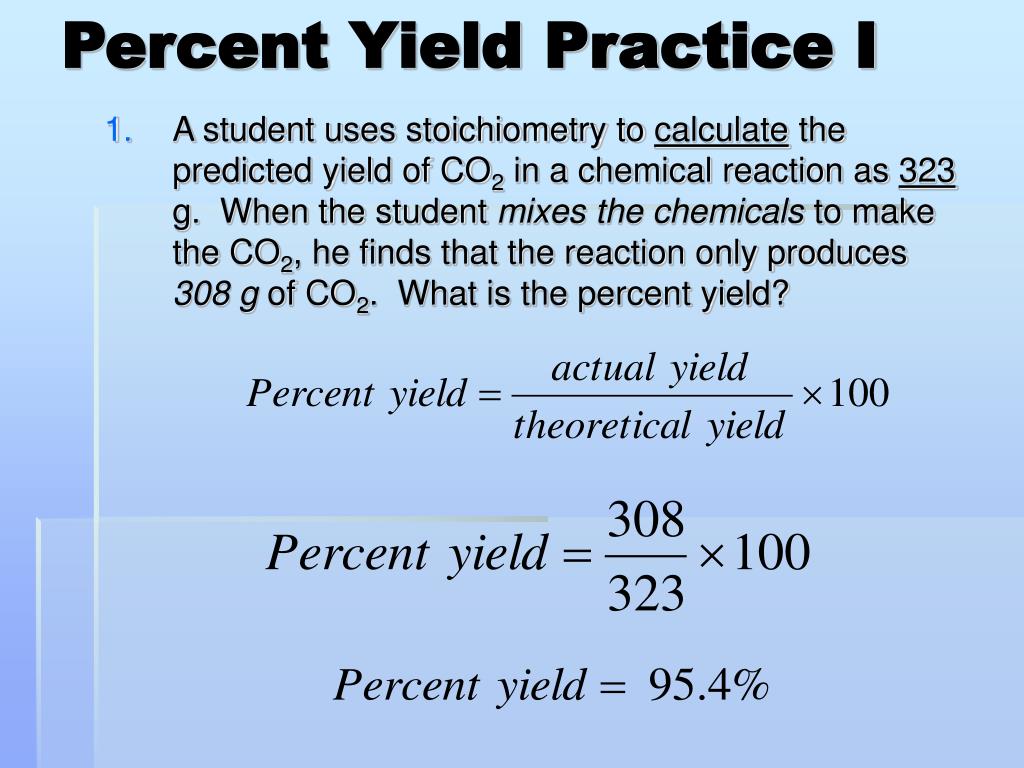
Percent yield relates the actual yield (what you get from an experiment) to the theoretical yield (what you calculate you should get). Here’s the formula:
Percent Yield = (Actual Yield / Theoretical Yield) × 100%
Let's use the previous reaction to illustrate:
- Theoretical Yield: 2.00 mol NH3 (from our limiting reactant calculation)
- Actual Yield: Suppose we collected 1.50 mol NH3
Solution:

Percent Yield = (1.50 mol / 2.00 mol) × 100% = 75%
This calculation helps us understand how efficient our reaction was in terms of turning reactants into products.
💡 Note: Efficiency in chemical reactions can be influenced by factors such as side reactions, impurities, or incomplete reactions.
Additional Examples

Example 1: Limiting Reactant Calculation

Consider the reaction:
FeS + 2 HCl → FeCl2 + H2S
With 2.00 mol FeS and 6.00 mol HCl, what is the limiting reactant?
- FeS can produce (2.00 / 1) = 2.00 mol FeCl2.
- HCl can produce (6.00 / 2) = 3.00 mol FeCl2.
FeS is the limiting reactant here as it produces the smaller amount of product.
Example 2: Percent Yield Calculation

For the reaction:
2 CO + O2 → 2 CO2
If we start with 5.00 mol CO and 2.00 mol O2, what is the percent yield if we collect 2.50 mol CO2?
- Theoretical Yield: Since O2 is the limiting reactant, producing 2 × 2.00 = 4.00 mol CO2.
- Percent Yield = (2.50 / 4.00) × 100% = 62.5%
Recap and Key Points

By now, you should be able to identify the limiting reactant in a reaction, calculate the theoretical yield based on stoichiometry, and compute the percent yield to assess the efficiency of your chemical reaction. Here are some key takeaways:
- The limiting reactant controls the amount of product formed.
- Theoretical yield is calculated using the balanced chemical equation and the moles of the limiting reactant.
- Percent yield is the ratio of actual to theoretical yield, expressed as a percentage.
With these skills, you're better equipped to predict outcomes in chemical synthesis and understand real-world applications of chemical reactions.
What if there are no limiting reactants?

+
If the reactants are in the correct stoichiometric ratio as per the balanced equation, all reactants are consumed equally, and there is no single limiting reactant.
How do impurities affect percent yield?

+
Impurities can reduce the actual yield by reacting with either the reactants or the products, or by simply not contributing to the desired reaction, thus lowering the percent yield.
Why is it important to calculate percent yield?

+
Calculating percent yield helps chemists assess the efficiency of a reaction, understand where losses occur, and potentially improve experimental conditions to maximize product yield.