5 Quick Tips to Master Cylinder and Cone Volumes
Understanding how to calculate the volumes of cylinders and cones is an essential skill in various fields, from engineering to home improvement. Whether you're planning to build something or need to determine capacities for liquid or gas containers, mastering these calculations can streamline your projects and enhance your efficiency. Here are five quick tips to help you become adept at calculating the volumes of cylinders and cones.
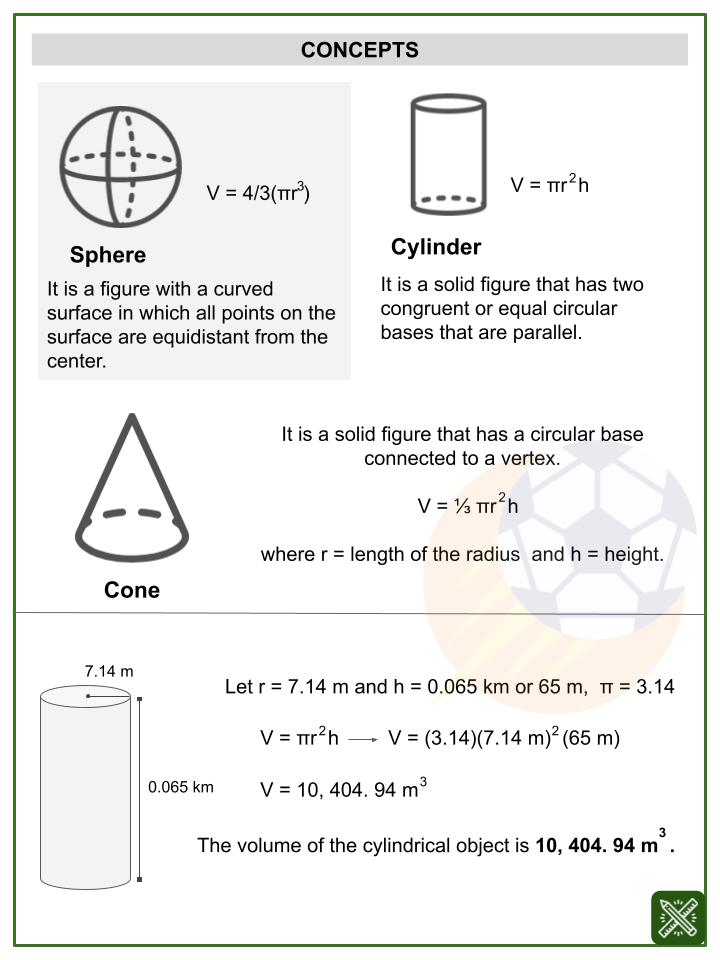
Visualize the Shapes

Before diving into the equations, a good understanding of the shapes helps:
- Cylinder: Imagine a can of soup or a drum. It has two parallel, congruent circular bases and a height connecting them.
- Cone: Picture an ice cream cone. It has a circular base tapering to a point. The key difference from a cylinder is its apex.
Visualizing these shapes can make it easier to remember the formulas and understand why they work.
Know the Formulas

Here are the essential formulas:
| Shape | Formula |
|---|---|
| Cylinder | V = πr²h |
| Cone | V = (1⁄3)πr²h |

Where:
- V is the volume
- r is the radius of the base circle
- h is the height of the shape
Use Proper Units

Consistency in units is crucial:
- When measuring, ensure your radius and height are in the same units, such as meters, feet, or inches.
- Be aware that since π is dimensionless, the unit of your volume calculation will be the unit of measurement cubed (e.g., cubic meters or cubic feet).
📝 Note: Forgetting to square or cube your units can lead to significant calculation errors.
Practice With Examples

Applying formulas to real-life examples can solidify your understanding:
- Cylinder Example: A cylindrical tank with a radius of 2 meters and a height of 3 meters. Using the formula, V = π (2)² (3) = 12π ≈ 37.68 m³.
- Cone Example: A conical container with a radius of 4 inches and a height of 10 inches. Using the formula, V = (1⁄3) π (4)² (10) = 53.33π ≈ 167.55 in³.
Utilize Technology

Modern tools can greatly assist:
- Use online calculators or apps to verify your calculations.
- Spreadsheet software like Excel has built-in functions (e.g., =PI()*power(radius,2)*height for cylinders) that can quickly compute these volumes for you.
By mastering these tips, you can quickly and accurately calculate the volumes of cylinders and cones, making your work in various fields more efficient and accurate. This knowledge can lead to better planning, cost estimations, and design decisions, enhancing both your professional capabilities and personal projects.
Why do cylinders have more volume than cones of the same base and height?

+
A cylinder’s volume formula does not account for tapering to a point, unlike the cone where one-third of the volume is lost due to its shape.
Can I calculate the volume without π?

+
While π is essential for exact calculations, for practical estimates, you can approximate π as 3.14 or use 22⁄7 for simplicity.
What should I do if my cone is truncated?

+
The formula for a truncated cone involves calculating the volume of two cones with different heights and then subtracting the smaller from the larger.