7 Geometric Mean Problems Solved for Better Understanding

In the realm of mathematics, the geometric mean is a vital tool used to calculate average growth rates, compare different rates of change, and find the central tendency of data sets with varying scale. Often misunderstood or miscalculated, the geometric mean stands out for its unique approach to representing the 'average' in contexts where multiplication or division is the operational norm. Here, we tackle seven specific geometric mean problems that will not only clarify its computation but also deepen your understanding of its application across various domains.
Understanding the Geometric Mean

Before diving into problems, let's solidify our grasp of the geometric mean. It is defined as:
- The nth root of the product of n numbers.
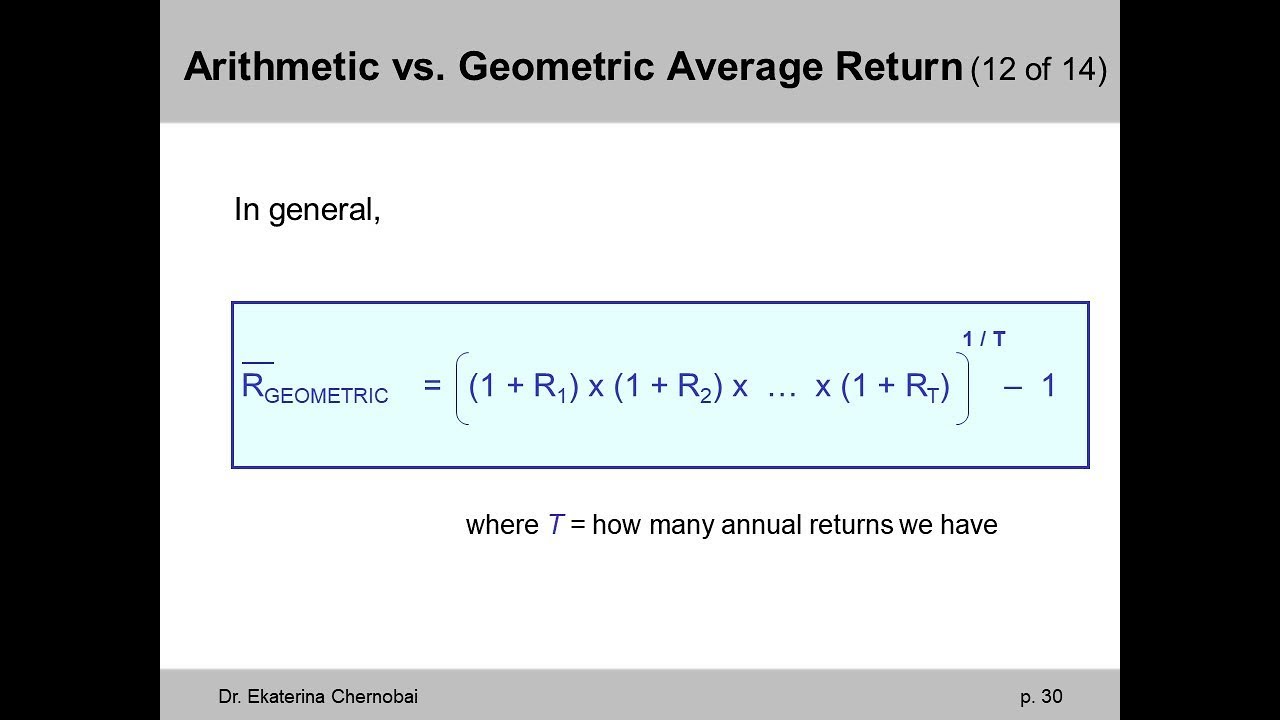
- Mathematically expressed as: GM = √[n](x₁ * x₂ * ... * xₙ)
This definition is particularly useful in fields like finance, where compound interest is at play, or in statistics, when analyzing ratio-level data. Unlike the arithmetic mean, the geometric mean considers the relationship between the numbers by taking their product, which makes it more appropriate for certain types of data analysis.
Problem 1: Simple Geometric Mean Calculation

Let's start with a straightforward example:
Given the numbers 2, 4, and 8, find their geometric mean.
Using the formula:
- GM = √[3](2 * 4 * 8)
- GM = √[3](64) ≈ 4
Important: Notice how the geometric mean is always less than or equal to the arithmetic mean, except when all values are equal.
Problem 2: Comparing Geometric and Arithmetic Means

Now, let's illustrate the difference between geometric and arithmetic means:
Given the numbers 1, 3, 5, and 15, find both means.
| Type | Formula | Result |
|---|---|---|
| Geometric | √[4](1 * 3 * 5 * 15) | ≈ 4.08 |
| Arithmetic | (1 + 3 + 5 + 15) / 4 | 6 |
This comparison helps to emphasize that the geometric mean is not just a different average but provides a different perspective on central tendency.
✏️ Note: The geometric mean is less sensitive to extreme values, making it more suitable for skewed data.
Problem 3: Financial Application

The geometric mean is particularly useful in finance. Suppose you have a stock with annual returns of 10%, -5%, 15%, and 8%. Calculate the average annual return using the geometric mean:
- GM = √[4](1.10 * 0.95 * 1.15 * 1.08) - 1
- GM ≈ 6.8%
Here, the arithmetic mean would misleadingly suggest a higher return due to the positive skewing effect of high returns, but the geometric mean provides a more accurate long-term average return.
Problem 4: Geometric Mean in Population Growth

Consider a population growing at rates of 2% per year, then 4%, and finally 3% over three years. What is the geometric mean growth rate?
- GM = √[3](1.02 * 1.04 * 1.03) - 1
- GM ≈ 3%
This example highlights how the geometric mean can provide a smoother estimation of average growth rates over time, avoiding the overstatement of growth by the arithmetic mean.
Problem 5: Properties of Logarithms

The geometric mean can be conveniently computed using logarithms, which simplifies the calculation of products to sums:
- Log(GM) = (log(x₁) + log(x₂) + ... + log(xₙ)) / n
Using this approach can be particularly efficient for datasets with many values:
- Find the geometric mean of 100, 500, and 200 using logarithms:
- log(100) + log(500) + log(200) = log(2.00 * 5.00 * 2.25)
- log(GM) = (2 + 3 + 2.301) / 3
- GM ≈ 2.48 * 10 = 248
Problem 6: Calculating Growth Rates in Different Periods

Assume an investment starts at $10,000 and grows to $11,000 over the first year, then to $11,500 over the second year, and finally to $12,100 over the third year. Find the geometric mean rate of growth per annum:
- GM = √[3](1.1 * 1.05 * 1.06) - 1
- GM ≈ 3.5%
Here, the arithmetic mean would overestimate the growth rate. The geometric mean, by taking the product of rates, provides a more accurate long-term growth estimate.
Problem 7: Application in Geometric Series

The geometric mean naturally arises when dealing with geometric series. If a, ar, ar², ..., ar^(n-1) is a geometric sequence, the geometric mean is essentially the common ratio 'r':
- Given a geometric series 1, 3, 9, 27, find the common ratio and the geometric mean:
- The common ratio r = 3 / 1 = 3
- GM = √[4](1 * 3 * 9 * 27) ≈ 4.53
This example illustrates that in a geometric sequence, the geometric mean can represent the typical rate of change or growth, which is different from the arithmetic mean's approach.
As we wrap up, understanding the geometric mean through these problems enriches one's mathematical insight, particularly in handling rates of growth, financial calculations, or any scenario where data must be evaluated in terms of multiplicative relationships. Each problem has offered a different lens through which we can appreciate the nuances of this statistical measure. It's evident that the geometric mean provides a valuable alternative to the more commonly understood arithmetic mean, particularly in contexts where growth or ratio-based comparisons are prevalent. Its application transcends mere mathematical exercises, touching upon real-world scenarios from finance to science, where understanding growth rates, compounded returns, or even the behavior of natural phenomena like population growth becomes crucial.
Why use the geometric mean instead of the arithmetic mean?

+
The geometric mean is more appropriate when dealing with rates of change or multiplicative processes, offering a more accurate representation of average growth or the ‘average’ of ratios.
Can the geometric mean ever be larger than the arithmetic mean?

+
No, due to the AM-GM inequality, the geometric mean is always less than or equal to the arithmetic mean, with equality only when all numbers are the same.
How do negative numbers or zero affect the geometric mean?

+
The geometric mean cannot be calculated with negative numbers or zero, as the logarithm of a negative number or zero does not exist in the real number system.
What does the geometric mean tell us about the data set?

+
The geometric mean provides insight into the central tendency of data where scale or rate is important, essentially showing the ‘typical’ multiplicative effect over the dataset.
Is there a practical example where the geometric mean is better than the arithmetic mean?

+
Absolutely, for instance, in finance, when calculating the average return of an investment over multiple periods, the geometric mean gives a more accurate long-term growth rate compared to the arithmetic mean which can be skewed by extreme values.