5 Ways to Master Literal Equations in Algebra

Algebra forms the backbone of advanced mathematics, and a core component of algebra is the ability to solve literal equations. Literal equations, where you solve for one variable in terms of others, are essential not only in academic settings but also in real-world applications like finance, engineering, and science. This blog post delves into five detailed methods that can help you become adept at tackling these equations. Here’s how you can elevate your skills:
1. Understand the Structure

The first step in mastering literal equations is understanding their structure. Every equation has variables and constants, with terms linked by operations like addition, subtraction, multiplication, and division. Let's consider a simple example:
Here, 'x' is what we aim to solve for, while 'a', 'b', and 'c' are constants or variables we are given.
💡 Note: Recognizing the form of an equation (whether it's linear, quadratic, or something else) helps in choosing the right strategy to solve for the variable.
2. Rearrangement Techniques

Rearranging the equation to isolate the desired variable is key:
- Inverse Operations: Apply the inverse of each operation involved to both sides of the equation to eliminate unwanted terms.
- Distributive Property: Use this property to multiply through parentheses, which can simplify complex expressions.
For instance, to solve ax + b = c for 'x':
- Subtract 'b' from both sides to cancel out the constant term.
- Divide both sides by 'a' to get 'x' on its own.
📝 Note: Always ensure that operations performed to one side of the equation are done to the other to maintain equality.
3. Substitution Method

This method involves substituting expressions for one variable in terms of another, which can simplify the equation:
| Step | Action |
|---|---|
| 1 | Solve one equation for one variable. |
| 2 | Substitute this expression into another equation. |
| 3 | Solve the new equation for the remaining variable. |
| 4 | Substitute back to find the value of the first variable. |

🚀 Note: This method is particularly useful in systems of equations where solving by substitution reduces complexity.
4. Quadratic Approach

If you encounter quadratic equations in your literal form, knowing how to manipulate them is crucial:
- Standard Form: Move all terms to one side to get the equation in standard form, e.g., ax^2 + bx + c = 0.
- Factoring: Factor the quadratic to find solutions, or use the quadratic formula for more complex cases.
- Completing the Square: Transform the equation into a perfect square trinomial, solving via roots.
5. Mastering Common Literal Equations
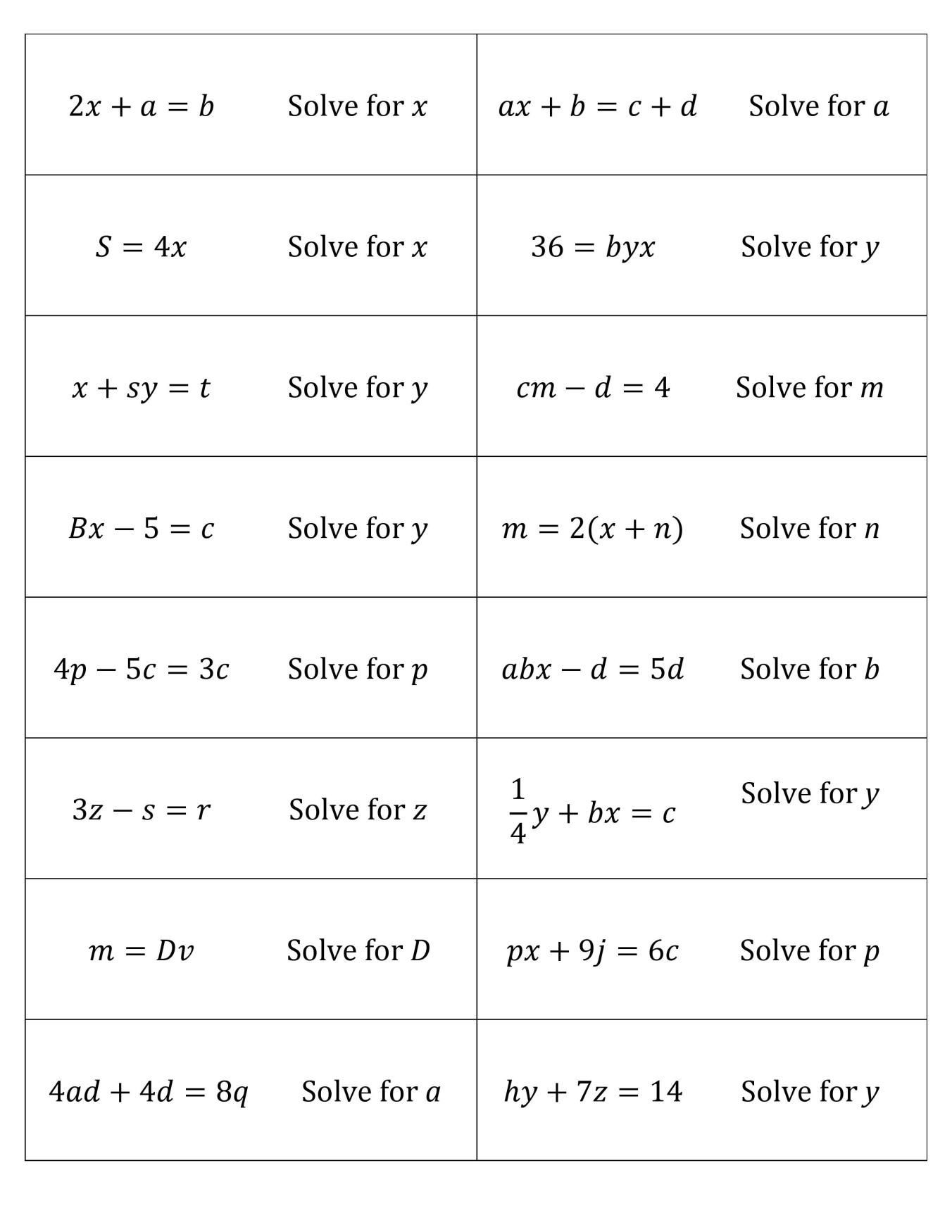
To enhance your proficiency, familiarize yourself with some commonly used literal equations:
- Distance Formula: d = rt
- Area of a Circle: A = \pi r^2
- Ohm’s Law: V = IR
Learning how to rearrange these to solve for different variables can increase your speed and accuracy:
- For d = rt, solve for time t by dividing both sides by r: t = \frac{d}{r}
In conclusion, mastering literal equations involves not only understanding their structure but also using various techniques like rearranging, substitution, and tackling quadratic forms. Through practice and application, these equations become less daunting, and you'll develop the ability to solve them quickly and efficiently. The key is to practice different scenarios and real-life problems to reinforce your understanding, which will significantly enhance your mathematical problem-solving skills.
What is a literal equation?

+
A literal equation is an equation that contains more than one variable, where you solve for one of these variables in terms of the others.
Why are literal equations important?

+
They are critical in understanding and applying mathematical concepts across various disciplines, from physics to finance, helping to analyze relationships between variables.
Can literal equations be solved without variables?

+
Literal equations inherently involve variables. However, you can solve for one variable given the values of others, effectively reducing it to a standard equation.