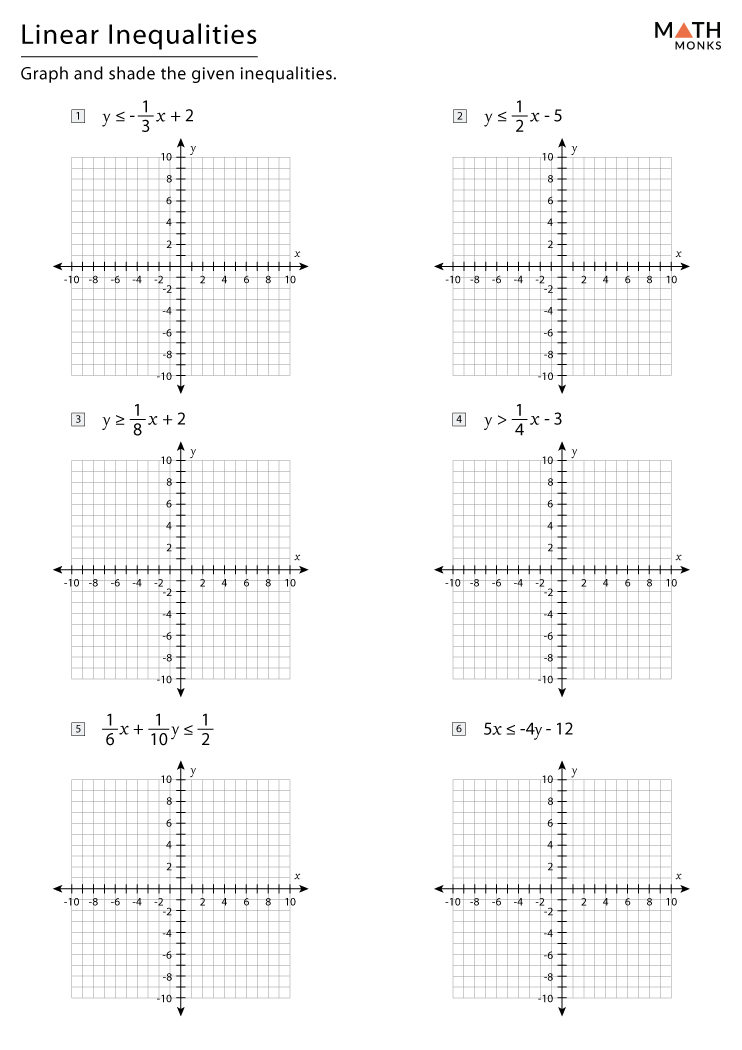
Master Division: Divide by Decimals Worksheet Guide

Teaching students the art of division, especially division by decimals, is both an essential part of their mathematical journey and a stepping stone to more complex arithmetic. Mastery of this skill not only boosts confidence in numbers but also lays a foundation for algebra and beyond. In this comprehensive guide, we will delve into various strategies, practical examples, and exercises to help students and educators alike in mastering division by decimals.
Understanding Division by Decimals

Before we dive into the exercises, it's crucial to understand what division by decimals entails. When dividing a number by a decimal, we essentially alter our perception of the division problem to turn it into a more straightforward integer division. Here are some key points:
- Dividing by a decimal is similar to dividing by a whole number, but with an additional step to adjust the numbers.
- To simplify the process, we can move the decimal point in the divisor (the number we're dividing by) to the right, turning it into a whole number.
- We must then move the decimal point in the dividend (the number being divided) the same number of places to the right, to maintain the quotient's value.
Examples to Grasp the Concept

Example 1: Dividing by a Decimal Smaller than 1

Let's divide 15 by 0.3:
- First, move the decimal point in 0.3 two places to the right to make it 3. Now, we're essentially dividing by 3.
- Move the decimal point in 15 two places to the right as well, turning it into 1500. Now, our division is 1500 ÷ 3.
- 1500 ÷ 3 = 500.
Example 2: Dividing by a Decimal Greater than 1

Let's divide 22.4 by 1.6:
- Move the decimal point in 1.6 one place to the right, turning it into 16.
- Move the decimal point in 22.4 one place to the right, turning it into 224. Now, our division is 224 ÷ 16.
- 224 ÷ 16 = 14.
✅ Note: Ensure to align the decimal points when converting both the dividend and divisor to integers. This step is critical to maintaining the accuracy of the final quotient.
Division by Decimals Worksheet

To solidify your understanding, here's a simple worksheet:
| Dividend | Divisor | Quotient |
|---|---|---|
| 30 | 0.5 | |
| 8.4 | 1.4 | |
| 22.5 | 0.5 | |
| 7 | 2.8 |
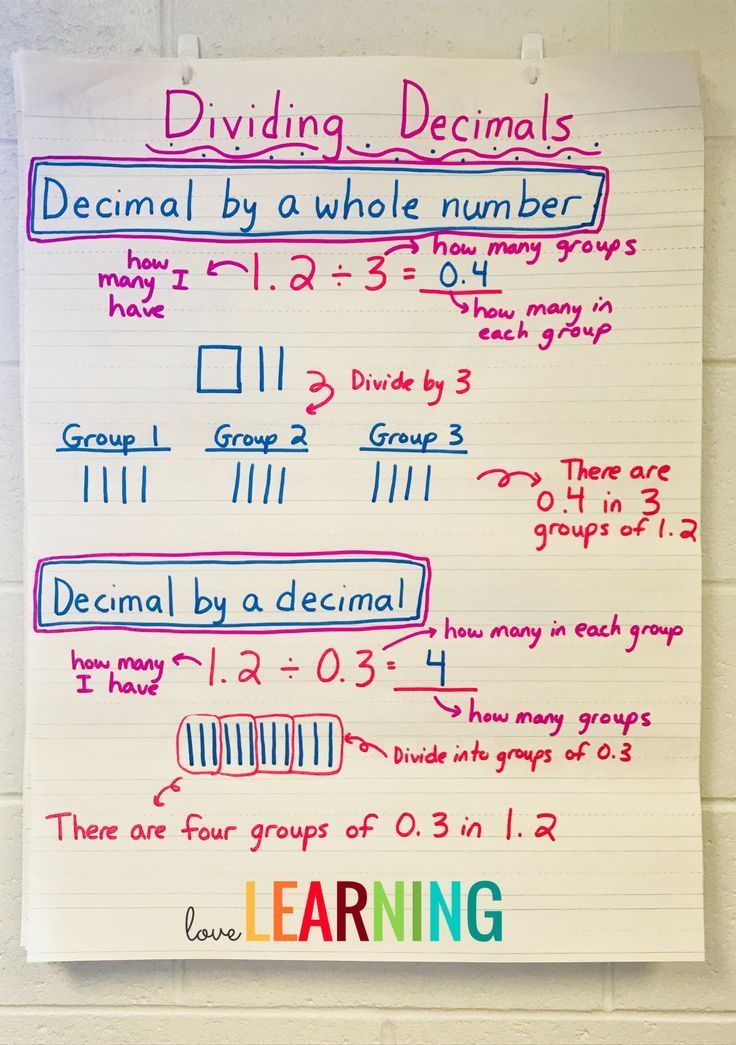
Tips for Teaching and Learning

When introducing students to division by decimals:
- Use Real-World Examples: Connect the problem to real-life scenarios, like dividing a bill among friends, to make the abstract concept more tangible.
- Practice Estimation: Before calculating exact quotients, let students estimate the result. This can help in understanding the magnitude and scale.
- Visual Aids: Utilize number lines, grids, or even physical manipulatives to visually represent the division process.
- Step-by-Step: Break down the division process into steps to avoid overwhelming the learner. Show how shifting the decimal points makes the division simpler.
🔍 Note: Always encourage students to check their work. They can do this by multiplying the quotient by the divisor to see if it approximates the original dividend.
Common Challenges and Solutions

Some common pitfalls when dividing by decimals include:
- Not aligning the decimal points when converting to integers.
- Failing to shift the decimal point in the dividend the same number of places as in the divisor.
- Misplacing the decimal point in the final answer.
Here are some solutions:
- Stress precision: Teach students to always count the number of decimal places moved to keep their work consistent.
- Practice with zeros: Often, adding zeros to the dividend (right side) can simplify the calculation and clarify misconceptions.
- Double-check results: Encourage students to multiply the result back to check if it matches the original problem.
Division by decimals may seem daunting, but with the right approach, it becomes a valuable tool in the student's mathematical toolkit. It's not just about getting the right answer; it's about understanding the process and developing a logical approach to problem-solving. As educators, our goal is to foster this understanding, encouraging both proficiency and conceptual clarity.
Why is understanding decimal division important?

+
Understanding decimal division is crucial because it prepares students for more complex mathematical operations, like ratios, proportions, and algebraic equations. It also aids in real-world scenarios where exact measurements and divisions are necessary.
How can I teach division by decimals effectively?

+
Use visual aids, real-life examples, and step-by-step processes. Start with simpler divisions and gradually increase the complexity. Encourage students to estimate before calculating and always check their work through multiplication.
What are common mistakes students make with decimal division?

+
Common mistakes include not aligning decimal points when converting to integers, forgetting to move the decimal point in the dividend, and misplacing the decimal point in the final quotient.