5 Trig Word Problems Solved: Answers Revealed

In the world of mathematics, trigonometry stands as a bridge between algebra and geometry, offering insights into the angles, distances, and ratios that define our physical world. Whether you're navigating the seas, designing buildings, or just solving a particularly tricky homework problem, trigonometry comes into play. Today, we'll delve into five common trig word problems, providing not just the answers but also a clear, step-by-step guide on how to tackle these scenarios. By the end of this post, you'll have a better grasp of how to apply trigonometric principles to real-world situations.
Understanding Trigonometry Basics

Before we dive into the problems, let’s briefly review some basic concepts:
- Sine, Cosine, and Tangent: These are the fundamental trigonometric functions, abbreviated as sin, cos, and tan respectively. Here’s a quick rundown:
- Sin(θ) = Opposite/Hypotenuse
- Cos(θ) = Adjacent/Hypotenuse
- Tan(θ) = Opposite/Adjacent
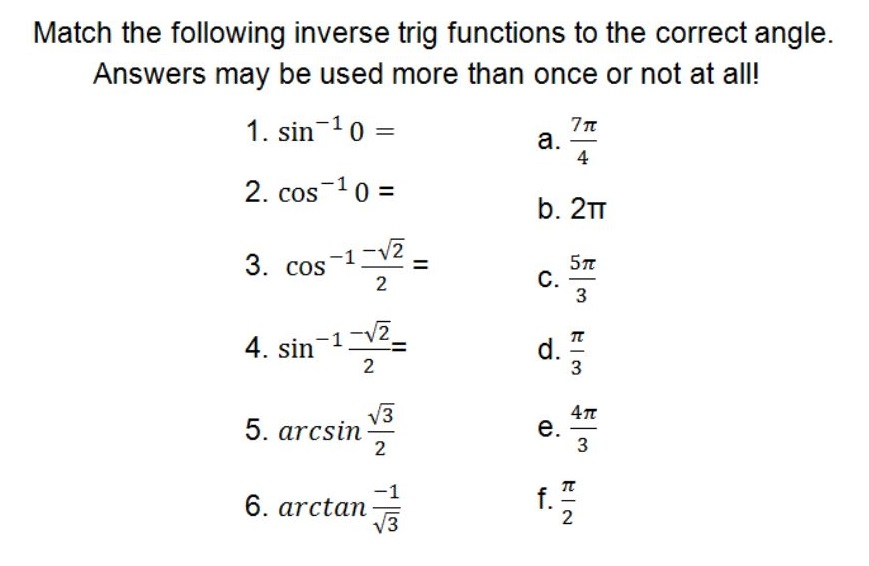
- Inverse Trigonometric Functions: These are denoted by arcsin, arccos, and arctan, which give us the angle when we know the ratio.
- Pythagorean Theorem: This theorem links the sides of a right triangle (a^2 + b^2 = c^2).
- Sine and Cosine of Complementary Angles: sin(θ) = cos(90° - θ).
Now, let’s solve some practical word problems!
Problem 1: Height of a Tree
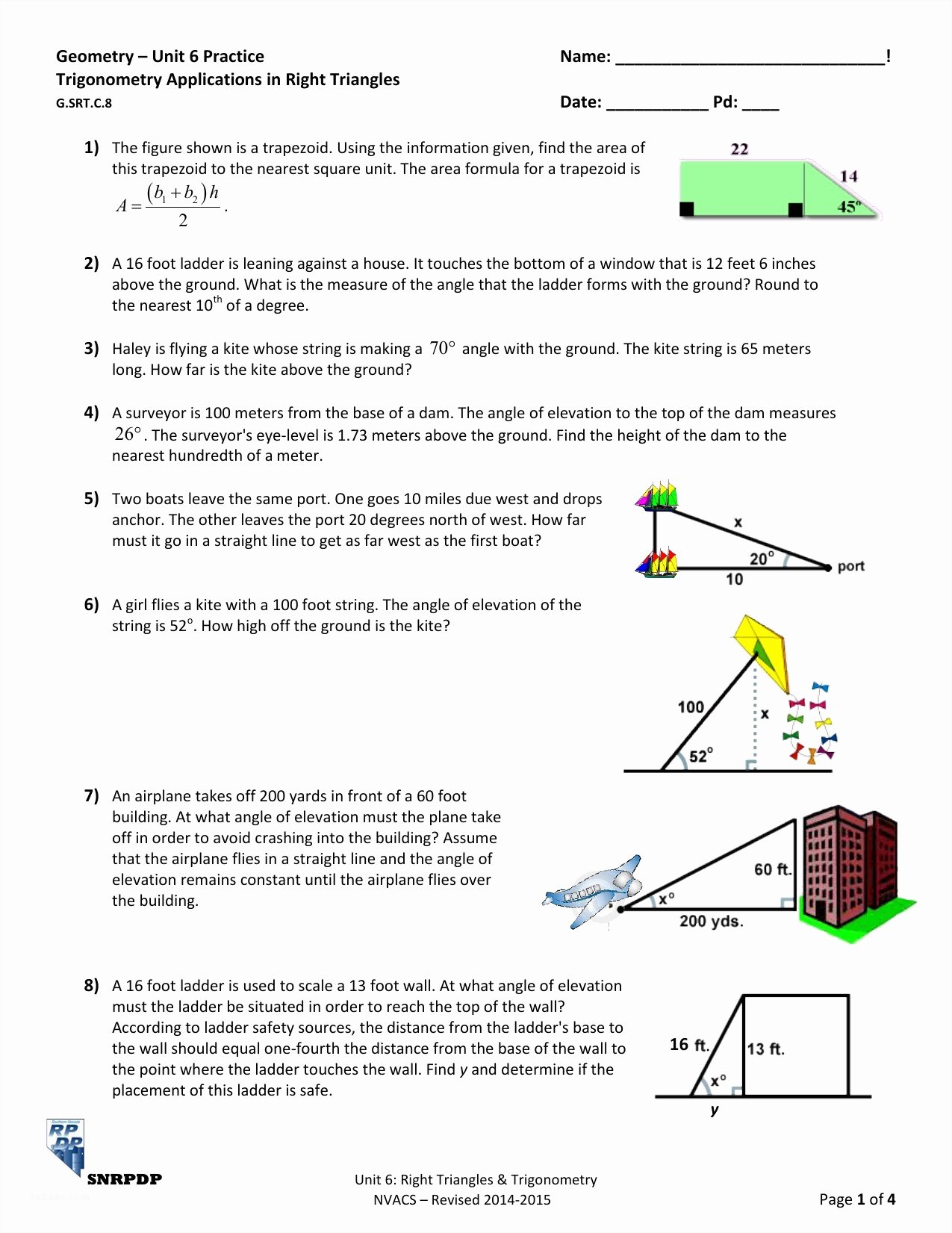
A tree casts a shadow that is 15 meters long. If the angle of elevation from the end of the shadow to the top of the tree is 30°, how tall is the tree?
Here’s how we solve it:
- Identify the triangle components:
- The shadow is the adjacent side to the angle (30°).
- The height of the tree is the opposite side to the angle.
- Use the tangent function:
tan(30°) = opposite/adjacent
height = 15 * tan(30°) ≈ 8.66 meters
🌳 Note: In real-world applications, the angle of elevation or depression can differ due to terrain or light conditions, so always take multiple measurements if possible.
Problem 2: Distance Across a Lake

From one side of a lake, the observer sees the top of a lighthouse at an angle of elevation of 25°. The height of the lighthouse is 50 meters, and the observer’s eye level is 1.5 meters above the ground. How far is the observer from the lighthouse?
- Identify the triangle components:
- The lighthouse height (48.5 meters - the difference between the top and the observer’s eye level) is the opposite side to the angle (25°).
- The distance across the lake is the adjacent side.
- Use the tangent function:
tan(25°) = opposite/adjacent
distance = (50 - 1.5) / tan(25°) ≈ 101.3 meters
🌊 Note: In scenarios involving bodies of water, atmospheric conditions can alter perceived distances or angles.
Problem 3: Elevator Angle
An elevator rises at an angle of 45° from the horizontal. If the horizontal distance covered is 12 meters, how high does the elevator rise?
- Identify the triangle components:
- The horizontal distance is the adjacent side to the angle (45°).
- The height is the opposite side to the angle.
- Use the tangent function:
tan(45°) = 1, so height = 12 meters
Problem 4: Tower’s Shadow Length

A tower casts a shadow 40 meters long when the sun’s elevation angle is 60°. Find the height of the tower.
- Identify the triangle components:
- The shadow length is the adjacent side to the angle (60°).
- The tower’s height is the opposite side to the angle.
- Use the tangent function:
tan(60°) = √3
height = 40 * √3 ≈ 69.28 meters
Problem 5: Angle of Depression

From a point on top of a cliff, the angle of depression to a boat is 40°. The height of the cliff above sea level is 80 meters. How far is the boat from the base of the cliff?
- Identify the triangle components:
- The cliff height is the opposite side to the angle of depression, which is the same as the angle of elevation when viewed from the boat.
- The distance from the base of the cliff to the boat is the adjacent side to the angle of elevation (50°).
- Use the tangent function:
tan(50°) ≈ 1.19175
distance = 80 / tan(50°) ≈ 67.2 meters
These five problems demonstrate how trigonometry can be used to solve real-life situations, from calculating distances to understanding angles in everyday contexts. By employing trigonometric functions like sine, cosine, and tangent, we've unraveled the math behind everyday phenomena. The key takeaways here are:
- Trigonometric functions provide a systematic approach to solving angular and spatial problems.
- Understanding the relationships between angles and sides in a right triangle is essential for applying trigonometry.
- Real-world conditions can introduce variables not considered in ideal scenarios, requiring practical adjustments.
As we've seen, trigonometry isn't just about solving for angles or lengths in abstract problems; it's a powerful tool for understanding the world around us, from the height of a tree to the distance across a lake. Whether you're a student, an engineer, or just someone curious about how things work, trigonometry offers invaluable insights into our physical environment, allowing us to quantify and predict the unseen dimensions that shape our lives.
What is the difference between sine, cosine, and tangent?

+
Sine (sin) is the ratio of the length of the opposite side to the hypotenuse. Cosine (cos) is the ratio of the adjacent side to the hypotenuse, and tangent (tan) is the ratio of the opposite side to the adjacent side in a right triangle.
How do I know which trigonometric function to use for a problem?

+
Choose your function based on the known sides and what you need to find:
- If you know the opposite side and the hypotenuse, use sine.
- If you know the adjacent side and the hypotenuse, use cosine.
- If you know both opposite and adjacent, use tangent.
Why do we use angles of elevation or depression in real-life problems?

+
Angles of elevation or depression are used to determine distances, heights, or angles in scenarios where direct measurement is impractical or impossible, like surveying land, measuring the height of buildings or mountains, or navigating ships and airplanes.
Can I use trigonometry for non-right triangles?

+
Yes, for non-right triangles, you would use the Law of Sines or the Law of Cosines to find angles or sides.
What are some applications of trigonometry outside of math class?

+
Trigonometry is used in fields like:
- Astronomy for calculating distances in space.
- Architecture and construction for designing structures.
- Navigation and surveying for determining positions.
- Computer graphics and game design for rendering 3D scenes.