Mastering Negative Fractions: Free Worksheet for Easy Learning
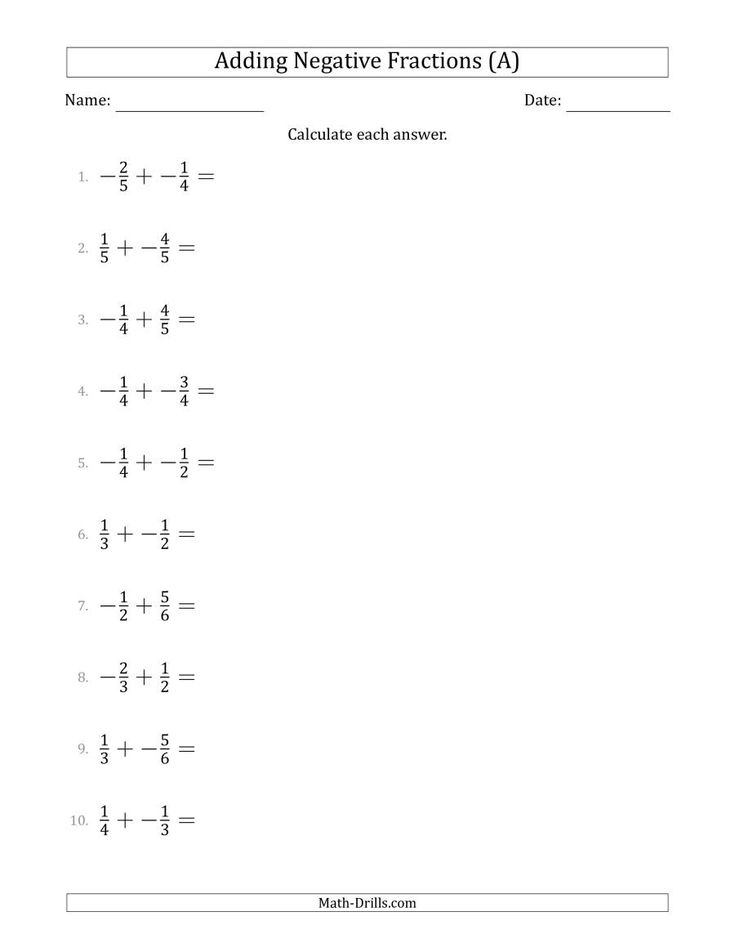
Negative fractions can often seem daunting, but they don't have to be. Understanding negative fractions is a critical part of mastering mathematics, both at the academic level and in real-world applications. This blog post will guide you through understanding negative fractions and how they work, offering insights and exercises through a free worksheet to simplify your learning journey. Whether you're a student struggling with negative fractions or a tutor looking for resources, this post aims to clear up common misconceptions and foster a solid grasp on this mathematical concept.
Understanding Negative Fractions
At its core, a negative fraction is simply a fraction where the numerator or the denominator or both are negative, resulting in a negative value. Here’s how they work:
- Negative Numerator: If the numerator is negative, the fraction is negative. For example, -3/4.
- Negative Denominator: A negative denominator with a positive numerator flips the fraction to negative. For instance, 3/(-4) = -3/4.
- Both Negative: If both the numerator and the denominator are negative, the negatives cancel out, and the fraction is positive. Like (-3)/(-4) = 3/4.
These rules ensure that the operations with negative fractions remain consistent with the rules of arithmetic for integers.
The Importance of Negative Fractions

Negative fractions play a crucial role in various fields:
- Finance: They help calculate debts, losses, and negative balances.
- Physics: Negative fractions are used to describe directions and quantities like negative velocities or accelerations.
- Chemistry: They are essential for understanding ions with a negative charge in chemical reactions.
These are just a few examples, showing that negative fractions are not just abstract concepts but have practical applications in real life.
Learning with Worksheets

A structured worksheet can be a powerful tool for learning negative fractions. Here’s how you can use a worksheet effectively:
Structure of a Good Worksheet

- Introduction to Concepts: A brief reminder of what negative fractions are, their properties, and how they are represented on a number line.
- Practice Problems: Exercises ranging from basic operations to more complex word problems involving negative fractions.
- Visual Aids: Include number lines and diagrams to help visualize the problems.
- Real-Life Scenarios: Problems that relate negative fractions to everyday situations to show relevance.
- Answer Key: Provide answers or explanations so learners can check their work and understand their mistakes.
| Topic | Example Problem |
|---|---|
| Adding Negative Fractions | -3/4 + (-1/2) = ? |
| Subtracting Negative Fractions | 1/3 - (-2/3) = ? |
| Multiplying and Dividing | (-1/2) * (-2/3) = ? |

💡 Note: Worksheets should also include a mix of positive and negative fractions to ensure a well-rounded practice.
Using the Worksheet

Here’s how you can maximize the learning from a negative fractions worksheet:
- Work Through Sequentially: Start with the simplest problems and gradually tackle the more complex ones.
- Check Each Step: Don't rush through problems. Ensure each step in your calculation is correct to avoid cumulative errors.
- Review Mistakes: Look at the answers to understand where you went wrong and why.
- Practice Regularly: Regular practice with worksheets can significantly improve your proficiency with negative fractions.
📝 Note: Consistency is key in mastering negative fractions. Dedicate time daily to practicing.
Why Practice is Essential

Understanding concepts is one thing; being able to apply them fluently is another:
- Flexibility: It helps you become flexible with operations, so you can solve problems in various contexts.
- Error Reduction: The more you practice, the less likely you are to make calculation errors.
- Confidence Building: Solving problems correctly boosts your confidence in your mathematical abilities.
Wrapping Up

Negative fractions might seem tricky at first, but with consistent practice and understanding, they can become as straightforward as positive fractions. This blog post has delved into what negative fractions are, why they are important, and how you can effectively use a worksheet to master them. By understanding the rules governing negative fractions and applying them through regular practice, you’ll enhance your mathematical prowess. Remember, the real-world relevance of negative fractions makes them not just an academic exercise but a valuable skill in various disciplines. Keep practicing, stay curious, and you’ll find that negative fractions will soon be second nature to you.
What are the rules for negative fractions?

+
The rules for negative fractions include: if the numerator is negative, the fraction is negative; if the denominator is negative, the fraction flips to negative; if both are negative, the negatives cancel out, making the fraction positive.
How can I practice negative fractions at home?

+
You can practice negative fractions at home by using free online worksheets, creating your own problems based on real-life scenarios, or using educational apps that focus on math skills.
Why are negative fractions important?

+
Negative fractions are important because they are used in finance for calculating losses or debts, in physics for denoting direction, in chemistry for charge balance, and in numerous other practical applications where negative quantities need to be represented and manipulated.