Mastering Pythagorean Theorem: Worksheet Answers Explained

Mathematics often brings us back to fundamental principles that form the bedrock of advanced concepts. One such principle is the Pythagorean Theorem. This post is dedicated to deconstructing the complexities of this theorem by providing answers to some worksheet questions, helping you master this timeless mathematical theorem.
Understanding the Pythagorean Theorem

The Pythagorean Theorem, often credited to the ancient Greek philosopher Pythagoras, is an equation that relates the three sides of a right triangle. In simple terms, it states:
- a2 + b2 = c2
Where a and b are the legs of the triangle, and c is the hypotenuse, the side opposite the right angle. Understanding this theorem is crucial for numerous applications in geometry, physics, and engineering.
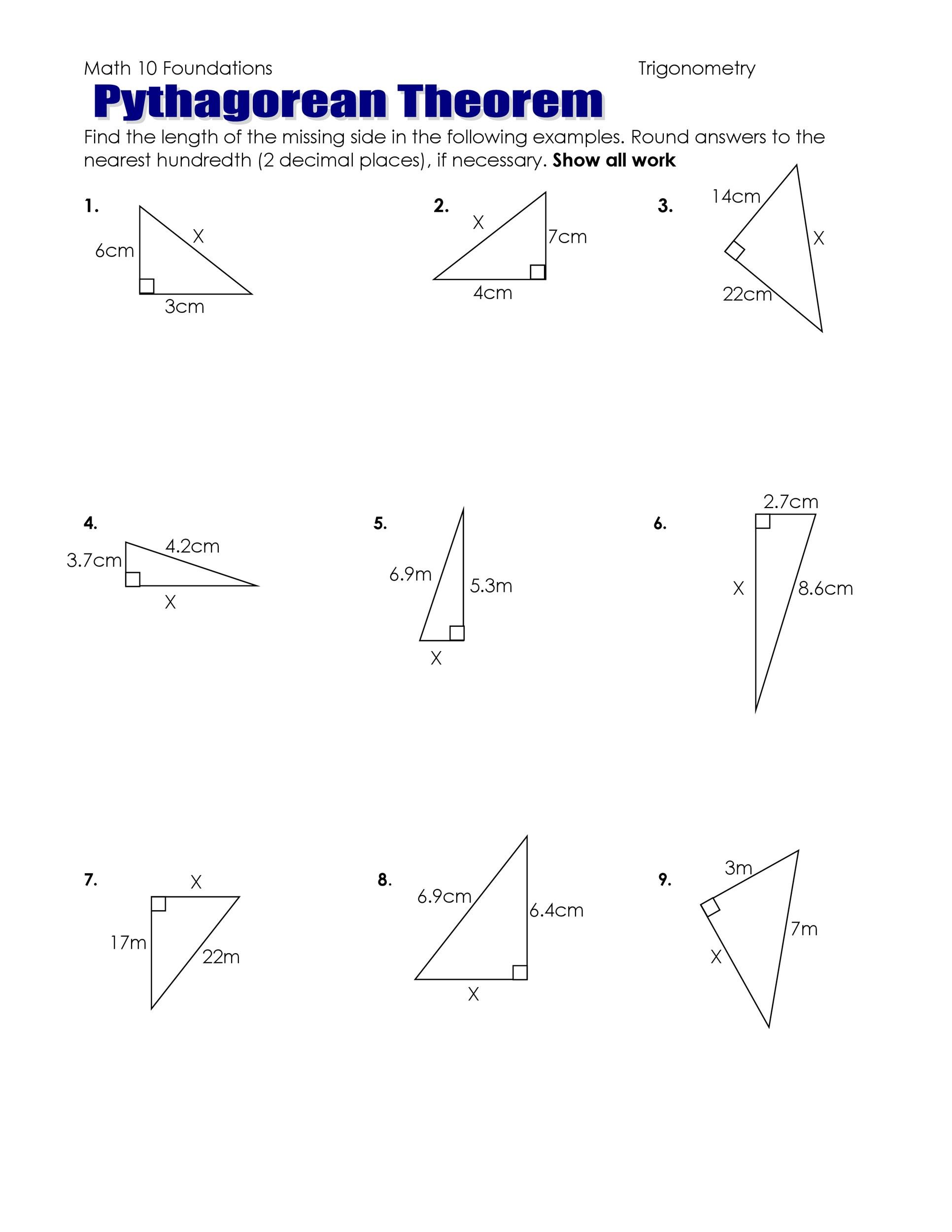
Worksheet Questions and Answers

Let's dive into some typical worksheet questions to see how the Pythagorean Theorem is applied:
Question 1: Finding the Hypotenuse

If the lengths of the legs of a right triangle are 3 and 4 units, find the length of the hypotenuse.
Solution:
Using the Pythagorean Theorem:
- 32 + 42 = c2
- 9 + 16 = c2
- 25 = c2
- c = √25
- c = 5
📝 Note: Always take the positive square root when solving for the length of a side.
Question 2: Finding One of the Legs

A right triangle has a hypotenuse of 13 units and one leg measuring 5 units. Find the length of the other leg.
Solution:
Again, we’ll use the Pythagorean Theorem, but this time, we solve for a:
- 52 + a2 = 132
- 25 + a2 = 169
- a2 = 144
- a = √144
- a = 12
📝 Note: Here, we ensure to take the positive square root to find a valid side length.
Question 3: Practical Applications

The distance between two points in a coordinate system can also be found using the Pythagorean Theorem. If point A is at coordinates (2, 3) and point B is at (5, 7), find the distance between A and B.
Solution:
We treat the coordinates as the endpoints of the triangle’s legs:
- The horizontal distance (leg) is the difference in x-coordinates: 5 - 2 = 3
- The vertical distance (leg) is the difference in y-coordinates: 7 - 3 = 4
- Now, we apply the theorem: 32 + 42 = d2
- 9 + 16 = d2
- 25 = d2
- d = √25
- d = 5
Expanding into Non-Right Triangles

While the Pythagorean Theorem is specifically designed for right triangles, its principles can be extended to non-right triangles through trigonometric functions or via the Law of Cosines, which is a generalization of the Pythagorean Theorem. Here's how:
The Law of Cosines
For any triangle, we can calculate the length of a side using:
- a2 = b2 + c2 - 2 * b * c * cos(A)
📝 Note: Here, a is the side opposite angle A, and the theorem allows for non-right angle calculations.
Conclusion

Through our journey from basic applications to more complex scenarios, we've seen how the Pythagorean Theorem not only stands as a cornerstone in basic geometry but also expands to form the basis for broader mathematical relationships. Mastery of this theorem enhances your analytical skills, helping you solve real-world problems with ease and precision. Whether you're calculating distances in a coordinate system or exploring the properties of various triangles, the Pythagorean Theorem provides a simple yet profound insight into the relationships between sides of triangles.
Why can’t the hypotenuse be shorter than the other sides?

+
In a right triangle, the hypotenuse is the longest side because it is the sum of the squares of the other two sides. Thus, it physically and mathematically must be longer than either leg.
Is the Pythagorean Theorem valid for non-right triangles?

+
The classic form of the Pythagorean Theorem applies only to right triangles. However, the Law of Cosines extends its principles to all types of triangles.
How do I apply the Pythagorean Theorem to distances in coordinates?

+
You can treat the horizontal distance between points as one leg, the vertical distance as another leg, and calculate the distance between the points as the hypotenuse.



