Free Printable Adding Exponents Worksheet for Easy Learning

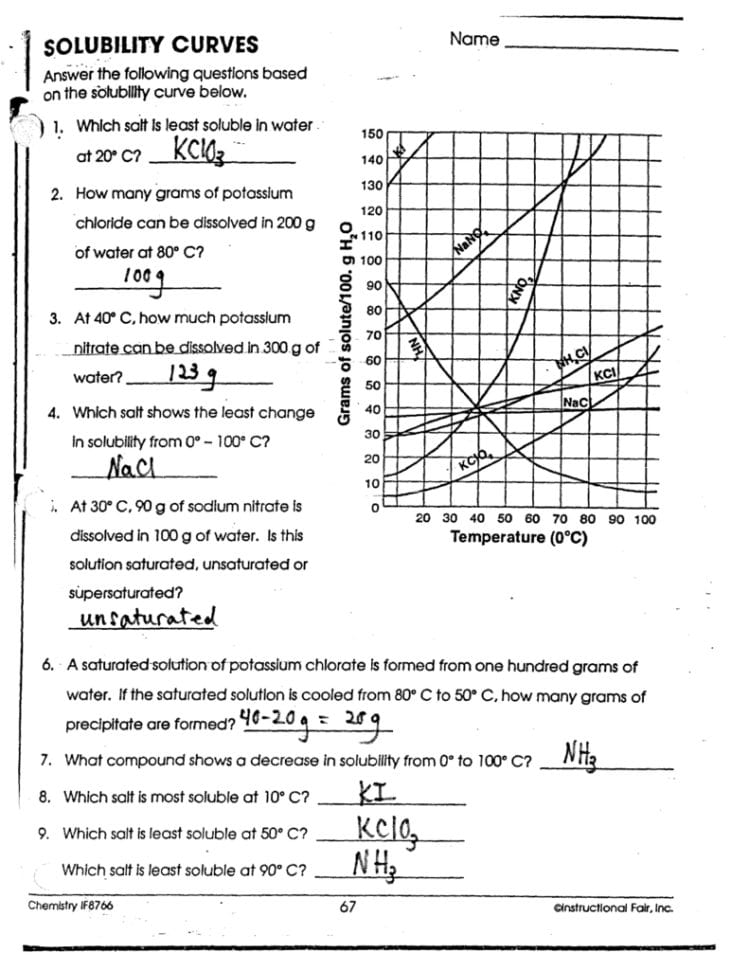
Welcome to this comprehensive guide on adding exponents with our free, printable worksheets. Today, we dive into the world of mathematics where understanding exponents can significantly ease problem-solving processes. Whether you are a student trying to grasp the concept or a teacher seeking resources to explain it, this post will offer you a structured approach to teaching and learning addition with exponents through practical exercises.
What Are Exponents?
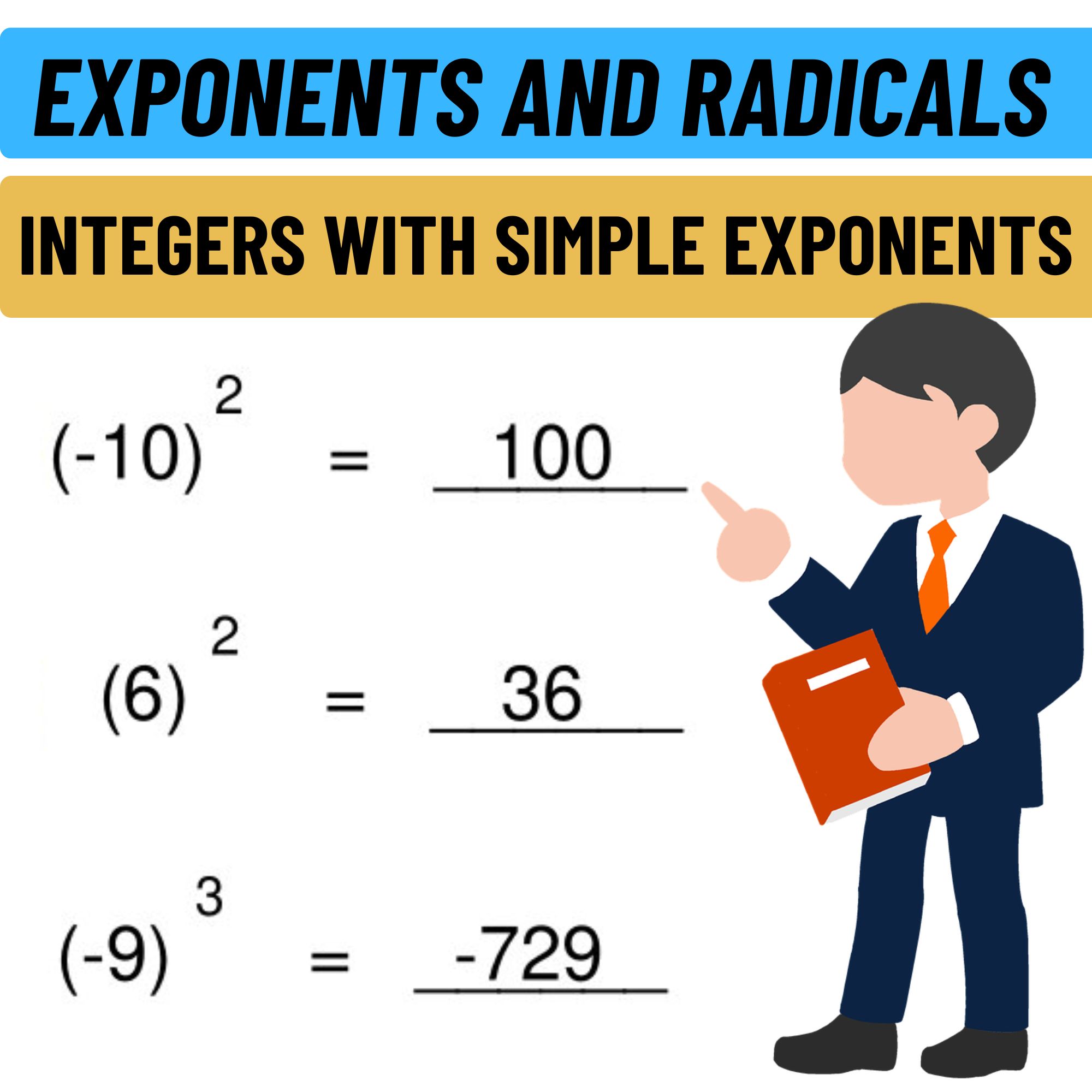

Exponents, also known as powers or indices, are shorthand notation for repeated multiplication. For example, 2^3 means 2 * 2 * 2, which equals 8. Understanding exponents is fundamental in algebra, calculus, and various other mathematical and scientific fields.
Why Learn to Add Exponents?
Adding exponents isn’t as simple as adding the base numbers. Here’s why it’s important to learn:
- Enhances Problem Solving: Exponent addition is essential for simplifying expressions.
- Simplifies Complex Equations: Knowledge of adding exponents allows for quicker, cleaner solutions.
- Foundation for Advanced Math: Higher mathematics frequently involves exponential functions.
How to Add Exponents: The Basic Rules

Before we delve into our free printable worksheets, let’s refresh the rules for adding exponents:
- Same Base, Same Exponent: When adding like terms, simply add the coefficients (numbers in front of the variables).
- Example: 2x + 3x = 5x
- When Exponents Differ: Here’s where it gets tricky. You can’t add exponents directly unless the bases and exponents are the same.
- Example: You can’t directly add 2^3 + 2^2 because the exponents differ.
To add exponents with different powers, you’ll need to use the distributive property or factoring in some cases.
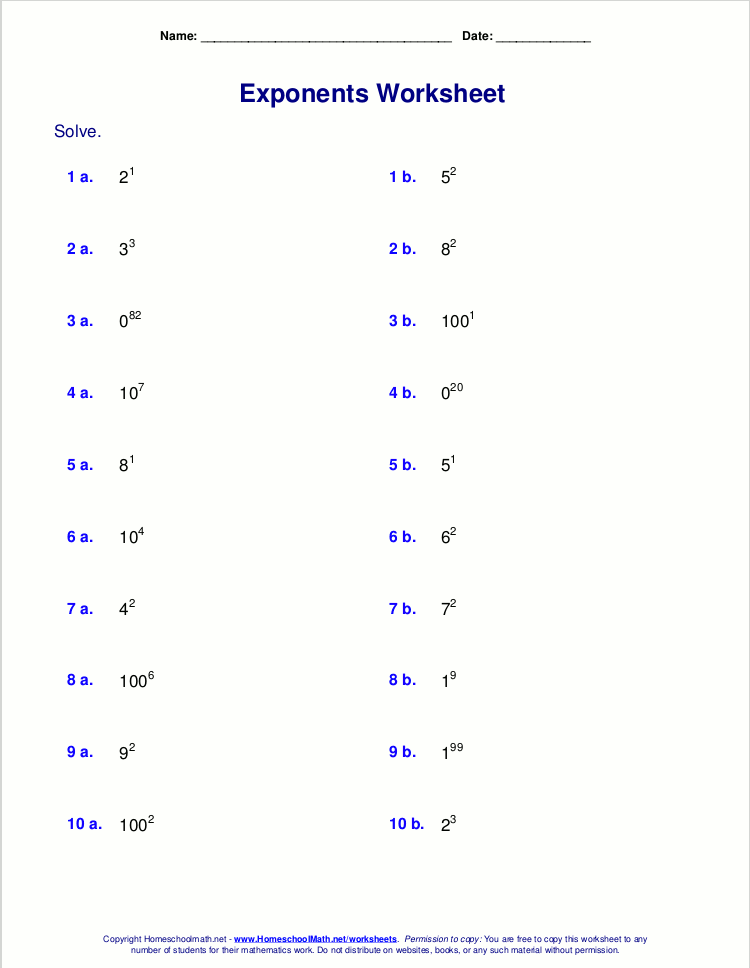
Worksheet Creation: A Step-by-Step Guide

Let’s create some printable adding exponents worksheets tailored for different learning levels:
- Choose the Age Group: Determine whether it’s for elementary, middle, or high school students.
- Select Difficulty: Pick between basic (same base, same exponent) or more advanced (using algebraic manipulation).
- Design Format:
- Problems on left, space for answers on right
- Multiple-choice questions for younger students
- Mixed problems for more advanced learners
- Create Examples: Provide clear, worked examples before the problems. For instance:
Example: 3x + 4x = 7x - Make Printable: Use a design tool or word processor to make the worksheet visually appealing and clear.
Sample Worksheet

| Problem | Answer |
|---|---|
| 5x + 2x = | |
| 4y² + y² = | |
| 2^3 + 2^3 = |

📝 Note: Ensure your worksheet has an answer key at the end for self-assessment or teacher verification.
Using Worksheets for Learning

Incorporating these worksheets into learning sessions provides:
- Practice: Reinforces concepts through repetition.
- Visualization: Helps in understanding how exponents work visually.
- Assessment: Allows students and teachers to track progress.
Summing up, adding exponents can seem daunting at first, but with our free printable worksheets, it becomes an enjoyable learning experience. We've explored the essentials of exponents, why they're important, the rules for adding them, and how to create and utilize worksheets effectively. By practicing regularly with these resources, students will gain confidence in their mathematical abilities, opening doors to more complex math topics down the line.
What are the basic rules for adding exponents?

+
When adding exponents, you can only add directly if the bases and exponents are identical. For instance, 3x + 4x = 7x. If the exponents or bases differ, you need to use algebraic properties to simplify or factor the expression first.
How can I help students understand adding exponents?

+
Start with visual examples, show how exponents work in real-life scenarios (like compound interest or population growth), and use manipulatives or diagrams to make the concept tangible. Use worksheets for repeated practice, reinforcing the rules in various contexts.
Can you add exponents with different bases?

+
Adding exponents with different bases isn’t possible without simplifying or factoring first. However, if you have something like x + y, these terms can be written side by side without addition because they represent different variables.