Master Adding and Subtracting Radicals with Ease

Understanding how to add and subtract radicals can unlock a whole new dimension of algebraic skills, enabling you to tackle more complex mathematical problems with ease. Radicals, or roots, are expressions involving the radical symbol (√), which represents a square root or other higher-order roots like cube roots, fourth roots, and so on. This guide will walk you through the process of adding and subtracting radicals, highlighting key steps and providing practical examples to help solidify your understanding.

Understanding Radicals

Before we delve into the arithmetic of radicals, it's essential to grasp their basic concept:

• Radical expression: An expression that uses a root, typically square roots (√), cube roots (³√), etc.
• Index: The number indicating the root to be taken, which is 2 for square roots, 3 for cube roots, and so forth.
• Radical: The number or expression under the radical symbol.

The Basics of Adding Radicals

Adding radicals is akin to combining like terms in algebra, but with an additional layer of complexity:

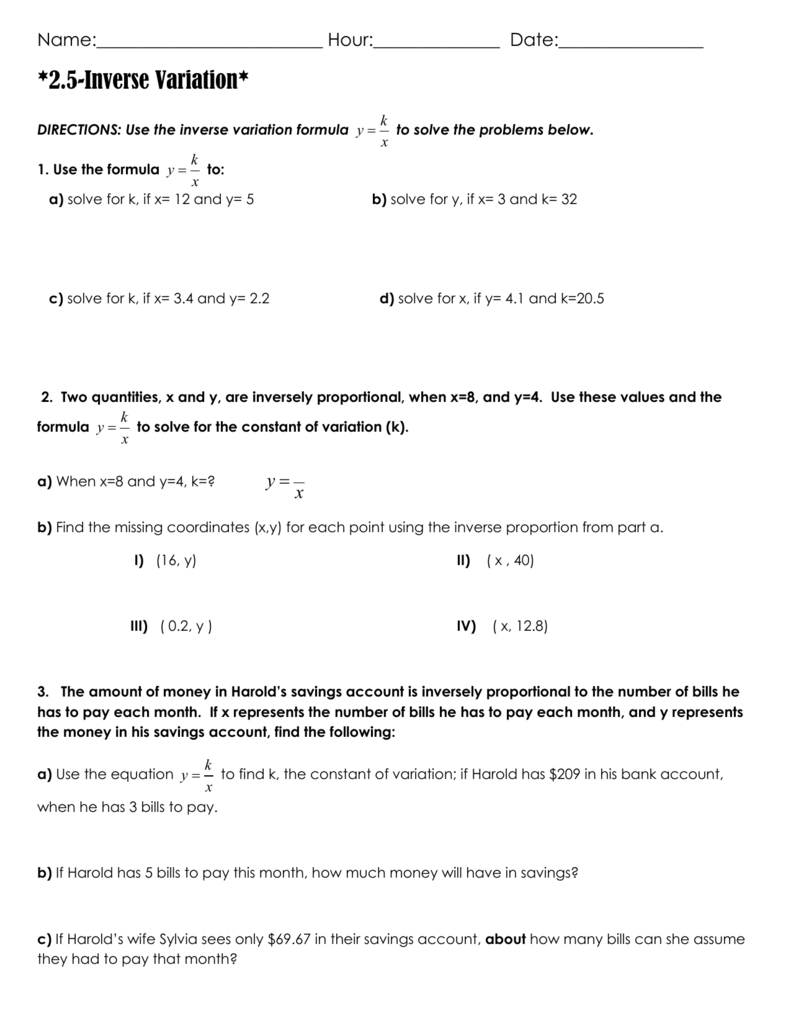
• Like radicals: You can only add radicals that have the same index and radicand. For instance, √3 + √3 equals 2√3.
• Unlike radicals: If the radicals do not match in both index and radicand, you cannot directly add them. For example, √2 + √5 cannot be added without further manipulation.

Here's how you go about adding like radicals:

1. Identify like terms (radicals with the same radicand and index).
2. Combine these terms by adding the coefficients in front of the radical symbol.
3. Write the result with the radical in its simplest form.

⚠️ Note: Ensure that you are only combining radicals when they are identical in both index and radicand.

Example:

• Add √3 + 4√3:

    √3 + 4√3 = (1 + 4)√3 = 5√3

The Mechanics of Subtracting Radicals

The process for subtracting radicals follows similar rules as adding:

• Like radicals: You can subtract like radicals just as you would add them by subtracting the coefficients.
• Unlike radicals: They must be simplified or made alike before subtraction can occur.

The steps are:

1. Identify like terms (radicals with the same radicand and index).
2. Subtract the coefficients in front of the radical symbol.
3. Write the result in its simplest form.

Example:

• Subtract 2√2 - √2:

    2√2 - √2 = (2 - 1)√2 = √2

Simplifying Radicals before Combining

Sometimes, radicals need to be simplified before they can be combined:

• Factorization: Factorize the radicands to see if you can simplify square roots or other roots.
• Simplify: If the radicands have a perfect square (for square roots) or a perfect cube (for cube roots) factor, you can simplify the radical.

🧠 Note: Simplifying radicals can often reveal like terms that weren't apparent initially.

Example:

• Add √8 + √50:

Combining Multiple Radicals

When dealing with expressions that involve multiple radicals, follow these steps:

1. Identify and simplify any radicals where possible.
2. Group like radicals together.
3. Add or subtract the coefficients of the like radicals.
4. Combine any remaining radicals if they are alike.

This process can get intricate, requiring careful attention to detail:

Example:

• Combine 5√3 + 2√7 - 3√3 + 12:

    5√3 + 2√7 - 3√3 + 12
    = (5√3 - 3√3) + 2√7 + 12
    = 2√3 + 2√7 + 12

Real-World Applications

Adding and subtracting radicals isn't just an abstract algebraic exercise. Here are a few practical scenarios where these skills are useful:

• Physics: In physics, radicals appear when calculating distances or velocities using vectors, where vector addition involves radicals.
• Engineering: Engineers often encounter radical expressions in stress analysis, fluid dynamics, and electrical circuits.
• Finance: Risk assessment models in finance can involve the use of square roots to compute standard deviations.

💡 Note: While these examples highlight the utility of radicals, real-world problems often involve more complex calculations that might require advanced mathematical tools.

The final key to mastering the addition and subtraction of radicals lies in consistent practice and understanding the underlying principles. By recognizing like terms, simplifying radicals when necessary, and applying these skills to real-life problems, you can enhance your mathematical proficiency. Each time you tackle a problem involving radicals, you're not only solving that problem but also building a foundation for future challenges in algebra and beyond.

Can you add radicals with different indices?

+

No, radicals with different indices cannot be directly added or subtracted. However, you can convert one radical to match the other by raising or lowering the index and adjusting the radicand accordingly. Then, you can combine the like terms if they become identical.

What if I encounter a radical with a fraction?

+

When dealing with a radical that has a fraction inside it, you can use the property of roots that says the square root of a fraction is the square root of the numerator divided by the square root of the denominator. Then, you can simplify or combine if possible.

Are there shortcuts or tricks for adding or subtracting radicals?

+

The main “trick” is recognizing and simplifying like terms. Also, learning how to use the distributive property effectively, especially when dealing with binomial or polynomial radicals, can make operations much easier.