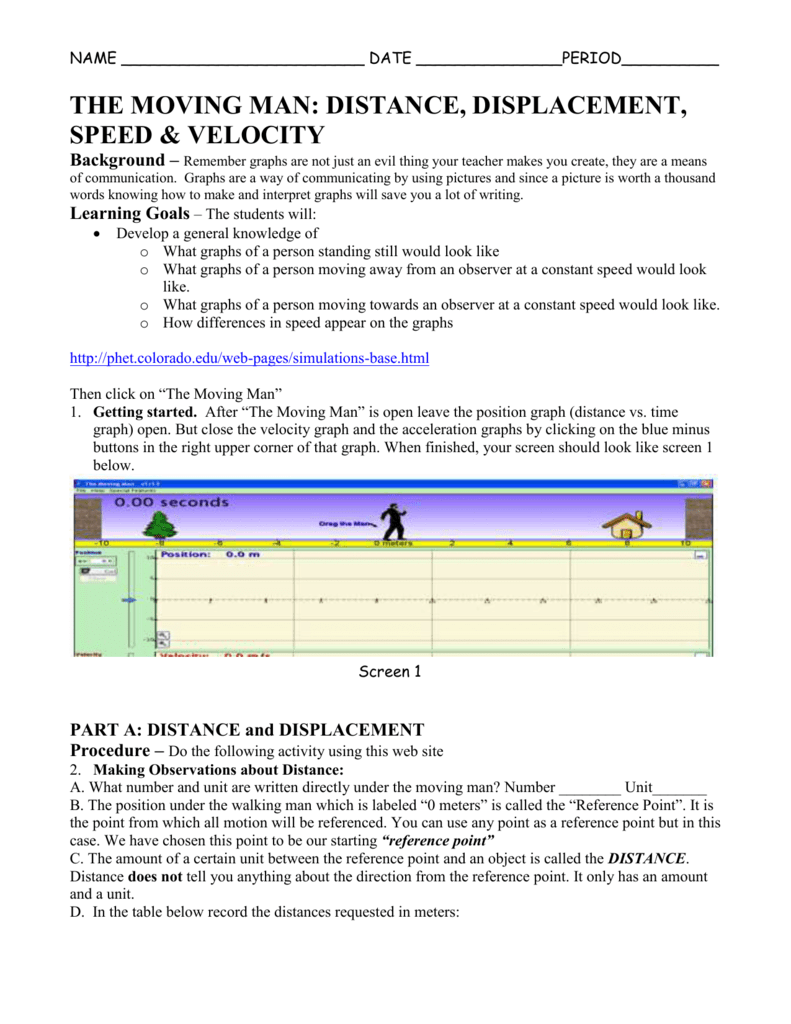
5 Kinetic and Potential Energy Worksheet Problems Explained

Understanding the Basics of Kinetic and Potential Energy

Before we delve into specific problems, let’s briefly review the core concepts of kinetic and potential energy. Kinetic energy refers to the energy an object possesses due to its motion, calculated by the equation KE = 1/2 * m * v2 where m is the mass and v is the velocity of the object. On the other hand, potential energy is the stored energy an object has due to its position or configuration, often related to gravitational potential energy which is given by PE = m * g * h, where g is the gravitational acceleration, and h is the height above a reference level.

Problem 1: Ball Falling from a Roof
Imagine a ball of mass 0.5 kg is dropped from a roof 15 meters high. Find its velocity just before it hits the ground.
- Calculate the potential energy: PE = m * g * h = 0.5 * 9.8 * 15 = 73.5 J
- Since all potential energy is converted to kinetic energy at the ground: KE = PE = 73.5 J
- Solve for velocity: 73.5 = 1/2 * 0.5 * v2 => v2 = 73.5 / 0.25 = 294 => v = √294 = 17.15 m/s
⚠️ Note: This is an ideal scenario assuming no air resistance or energy losses due to heat or sound.
Problem 2: Roller Coaster at Different Points

A roller coaster car with a total mass of 500 kg is at the top of a 30-meter hill, moving with an initial speed of 10 m/s. What is the velocity at the bottom of the hill?
- Potential Energy at top: PE = 500 * 9.8 * 30 = 147,000 J
- Kinetic Energy at top: KE = 1/2 * 500 * 102 = 25,000 J
- Total energy: 147,000 + 25,000 = 172,000 J
- At the bottom, all energy is kinetic: 172,000 = 1/2 * 500 * v2
- Solve for velocity: v2 = 172,000 / 250 = 688 => v = √688 ≈ 26.23 m/s
Problem 3: Swing Pendulum

A child on a swing reaches a height of 2 meters at the peak. Calculate their velocity at the lowest point of the swing if their mass is 25 kg.
- Potential Energy at top: PE = 25 * 9.8 * 2 = 490 J
- At the bottom, all potential energy converts to kinetic energy: 490 = 1/2 * 25 * v2
- Solve for velocity: v2 = 490 / 12.5 = 39.2 => v = √39.2 ≈ 6.26 m/s
⚠️ Note: The swing’s arc and the child’s position can affect the accuracy of these calculations in real-world scenarios.
Problem 4: Bungee Jumping

A bungee jumper of 70 kg jumps from a bridge 50 meters high. The jumper reaches a point 10 meters below the bridge before bouncing back. Find the jumper’s velocity at that point.
- Calculate potential energy at bridge height: PE = 70 * 9.8 * 50 = 34,300 J
- Calculate potential energy at 10 meters below the bridge: PE = 70 * 9.8 * (50-10) = 27,440 J
- Energy difference: 34,300 - 27,440 = 6,860 J (lost to air resistance and bungee cord stretching, but we’ll use conservation of energy for simplicity)
- Solve for velocity: 6,860 = 1/2 * 70 * v2 => v2 = 6,860 / 35 = 196 => v = √196 ≈ 14 m/s
Problem 5: Spring Compression

A 1 kg block compresses a spring by 20 cm (k = 100 N/m). What is the maximum height the block reaches when released?
- Spring potential energy: PE = 1/2 * k * x2 = 1/2 * 100 * 0.22 = 2 J
- This energy will lift the block: m * g * h = 2 => h = 2 / (1 * 9.8) ≈ 0.204 m
Throughout these problem explanations, we've not only applied the principles of kinetic and potential energy but also looked at real-world scenarios where these energies are commonly encountered. Understanding how energy transforms from one form to another is key to grasping many natural phenomena and engineering applications. These examples help us see the practical uses of these fundamental physics concepts, from amusement park rides to understanding the dynamics of simple objects in motion.
What happens if energy is lost due to friction or air resistance?

+
When energy is lost due to friction or air resistance, the total mechanical energy (kinetic + potential) decreases because some of the energy is converted into thermal or sound energy. This means that the calculations involving conservation of energy would need to account for these losses.
How does mass affect kinetic and potential energy?

+
Both kinetic and potential energy are directly proportional to mass. More mass increases the potential energy at a given height or the kinetic energy at a given velocity.
Can kinetic energy ever be negative?

+
No, kinetic energy is always positive or zero because it depends on the square of velocity, which itself is always positive or zero.