Phet Moving Man Worksheet Answers: Ultimate Guide
Explore how physics and software simulation can intertwine in an educational context with our guide on the "Phet Moving Man Worksheet Answers." For students and teachers alike, Phet's "Moving Man" simulation offers a dynamic learning environment where physics comes to life. This guide not only provides answers to the worksheet but also offers deeper insights into the physics principles demonstrated.
Introduction to Phet Moving Man Simulation

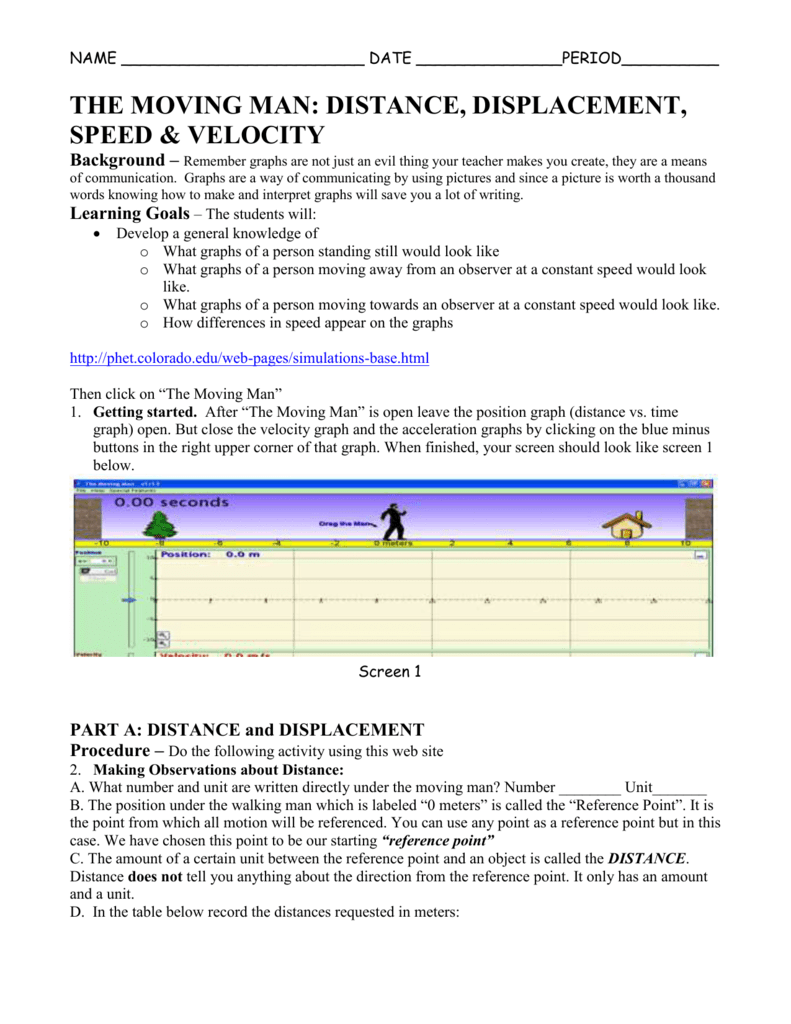
The PhET Interactive Simulations at the University of Colorado Boulder have created an effective tool for visualizing the abstract concepts of kinematics with their "Moving Man" simulation. This simulation allows users to:
- Manipulate the speed, velocity, and acceleration of a virtual man.
- Observe the effects on his movement in real-time.
- Learn about the relationships between position, velocity, and acceleration through graphs.
Understanding the Worksheet Questions

The worksheet typically includes questions that explore various physics concepts. Here, we will delve into some common questions and their answers:
What is the relationship between velocity and position?

Velocity is the rate of change of position with respect to time. This relationship can be understood through the slope of the position-time graph:
- If the slope is positive, the velocity is positive, indicating the man is moving to the right.
- If the slope is negative, the velocity is negative, indicating movement to the left.
- A zero slope (flat line) signifies no movement, hence the velocity is zero.
How does acceleration affect velocity?

Acceleration is the rate of change of velocity. Here’s how it interacts:
- Positive acceleration will increase the velocity.
- Negative acceleration (deceleration) will decrease the velocity.
- No acceleration means constant velocity.
Position vs. Time Graph Analysis

Using the “Moving Man” simulation:
- Create scenarios where the man starts from rest, accelerates, and reaches a constant speed.
- Observe how the position-time graph changes with:
- A straight line when moving at constant velocity.
- A curve when accelerating or decelerating.
Velocity vs. Time Graph Analysis

When you plot velocity against time:
- A flat line represents constant velocity.
- An upward or downward slope represents acceleration or deceleration, respectively.
- The area under the curve of this graph represents displacement, providing insights into the man's position over time.
Key Insights from Simulation

Below is a summary of how different scenarios can be created in the simulation for educational purposes:
| Scenario | Velocity | Acceleration | Graph Shape |
|---|---|---|---|
| Constant Velocity | Positive | Zero | Straight Line |
| Positive Acceleration from Rest | Increasing from zero | Positive | Upward Concave |
| Negative Acceleration (Deceleration) | Decreasing | Negative | Downward Concave |

⚠️ Note: The simulation can only simulate idealized situations, neglecting real-world factors like air resistance or friction, which can influence actual physical outcomes.
Final Insights

In this comprehensive guide, we've delved into the essential aspects of using the Phet "Moving Man" simulation for educational purposes. By exploring the connection between position, velocity, and acceleration, educators and learners can develop a deeper understanding of physics principles in a controlled, visual environment. The simulation provides not just answers but an interactive platform for exploration, making learning both fun and insightful.
What does the slope of the velocity-time graph represent?

+
The slope of the velocity-time graph represents acceleration. A positive slope means acceleration, and a negative slope indicates deceleration.
How can I simulate deceleration in the “Moving Man” simulation?

+
Set a negative acceleration value to simulate deceleration. The man will slow down until he stops or reverses direction, depending on the initial conditions.
Can real-world physics problems be accurately solved using this simulation?

+
While the simulation is excellent for basic principles, it simplifies many real-world factors like air resistance, friction, or non-linear forces, which can affect practical applications.