Mastering Volume Calculations: Cylinders, Cones, Spheres Worksheet

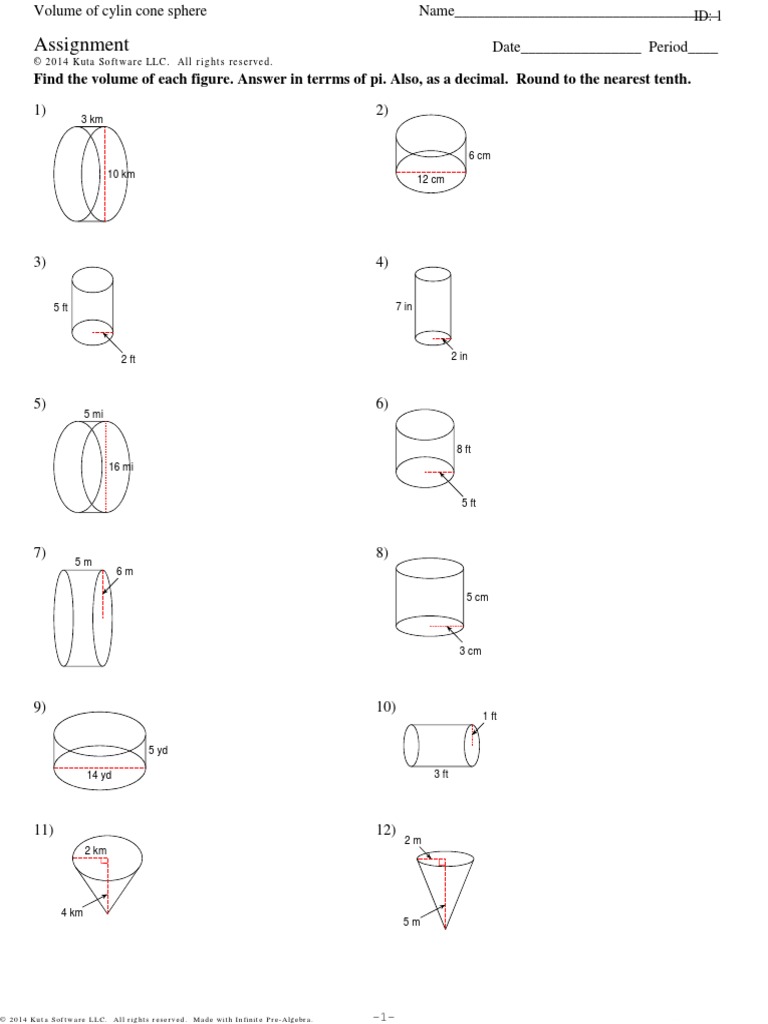
Understanding volume calculations is essential for students and professionals in various fields, ranging from engineering to baking. Today, we're going to dive deep into the volume calculations for three common geometric shapes: cylinders, cones, and spheres. This guide will provide detailed instructions, practical examples, and helpful tips to master these calculations, making them both useful for educational purposes and real-world applications.

Volume of a Cylinder

The volume of a cylinder is calculated by the formula:

V = πr²h

Here’s how to approach this:

• Identify the radius ®: This is the distance from the center of the base to its edge.
• Measure the height (h): The perpendicular height from the base to the top of the cylinder.
• Use π (pi), which is approximately 3.14159.
• Calculate the volume by multiplying these variables together.

📏 Note: Always ensure your measurements for radius and height are in the same units for accurate results.

Practical Example

Let’s consider a cylinder with a radius of 5 cm and a height of 10 cm:

V = π * 5² * 10

V ≈ 3.14159 * 25 * 10

V ≈ 785.3975 cm³

Volume of a Cone

The volume of a cone can be found using:

V = ¹⁄₃ * πr²h

Here are the steps:

• Find the radius ®: Similar to the cylinder, it’s the distance from the center to the edge of the base.
• Determine the height (h): The distance from the center of the base to the apex of the cone.
• Plug these values into the formula, along with π.

Practical Example

If you have a cone with a base radius of 6 cm and a height of 10 cm:

V = 1⁄3 * π * 6² * 10

V ≈ ¹⁄₃ * 3.14159 * 36 * 10

V ≈ 376.9911 cm³

Volume of a Sphere

The volume of a sphere is given by:

V = ⁴⁄₃ * πr³

Here’s how you calculate it:

• Identify the radius ®: The distance from the center to the surface of the sphere.
• Plug the radius into the formula along with π.

Practical Example

Consider a sphere with a radius of 4 cm:

V = 4⁄3 * π * 4³

V ≈ ⁴⁄₃ * 3.14159 * 64

V ≈ 268.0826 cm³

⚠️ Note: The formula for a sphere uses the cube of the radius, making it sensitive to changes in size.

Understanding these volume calculations can not only help in academic settings but also in practical scenarios like manufacturing, cooking, or even in determining the capacity of containers. The formulas are simple once you understand the logic behind them, and with practice, calculating these volumes becomes second nature.

The recap of what we've learned:

• The cylinder has a volume formula that accounts for the area of its circular base times its height.
• The cone's volume is one-third of what it would be if it were a cylinder of the same base and height.
• The sphere's volume formula reflects its three-dimensional symmetry.

Now, let's move on to some frequently asked questions to solidify our understanding of these volume calculations.

Can I use different units for radius and height when calculating volumes?

+

It’s recommended to use consistent units for accurate calculations. Mixing units will lead to incorrect volume measurements.

What happens if you double the radius or height of a cone or cylinder?

+

If you double the radius, the volume increases by a factor of 4 because volume is proportional to the square of the radius. Doubling the height will double the volume for both shapes.

Why does the sphere formula include a factor of ⁴⁄₃?

+

This fraction comes from calculus and integrates to give the correct volume for a three-dimensional sphere. It relates to the surface area of a sphere and its three-dimensional nature.

How do you find the volume if you know the surface area?

+

Finding the volume from the surface area directly isn’t straightforward since the surface area doesn’t give you a unique shape. However, if you know the shape, you can use the specific formula for that shape to derive the radius or height, then calculate the volume.