The Remainder And Factor Theorems Worksheet Answers

Polynomials and algebra often involve complex problem-solving techniques, with the Remainder Theorem and Factor Theorem playing a pivotal role. Here's a comprehensive look at these fundamental concepts with detailed worksheet answers:
Understanding the Remainder Theorem
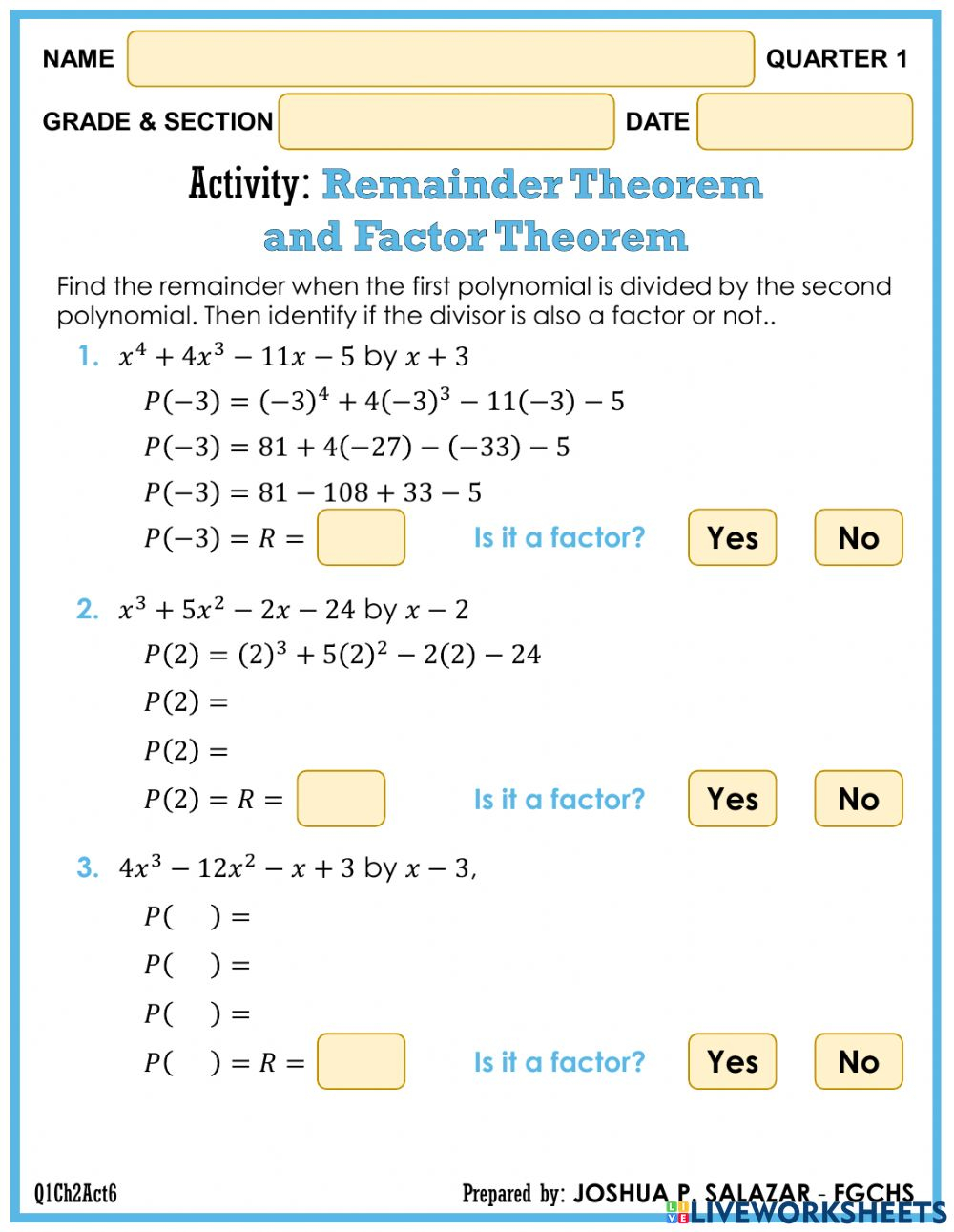
The Remainder Theorem states that for a polynomial f(x) and a value c , the remainder of dividing f(x) by (x - c) is exactly f(c) . This theorem simplifies the process of finding remainders without long division:
- If f(x) = 3x^3 - 7x^2 + 2x - 5 , and c = 2 , then the remainder is f(2) .
- Calculate f(2) :
- f(2) = 3(2)^3 - 7(2)^2 + 2(2) - 5 = 24 - 28 + 4 - 5 = -5
📝 Note: Always evaluate the polynomial at the given value directly to find the remainder.
Exploring the Factor Theorem
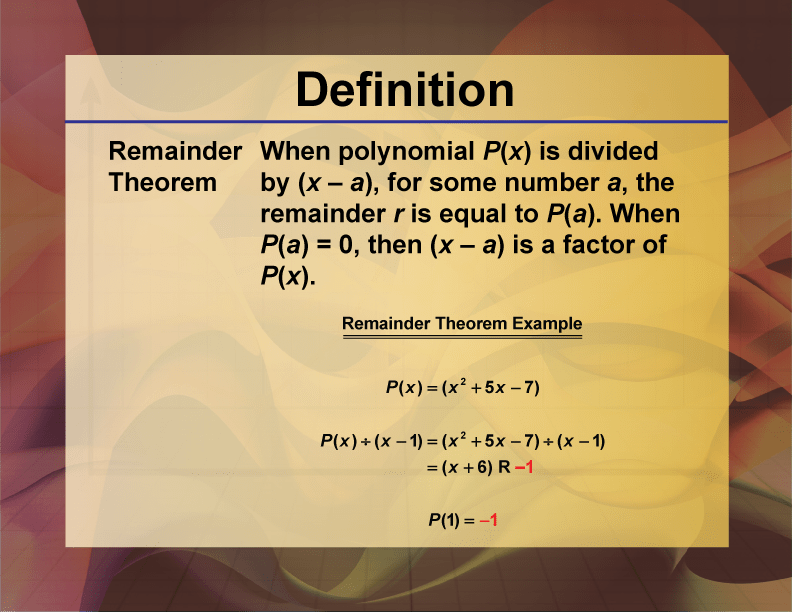
The Factor Theorem is a direct derivative of the Remainder Theorem. It states that if f(c) = 0 , then (x - c) is a factor of f(x) . Here's how it applies:
- Given f(x) = x^3 - 6x^2 + 11x - 6 :
- If f(2) = 0 , then (x - 2) is a factor.
- To verify:
- Calculate f(2) = 8 - 24 + 22 - 6 = 0 . Hence, (x - 2) is indeed a factor.
Worksheet Example

Consider a polynomial g(x) = 2x^4 + 5x^3 - 14x^2 - 19x + 6 . Here are some example problems:
Question 1:

Find the remainder when ( g(x) ) is divided by ( (x - 1) ).
- The remainder ( R(1) = g(1) = 2(1)^4 + 5(1)^3 - 14(1)^2 - 19(1) + 6 = 0 )
- Therefore, the remainder is 0.
Question 2:

Using the Factor Theorem, determine if ( (x - 1) ) is a factor of ( g(x) ).
- Since ( g(1) = 0 ), ( (x - 1) ) is a factor.
Question 3:
What are the factors of ( g(x) ) if ( (x - 1)(x + 2) ) are known factors?
- By synthetic division or synthetic division method:
2 5 -14 -19 6 1 2 7 -7 -26 -2 -4 -2 32 12 2 1 -7 -31 6 
- The last row represents the quotient: ( x^2 + 2 ), thus:
- Since ( x^2 + 2 ) is irreducible, ( g(x) = (x - 1)(x + 2)(x^2 + 2) ).
These steps showcase how the theorems simplify polynomial factorizations and remainder calculations:
📘 Note: Not all factors are of the form (x - c) . Sometimes, synthetic division helps determine higher-degree factors.
Final Thoughts

The Remainder and Factor Theorems offer straightforward ways to determine remainders and factors of polynomials, enhancing problem-solving efficiency. By understanding these theorems, students and algebra enthusiasts can simplify intricate polynomial problems, making what would otherwise be tedious, manageable. It's crucial to not only know how to apply these theorems but also to understand their mathematical foundations for better problem-solving strategies in algebra.
What is the difference between the Remainder Theorem and the Factor Theorem?

+
The Remainder Theorem helps determine the remainder when a polynomial is divided by a linear polynomial. The Factor Theorem, on the other hand, states that if substituting a value into a polynomial results in zero, then that value represents a root, and the corresponding linear term is a factor of the polynomial.
Can the Remainder Theorem be used with complex numbers?

+
Yes, the Remainder Theorem works with complex numbers, but the calculations become more intricate as you need to consider complex division and evaluation.
How do I know if I’ve correctly found all the factors of a polynomial?
+
If the product of all your found factors equals the original polynomial, or if the degree of the remaining polynomial equals the degree of the original minus the number of linear factors you’ve found, then you have likely found all factors.



