5 Essential Tips for Solving Systems of Equations

Solving systems of equations is a fundamental skill in algebra that often appears daunting to many students. Whether you're dealing with linear or non-linear systems, mastering this concept can be crucial not just for academic success, but also for understanding various real-world problems in fields like engineering, economics, and physics. Here are five essential tips to help you tackle systems of equations effectively.
1. Understand the Methods

There are several methods for solving systems of equations:
- Substitution Method: Ideal when one equation has an easily isolable variable.
- Elimination Method: Useful when you can eliminate one variable by adding or subtracting the equations.
- Graphing Method: Graph the equations and look for intersection points.
- Matrix Method: Utilizes matrices to solve systems of equations; especially beneficial for larger systems.
📝 Note: Each method has its advantages, and choosing the right one can simplify the process significantly.
2. Simplify Equations

Before diving into any method, simplify the equations:
- Combine like terms.
- Distribute any parentheses.
- Try to isolate variables if possible.
This step reduces complexity and makes subsequent steps easier to follow.
📘 Note: Simplification can often transform a difficult problem into a manageable one.
3. Visualize the Problem
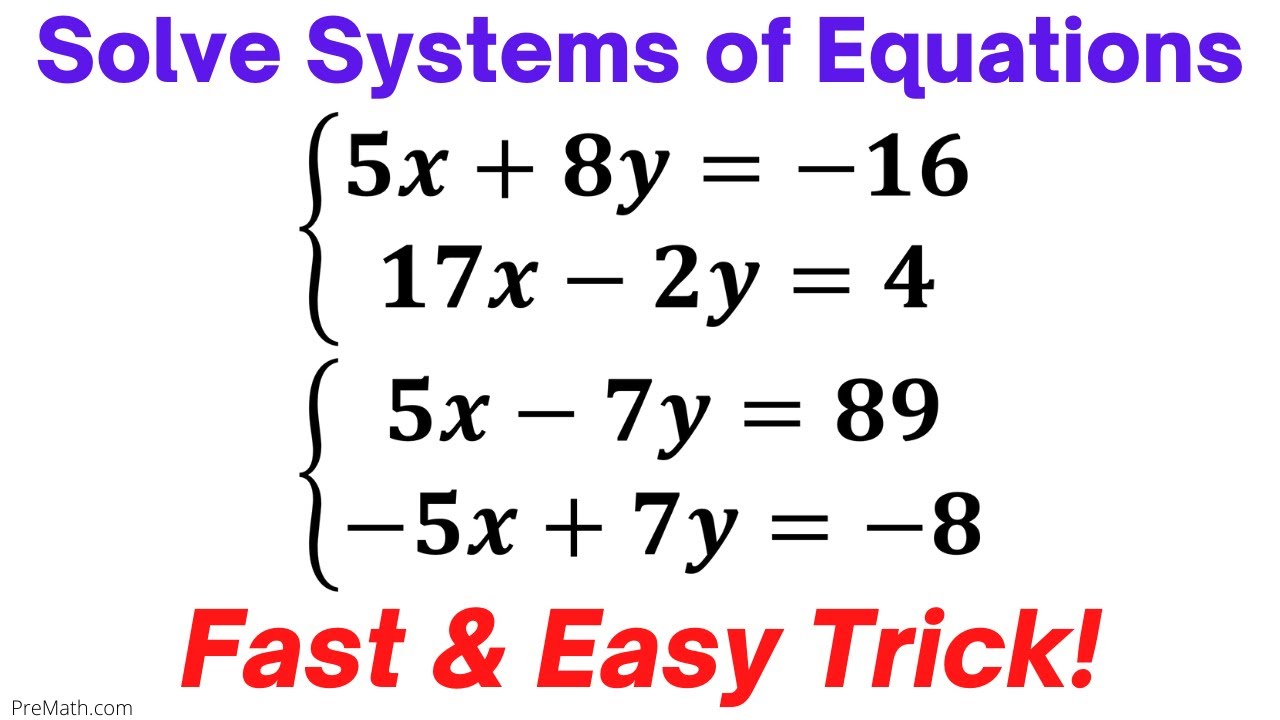
Use graphical representation to:
- Understand the relationship between equations.
- Identify potential solutions or intersections visually.

🌐 Note: Visualization can give you an intuitive feel for how solutions might behave.
4. Leverage Technology

Utilize technology to:
- Check your work using graphing calculators or software.
- Solve complex systems with tools like Wolfram Alpha or MATLAB.
- Simulate or model problems to understand how changes affect the system.
| Tool | Function |
|---|---|
| Graphing Calculator | Graph equations, find intersections |
| Wolfram Alpha | Solve complex systems, step-by-step solutions |
| MATLAB | Mathematical modeling and computation |

🔧 Note: Technology can act as a powerful tool but should not replace understanding the concepts.
5. Practice with Real-World Applications

Connect theory to practice by:
- Solving word problems where systems of equations arise naturally.
- Applying systems of equations to optimize scenarios, like cost or resource allocation.
- Using real-life data to construct and solve systems.
This approach helps in:
- Seeing the relevance of systems of equations.
- Improving problem-solving skills in context.
In mastering the art of solving systems of equations, these tips provide a structured approach that not only enhances your ability to solve such systems but also builds a deeper understanding of how mathematical principles apply in various scenarios. By understanding the methods, simplifying where necessary, visualizing problems, leveraging technology, and practicing with real-world applications, you’ll be better equipped to tackle any set of equations that come your way.
What’s the easiest method to solve a system of equations?

+
The easiest method often depends on the specifics of the equations. Substitution might be simplest if one equation can be quickly solved for one variable, or elimination if adding/subtracting aligns the variables to cancel out.
Why are systems of equations important in real life?

+
Systems of equations model many real-world scenarios where multiple conditions or relationships must be satisfied simultaneously, like in budgeting, network flows, chemical equilibrium, or economic equilibrium.
Can I use only technology to solve all systems of equations?

+
While technology can solve most systems, understanding the manual methods is crucial for conceptual understanding, error checking, and problem-solving in scenarios where technology isn’t available or practical.