Simplify Rational Expressions Worksheet: Complete Answers
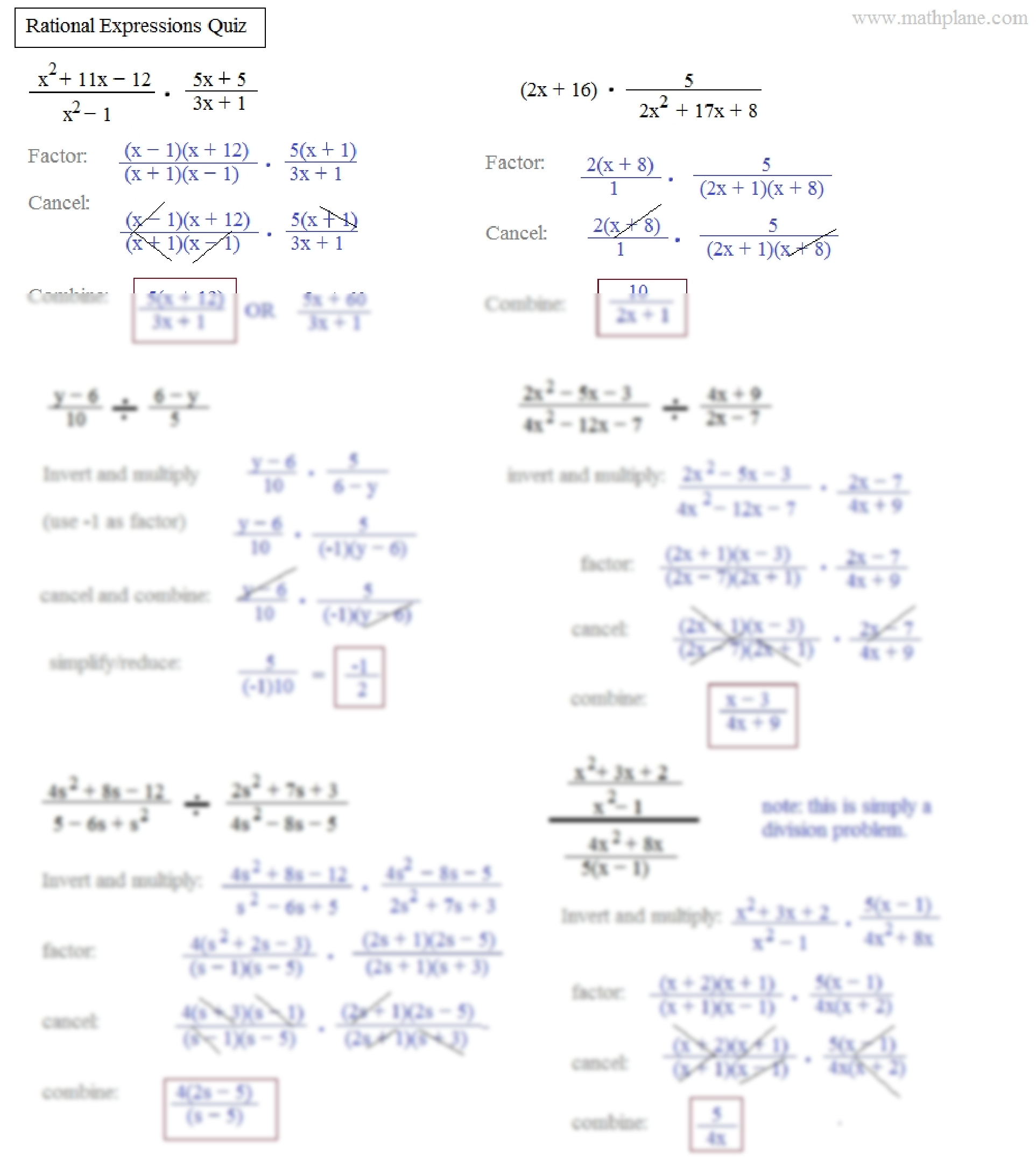
Worksheets are a staple in education for reinforcing learning, and when it comes to mathematics, particularly algebra, a Simplify Rational Expressions Worksheet can be incredibly useful. Rational expressions, fractions involving polynomials, often appear complex, but with practice and understanding, they can be simplified to a form that is more manageable. This blog post dives into the world of rational expressions, providing an in-depth look at how to simplify them effectively.
Understanding Rational Expressions

A rational expression is any expression of the form $\frac{P(x)}{Q(x)}$ where P(x) and Q(x) are polynomials, and Q(x) is not equal to zero. The simplification process involves:
- Factoring the numerator and denominator.
- Canceling out common factors in the numerator and denominator.
- Ensuring that the remaining expression is in its simplest form.
Steps to Simplify Rational Expressions

1. Factorize the Numerator and Denominator

Begin by factoring out the greatest common factor (GCF) from both the numerator and the denominator. This could involve:
- Factoring out constants.
- Breaking down quadratic expressions using methods like difference of squares, grouping, or completing the square.
- Looking for any common binomial factors.
🌟 Note: Always check for common factors first to avoid unnecessary complications in simplification.
2. Cancel Common Factors

Once you’ve factored the expressions, look for common factors in the numerator and denominator:
- Identify any factors that appear in both numerator and denominator.
- Cancel out these common factors, which reduces the complexity of the expression.
3. Check for Any Further Simplification

After canceling common factors, review the expression for any further simplifications:
- Check for possible cancellations of monomials or binomials.
- Ensure no factors were left out during the initial factorization.
4. Handle Excluded Values

Any value that makes the denominator zero is excluded from the domain of the rational expression. When simplifying, ensure these values are accounted for in the final answer:
- List the values that make the simplified denominator zero as conditions for the simplified expression.
Examples of Simplifying Rational Expressions

| Original Expression | Step-by-Step Simplification |
|---|---|
| \frac{x^2 + 4x + 4}{x^2 - 4} |
Numerator: x^2 + 4x + 4 = (x + 2)^2 Denominator: x^2 - 4 = (x - 2)(x + 2) After canceling: \frac{(x + 2)^2}{(x - 2)(x + 2)} = \frac{x + 2}{x - 2} for x ≠ ±2 |
| \frac{2x^3 - 8x^2}{6x^3 - 24x^2 + 24x} |
Numerator: 2x^2(x - 4) Denominator: 6x(x - 4)^2 After canceling: \frac{2x^2(x - 4)}{6x(x - 4)^2} = \frac{2x^2}{6x(x - 4)} = \frac{x}{3(x - 4)} for x ≠ 0, 4 |

Important Notes on Simplification

When dealing with rational expressions, there are a few key points to remember:
- Restricted Values: Always list the values that make the denominator zero after simplifying the expression.
- Factors vs. Terms: Only common factors can be canceled, not terms.
- Check for Higher Degree Polynomials: Higher degree polynomials might have complex roots, requiring advanced factorization techniques.
⚠️ Note: Always ensure that you’ve factored completely to avoid missing any simplifications.
From understanding the basics of rational expressions to applying practical examples, this blog has outlined the steps necessary to simplify these expressions effectively. Remember, practice is the key to mastery, and the inclusion of real-world applications helps reinforce the mathematical concepts covered. We've covered factorization, cancellation, and the importance of considering excluded values in rational expressions. With this knowledge, you're now equipped to tackle any rational expression simplification problem.
Why can’t we cancel terms instead of factors when simplifying rational expressions?

+
Only factors can be canceled because they are being multiplied or divided. Terms, which are added or subtracted, cannot be canceled individually as their presence changes the overall sum or difference.
What do I do if there are no common factors to cancel?

+
If no common factors exist, then the rational expression is already in its simplest form. However, be sure to double-check the factoring to ensure all possible simplifications have been considered.
How do you handle complex polynomials in rational expressions?

+
Complex polynomials might require more advanced techniques like synthetic division, the rational root theorem, or using complex numbers. Familiarity with these methods can be beneficial for higher-level mathematics.
Why is factoring important when simplifying rational expressions?

+
Factoring helps identify common factors that can be canceled, reducing the expression to its simplest form, and uncovering any values that would make the denominator zero, thus excluding them from the domain.


