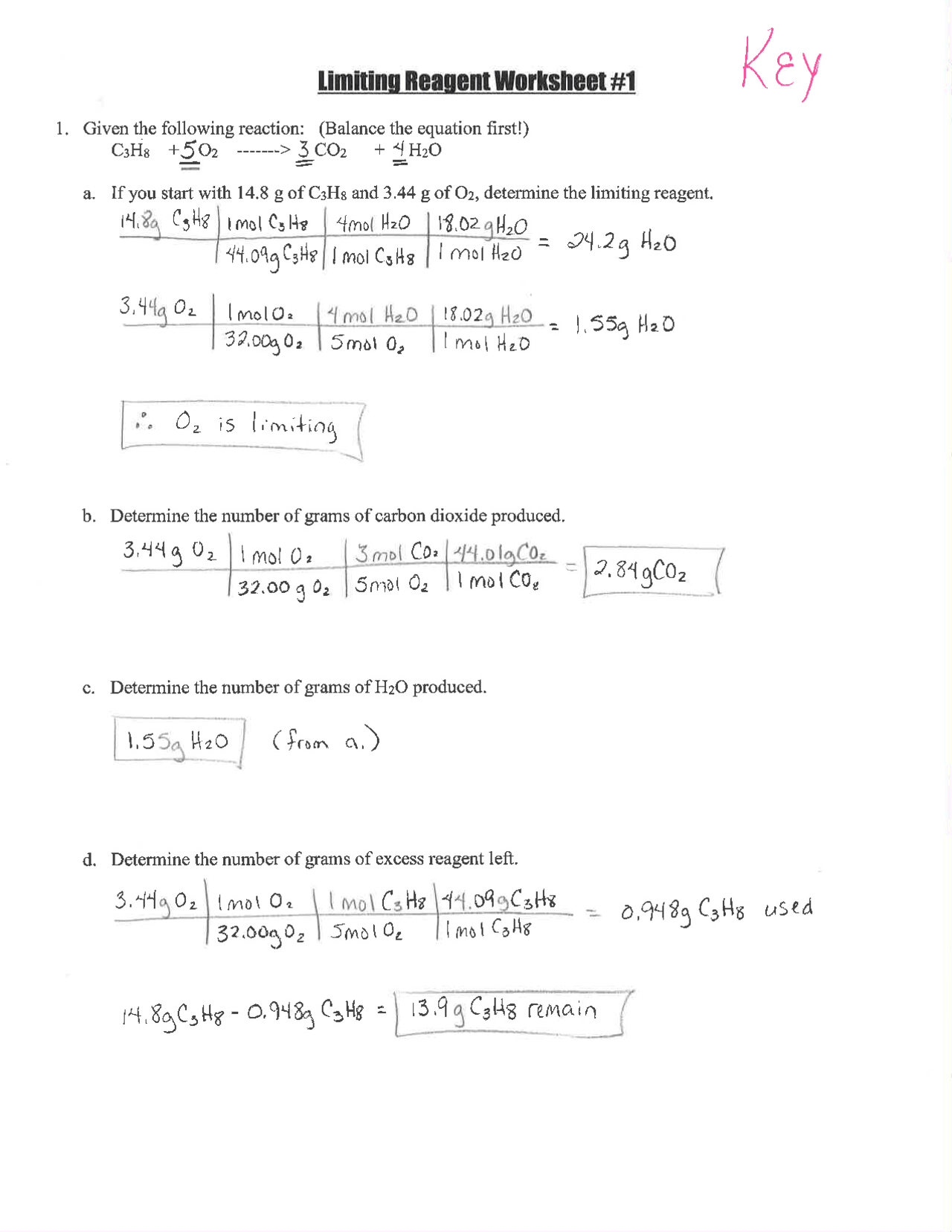
Solve Real-World Problems with Systems of Equations

Understanding Systems of Equations

Systems of equations are a powerful tool for solving real-world problems. A system of equations is a set of two or more equations that have the same variables. These equations can be linear or nonlinear, and they can be solved using a variety of methods.

What is a Linear System of Equations?

A linear system of equations is a set of two or more linear equations that have the same variables. A linear equation is an equation in which the highest power of the variable is 1. For example:

2x + 3y = 7 x - 2y = -3

These two equations form a linear system of equations. The variables are x and y, and the equations are linear because the highest power of the variables is 1.

What is a Nonlinear System of Equations?

A nonlinear system of equations is a set of two or more nonlinear equations that have the same variables. A nonlinear equation is an equation in which the highest power of the variable is greater than 1. For example:

x^2 + y^2 = 25 x - y = 1

These two equations form a nonlinear system of equations. The variables are x and y, and the equations are nonlinear because the highest power of the variables is greater than 1.

Solving Systems of Equations

There are several methods for solving systems of equations, including:

• Substitution Method: This method involves solving one equation for one variable and substituting the result into the other equation.
• Elimination Method: This method involves adding or subtracting the equations to eliminate one variable.
• Graphical Method: This method involves graphing the equations on a coordinate plane and finding the point of intersection.

Solving Linear Systems of Equations

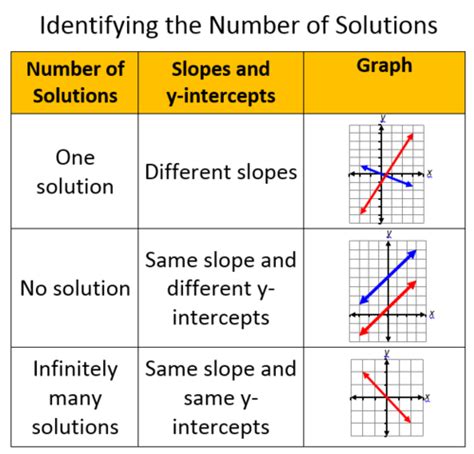
Linear systems of equations can be solved using the substitution method or the elimination method.

Example 1: Substitution Method

Solve the system of equations:

x + 2y = 7 3x - 2y = -1

Solution:

Solve the first equation for x:

x = 7 - 2y

Substitute the result into the second equation:

3(7 - 2y) - 2y = -1

Expand and simplify:

21 - 6y - 2y = -1

Combine like terms:

-8y = -22

Divide by -8:

y = ¹¹⁄₄

Now substitute the value of y back into one of the original equations to find x:

x + 2(¹¹⁄₄) = 7

Simplify:

x + ²²⁄₄ = 7

x = 7 - ²²⁄₄

x = ⁶⁄₄

x = ³⁄₂

Therefore, the solution is x = ³⁄₂ and y = ¹¹⁄₄.

Example 2: Elimination Method

Solve the system of equations:

2x + 3y = 7 x - 2y = -3

Solution:

Add the two equations to eliminate x:

(2x + 3y) + (x - 2y) = 7 + (-3)

Combine like terms:

3x + y = 4

Now subtract the second equation from the first equation to eliminate x:

(2x + 3y) - (x - 2y) = 7 - (-3)

Combine like terms:

x + 5y = 10

Now we have two new equations:

x + 5y = 10 3x + y = 4

We can solve these equations using substitution or elimination.

Solving Nonlinear Systems of Equations

Nonlinear systems of equations can be solved using the substitution method or the graphical method.

Example 3: Substitution Method

Solve the system of equations:

x^2 + y^2 = 25 x - y = 1

Solution:

Solve the second equation for x:

x = y + 1

Substitute the result into the first equation:

(y + 1)^2 + y^2 = 25

Expand and simplify:

y^2 + 2y + 1 + y^2 = 25

Combine like terms:

2y^2 + 2y - 24 = 0

Divide by 2:

y^2 + y - 12 = 0

Factor:

(y + 4)(y - 3) = 0

Solve for y:

y + 4 = 0 or y - 3 = 0

y = -4 or y = 3

Now substitute the values of y back into one of the original equations to find x:

x - (-4) = 1 or x - 3 = 1

Simplify:

x + 4 = 1 or x = 4

x = -3 or x = 4

Therefore, the solutions are x = -3 and y = -4, or x = 4 and y = 3.

Applications of Systems of Equations

Systems of equations have many real-world applications, including:

• Physics and Engineering: Systems of equations are used to model the motion of objects, including the trajectory of projectiles and the vibration of springs.
• Economics: Systems of equations are used to model the behavior of economic systems, including the supply and demand of goods and services.
• Computer Science: Systems of equations are used to model the behavior of complex systems, including the flow of traffic and the spread of diseases.

Example 4: Physics Application

A projectile is launched from the ground with an initial velocity of 20 m/s at an angle of 30 degrees above the horizontal. The acceleration due to gravity is 9.8 m/s^2. Find the maximum height reached by the projectile.

Solution:

Let x be the horizontal distance traveled by the projectile and y be the vertical distance. We can model the motion of the projectile using the following system of equations:

x = 20cos(30)t y = 20sin(30)t - (¹⁄₂)9.8t^2

Solve the system of equations to find the maximum height reached by the projectile.

Conclusion

Systems of equations are a powerful tool for solving real-world problems. They can be used to model a wide range of phenomena, from the motion of objects to the behavior of economic systems. By using substitution, elimination, or graphical methods, we can solve systems of equations and gain insights into the world around us.

What is a system of equations?

+

A system of equations is a set of two or more equations that have the same variables.

What is the difference between a linear and nonlinear system of equations?

+

A linear system of equations is a set of linear equations, while a nonlinear system of equations is a set of nonlinear equations.

What are some methods for solving systems of equations?

+

Some methods for solving systems of equations include substitution, elimination, and graphical methods.

Related Terms:

• Systems of Equations Applications Worksheet
• solving systems word problems worksheet
• system of equations pdf worksheet
• solving linear equations application problems
• solving systems of equations applications
• systems of equations practice worksheet