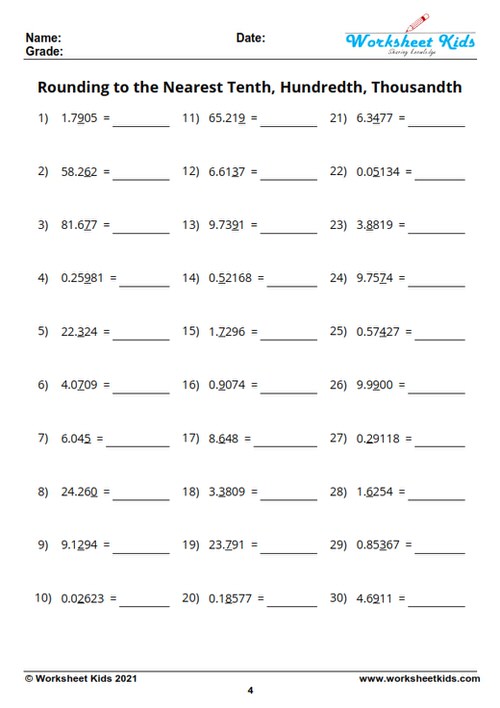
5 Stoichiometry Problems Solved Easily

The realm of stoichiometry often seems daunting at first, but with the right understanding, solving problems in this field can become straightforward and almost intuitive. Here, we'll dive into five stoichiometry problems, explaining the thought process and step-by-step solutions, making what is considered complex into something simple and engaging.

Problem 1: The Classic Iron-Sulfur Reaction

Let’s start with an easy one. Imagine we have:

• 45.0 grams of iron (Fe)
• An excess amount of sulfur (S₈)

We want to know how many grams of iron(II) sulfide (FeS) we can produce.

The balanced equation is:

8Fe + S8 → 8FeS

Solving:

1. Convert the given mass of iron to moles: \[ \text{Moles of Fe} = \frac{45.0 \text{ g}}{55.845 \text{ g/mol}} \approx 0.806 \text{ mol} \]
2. Use the stoichiometry from the balanced equation to find the moles of FeS produced: \[ \text{Moles of FeS} = 0.806 \text{ mol Fe} \times \frac{8 \text{ mol FeS}}{8 \text{ mol Fe}} = 0.806 \text{ mol FeS} \]
3. Convert moles of FeS back to grams: \[ \text{Grams of FeS} = 0.806 \text{ mol} \times 87.91 \text{ g/mol} \approx 70.89 \text{ g} \]

So, 70.89 grams of iron(II) sulfide can be produced from 45.0 grams of iron with an excess of sulfur.

🌟 Note: Remember that the conversion between moles and grams always requires molar mass. Always keep an eye on the stoichiometric coefficients in the balanced equation to determine the ratio of reactants to products.

Problem 2: Limiting Reactant in Combustion

Now let’s tackle the scenario where the quantities of both reactants are given:

• 12.0 grams of methane (CH₄)
• 32.0 grams of oxygen (O₂)

We want to determine:

• Which reactant is limiting?
• How many moles of water (H₂O) will be produced?

The balanced combustion reaction is:

CH4 + 2O2 → CO2 + 2H2O

Solving:

1. Convert mass to moles for both reactants: \[ \text{Moles of CH}_4 = \frac{12.0 \text{ g}}{16.04 \text{ g/mol}} \approx 0.748 \text{ mol} \] \[ \text{Moles of O}_2 = \frac{32.0 \text{ g}}{32.00 \text{ g/mol}} = 1 \text{ mol} \]
2. Determine the limiting reactant by comparing the mole ratios: \[ \text{Moles of CH}_4 \text{ reacted with O}_2 = 0.748 \text{ mol} \times \frac{2 \text{ mol O}_2}{1 \text{ mol CH}_4} = 1.496 \text{ mol O}_2 \] Since we only have 1 mole of O₂, O₂ is the limiting reactant.
3. Calculate the moles of water produced: \[ \text{Moles of H}_2\text{O} = 1 \text{ mol O}_2 \times \frac{2 \text{ mol H}_2\text{O}}{2 \text{ mol O}_2} = 1 \text{ mol H}_2\text{O} \]

So, the limiting reactant is oxygen, and 1 mole of water will be produced.

⚠️ Note: When dealing with limiting reactants, you must determine which reactant is in excess, as this will influence the amount of product formed.

Problem 3: Aqueous Solutions and Molarity

Here we’ll work with concentrations in aqueous solutions:

• 25 mL of 0.100 M hydrochloric acid (HCl) is added to 100 mL of 0.050 M barium hydroxide (Ba(OH)₂)
• Determine how many moles of barium chloride (BaCl₂) will form and the final concentration of the resulting solution.

The balanced reaction is:

2HCl + Ba(OH)2 → BaCl2 + 2H2O

Solving:

1. Calculate the moles of HCl and Ba(OH)₂: \[ \text{Moles of HCl} = 0.100 \text{ M} \times 0.025 \text{ L} = 0.0025 \text{ mol} \] \[ \text{Moles of Ba(OH)}_2 = 0.050 \text{ M} \times 0.100 \text{ L} = 0.0050 \text{ mol} \]
2. Find the limiting reactant: \[ \text{Moles of HCl} = 0.0025 \text{ mol} \times \frac{1 \text{ mol Ba(OH)}_2}{2 \text{ mol HCl}} = 0.00125 \text{ mol Ba(OH)}_2 \] Since we have 0.0050 moles of Ba(OH)₂, HCl is the limiting reactant.
3. Determine moles of BaCl₂ produced: \[ \text{Moles of BaCl}_2 = 0.0025 \text{ mol HCl} \times \frac{1 \text{ mol BaCl}_2}{2 \text{ mol HCl}} = 0.00125 \text{ mol BaCl}_2 \]
4. Calculate the final volume of the solution: \[ V_{total} = 25 \text{ mL} + 100 \text{ mL} = 125 \text{ mL} = 0.125 \text{ L} \]
5. Find the final concentration of BaCl₂: \[ C_{BaCl_2} = \frac{0.00125 \text{ mol}}{0.125 \text{ L}} = 0.01 \text{ M} \]

The 0.00125 moles of barium chloride will form, with the final concentration being 0.01 M.

Problem 4: Ideal Gas Law Calculation

This problem involves gases:

• Determine the volume of hydrogen gas (H₂) produced at STP (Standard Temperature and Pressure) from the reaction of 5.67 grams of sodium with hydrochloric acid.

The reaction is:

2Na + 2HCl → 2NaCl + H2

Solving:

1. Convert grams of sodium to moles: \[ \text{Moles of Na} = \frac{5.67 \text{ g}}{23.0 \text{ g/mol}} \approx 0.247 \text{ mol} \]
2. From the balanced equation, 2 moles of Na produce 1 mole of H₂, so: \[ \text{Moles of H}_2 = 0.247 \text{ mol Na} \times \frac{1 \text{ mol H}_2}{2 \text{ mol Na}} \approx 0.124 \text{ mol} \]
3. Using the ideal gas law at STP (where 1 mole of gas occupies 22.4 L): \[ V_{H_2} = 0.124 \text{ mol} \times 22.4 \text{ L/mol} \approx 2.77 \text{ L} \]

So, 2.77 liters of hydrogen gas will be produced.

🔬 Note: Always consider conditions like temperature and pressure when dealing with gases, as they can significantly impact the volume occupied by the gas.

Problem 5: Stoichiometry with Kinetics

This problem involves combining stoichiometry with chemical kinetics:

• Given the following rate law for the decomposition of N₂O₅: Rate = k[N₂O₅], where k = 5.6 × 10^-4 s^-1, how much N₂O₅ remains after 600 seconds, if you start with 3.0 mol of N₂O₅?

The balanced reaction is:

2N2O5 → 4NO2 + O2

Solving:

1. Use the integrated rate law for a first-order reaction: \[ \text{ln}[N_2O_5]_t - \text{ln}[N_2O_5]_0 = -kt \] Where, [N₂O₅]_t is the concentration at time t, [N₂O₅]₀ is the initial concentration, k is the rate constant, and t is time.
2. Solve for [N₂O₅]_t: \[ \text{ln}[N_2O_5]_t = \text{ln}3.0 - (5.6 \times 10^{-4} \text{ s}^{-1}) \times 600 \text{ s} \] \[ \text{ln}[N_2O_5]_t \approx \text{ln}3.0 - 0.336 \] \[ \text{ln}[N_2O_5]_t \approx 1.099 - 0.336 \] \[ [N_2O_5]_t \approx e^{0.763} \approx 2.14 \text{ mol} \]

Thus, 2.14 moles of N₂O₅ will remain after 600 seconds.

🧪 Note: Integrating rate laws allows us to understand how reactant concentrations change over time, which is crucial for predicting outcomes in chemical reactions.

In this exploration of stoichiometry, we’ve simplified complex reactions and calculations into manageable steps. Whether it’s determining limiting reactants, calculating gas volumes, or combining stoichiometry with kinetics, the key is understanding the relationships between reactants and products through balanced chemical equations and utilizing fundamental concepts of chemistry. This foundational knowledge not only helps in solving problems but also in appreciating the beauty and precision of chemical reactions. Here’s to a deeper understanding and mastery of stoichiometry.

What is stoichiometry?

+

Stoichiometry is the branch of chemistry that deals with the quantitative relationships between reactants and products in chemical reactions. It involves using balanced chemical equations to calculate the amount of reactants consumed or products formed.

How do you determine the limiting reactant in a reaction?

+

Determine the limiting reactant by comparing the moles of each reactant available with the moles required by the stoichiometry of the balanced equation. The reactant that runs out first based on these calculations is the limiting reactant.

Why is the molarity concept important in aqueous solutions?

+

Molarity is important because it allows chemists to express the concentration of solutes in solution, which is critical for performing calculations involving reactions in solution, determining reaction rates, and understanding osmotic pressure.