Mastering Systems of Equations Word Problems: Effective Worksheets

When students first encounter systems of equations, they often view them as an abstract and challenging concept. However, by transforming these equations into word problems, learning becomes more tangible and relatable. This blog post will delve into why word problems are beneficial for mastering systems of equations, how to effectively design and utilize worksheets for this purpose, and share techniques that can make your students excel in algebraic word problems.
Why Word Problems are Crucial for Learning Systems of Equations
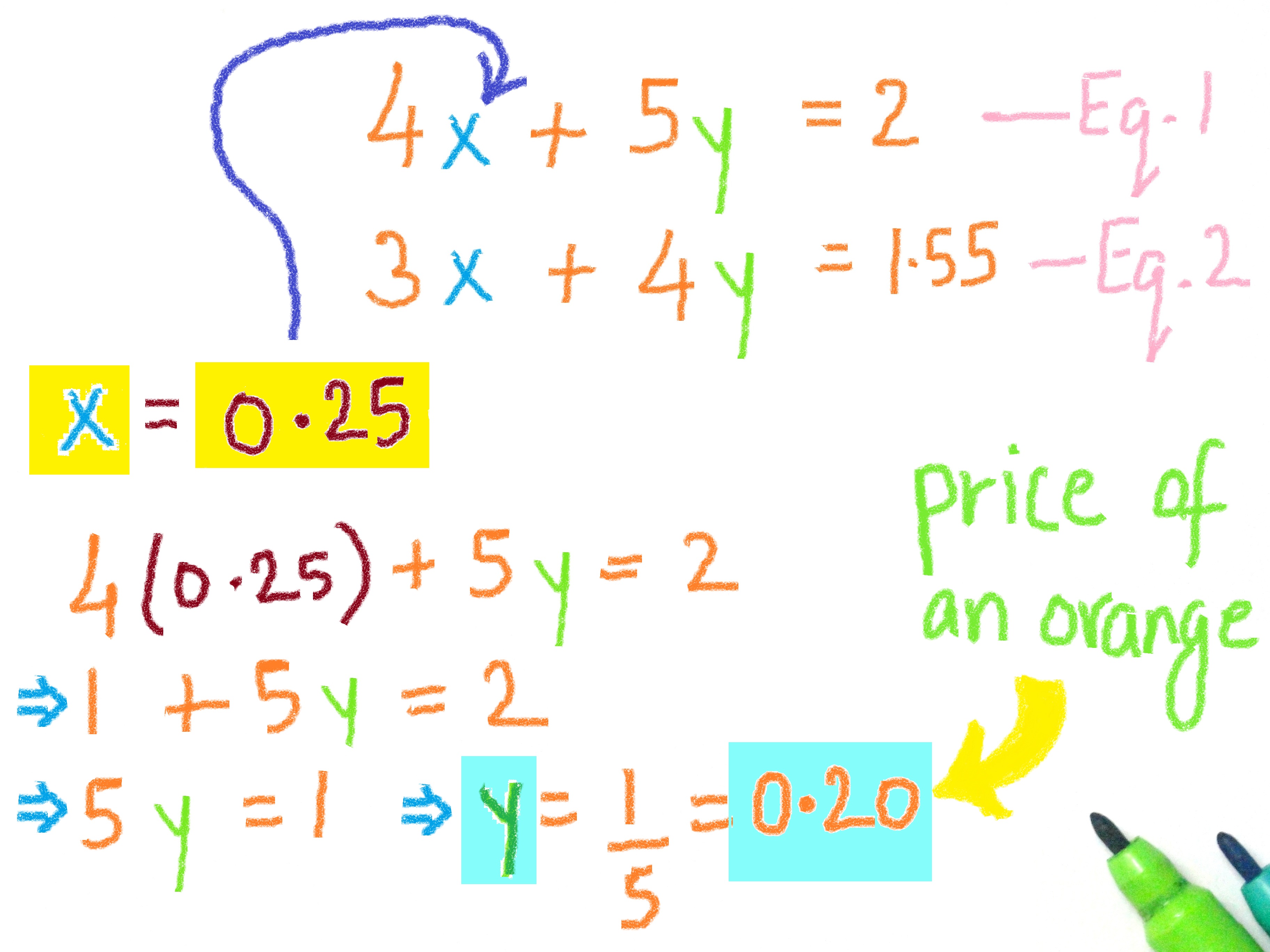

Word problems turn abstract algebra into practical, real-world scenarios. Here are several reasons why integrating systems of equations into word problems enhances learning:
- Contextual Learning - By connecting math to real-life scenarios, students can see the relevance of algebra in daily life, which improves comprehension and retention.
- Problem Solving Skills - Word problems demand not only algebraic skills but also critical thinking and the ability to formulate and solve problems.
- Developing Mathematical Communication - Students learn to translate from verbal descriptions to equations, an essential skill in mathematics.
Designing Effective Worksheets for Systems of Equations Word Problems


To create a worksheet that truly benefits students, consider the following guidelines:
- Clarity in Language - Ensure the word problem is stated clearly, avoiding confusing terms or overly complex language.
- Scaffolded Problems - Gradually increase problem complexity to build confidence and understanding step by step.
- Visual Aids - Use diagrams, charts, or pictures that can help visualize the scenario described in the problem.
| Complexity Level | Example Problem |
|---|---|
| Beginner | Mary has 12 and John has 5. Together they save $17. How much do they have together? |
| Intermediate | Two trains are traveling in opposite directions. Train A is 30 km/h faster than train B. After 2 hours, they are 180 km apart. What are their speeds? |
| Advanced | A boat can travel 60 miles downstream in the same time it takes to travel 40 miles upstream. The current’s speed is 5 mph. What is the boat’s speed in still water? |

Step-by-Step Techniques to Solve Word Problems

Here’s how you can guide students through solving word problems involving systems of equations:
- Understand the Problem - Read the problem carefully. Identify the knowns and unknowns. What are we solving for?
- Set Up Equations - Translate the problem into two or more equations. Each equation should represent one aspect of the problem.
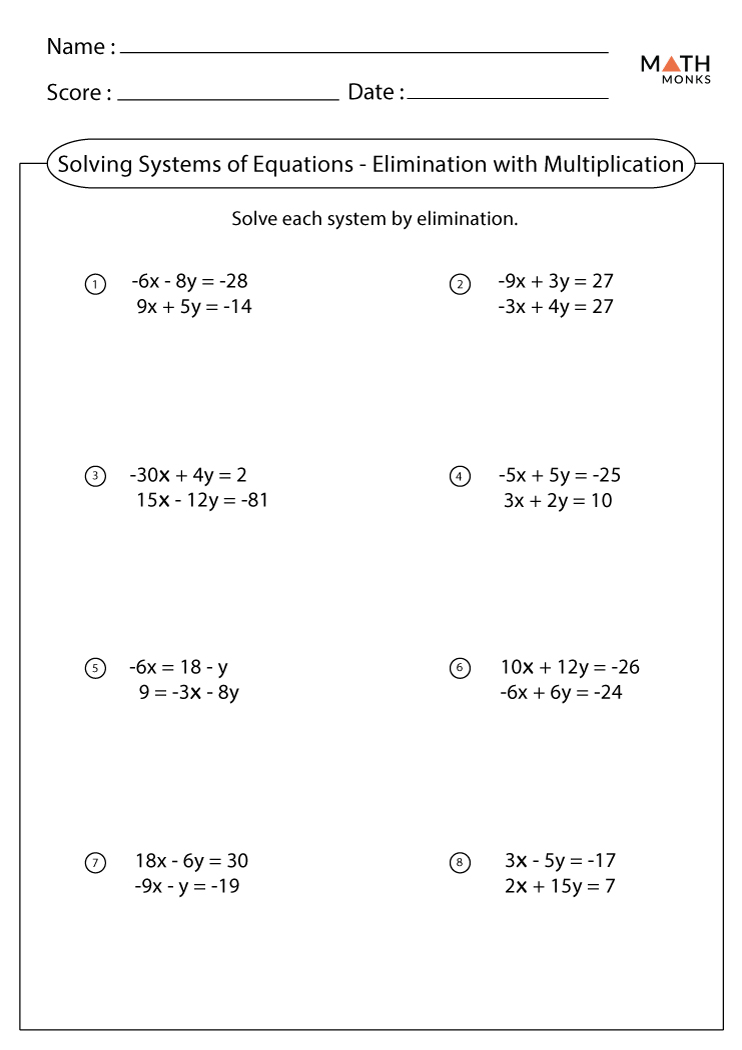
- Solve the System - Use substitution, elimination, or graphing to solve the equations.
- Check Your Work - Verify your solution by substituting the answers back into the original problem to ensure logical coherence.
⚠️ Note: Always check that your solution makes sense within the context of the problem. A mathematical answer might not always be a practical one.
Incorporating Real-Life Applications in Worksheets


To deepen understanding, you can include problems related to:
- Business - Cost and profit calculations.
- Economics - Supply and demand.
- Physics - Motion problems with multiple moving objects.
Assessing Student Progress

Progress evaluation is crucial to ensure learning. Here are some methods to assess students:
- Worksheet Evaluation - Look for accuracy, logical flow, and correct equation setup.
- Group Work and Discussion - Encourage students to solve problems together, promoting peer teaching.
- Quizzes and Tests - Use various problem types to gauge understanding at different complexity levels.
Common Challenges Students Face and How to Overcome Them

Understanding and overcoming common hurdles in solving systems of equations word problems is key:
- Translating Words into Equations - Provide explicit examples and practice exercises where students must convert phrases into mathematical statements.
- Solving Complex Systems - Use step-by-step guided solutions to illustrate the problem-solving process.
- Interpreting Results - Train students to understand what their results mean in the context of the problem.
Final Thoughts

Mastering systems of equations through word problems isn’t just about solving equations; it’s about making algebra meaningful and practical. By using well-designed worksheets, fostering a step-by-step problem-solving approach, and integrating real-life applications, students can not only solve equations but also apply their knowledge effectively in various contexts. Emphasize the importance of clarity, context, and logical thinking, and your students will be well on their way to algebraic proficiency.
Why do we use word problems to teach systems of equations?

+
Word problems provide context to the equations, making them more relatable and understandable. This contextual learning aids in retention and application of algebraic concepts.
What strategies can help students solve word problems involving systems of equations?

+
Here are some strategies:
- Carefully read and understand the problem.
- Translate the problem into algebraic equations.
- Use substitution, elimination, or graphing to solve the system.
- Check solutions for logical consistency.
How can I make word problem worksheets engaging for students?
+
Consider these ideas:
- Use visuals and diagrams to aid understanding.
- Incorporate problems based on student interests or current events.
- Challenge students with different levels of complexity.
- Encourage collaborative problem solving.
What are the signs that a student is struggling with systems of equations word problems?

+
Look for:
- Difficulty translating word problems into equations.
- Struggles in setting up a system of equations.
- Inconsistencies or inaccuracies in solving the system.
- Inability to verify or interpret results logically.
How can worksheets help in mastering systems of equations?

+
Worksheets provide:
- Structured practice for repetition.
- Opportunities for progressive learning from simple to complex.
- Immediate feedback when designed with answer keys or discussion prompts.