3 Essential Ways to Solve Quadratic Equations by Factoring

Exploring the methods to solve quadratic equations by factoring can be a valuable tool in mathematics. Whether you're a student in high school or tackling more complex algebraic problems, mastering these techniques can significantly simplify your math work.
Understanding Quadratic Equations

A quadratic equation is an equation of the form ax2 + bx + c = 0, where a, b, and c are constants with a ≠ 0. The goal is to find the value of x which makes the equation true. Here, we’ll focus on the method of factoring.
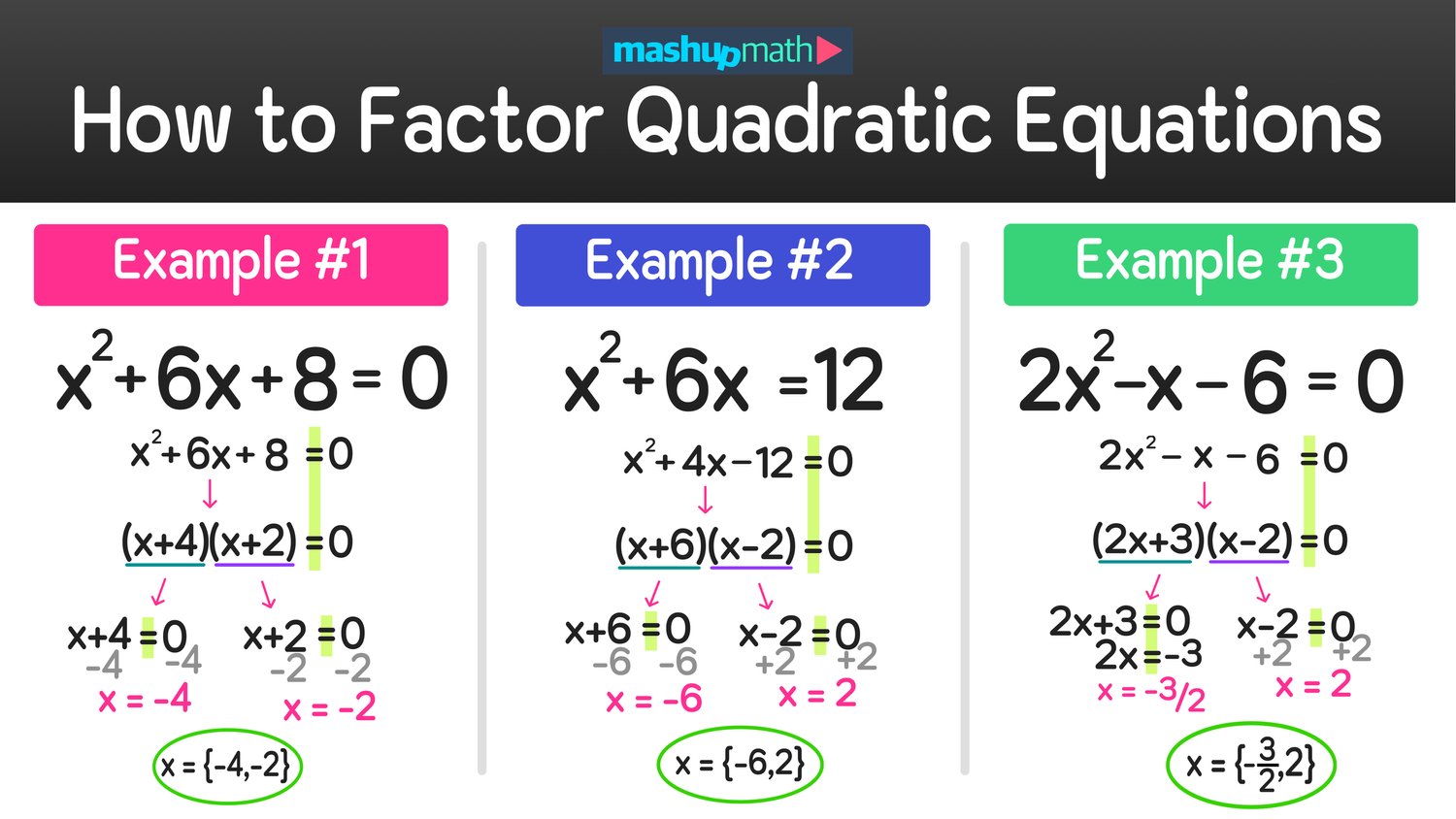
Method 1: Factoring with Integer Roots

When the quadratic equation has two real roots that are integers, factoring can be particularly straightforward:
- Identify the product and sum of the roots.
- Set up two binomials with these roots.
- Multiply these binomials to check your work.
Here’s an example:
x2 - 5x + 6 = 0
- The product of the roots = 6, sum = -5.
- The roots are -2 and -3 since (-2)(-3)=6 and (-2)+(-3)=-5.
- The equation can be factored as (x - 2)(x - 3) = 0.
📝 Note: Always verify your solutions by substituting them back into the original equation.
Method 2: Completing the Square

If the roots aren’t integers, you might resort to completing the square:
- Move the constant term c to the other side of the equation.
- Add and subtract the square of half the coefficient of x to complete the square.
- Take the square root of both sides.
Consider:
x2 + 8x + 10 = 0
- Move the constant to the right: x2 + 8x = -10.
- Complete the square: x2 + 8x + 16 = -10 + 16.
- Now, you have (x + 4)2 = 6.
- Take the square root: x + 4 = ±√6.
With solutions x = -4 ± √6.
🧠 Note: Completing the square is especially useful when dealing with quadratic equations in vertex form or those with irrational roots.
Method 3: Using the Quadratic Formula

For general quadratic equations, the quadratic formula is the most reliable approach:
- Plug a, b, and c into the formula:
x = [-b ± √(b2 - 4ac)] / (2a)
For example:
2x2 + 5x - 3 = 0
- a = 2, b = 5, c = -3
- Substitute these into the formula.
- Get x = [-5 ± √(25 + 24)] / 4.
- Simplify to x = [-5 ± √49] / 4, hence x = (-5 ± 7) / 4.
- The solutions are x = 1⁄2 and x = -3.
This method works for all quadratic equations, whether the roots are real or complex.
Summing Up

In this post, we’ve discussed three primary ways to solve quadratic equations through factoring. Understanding how to apply integer root factoring, completing the square, and using the quadratic formula equips you with versatile tools for problem-solving in algebra. These methods, each with its unique application, help in navigating the landscape of quadratic equations more efficiently. By consistently practicing these techniques, you’ll enhance your mathematical intuition and problem-solving skills.
Can I use factoring if the roots are not integers?

+
Yes, but you might need to use more advanced techniques like completing the square or the quadratic formula when the roots are not integers.
What should I do if the discriminant (b2-4ac) is negative?
+
The equation then has complex roots. You can still solve it using the quadratic formula, and the solutions will involve imaginary numbers.
How can I tell which method to use for solving a quadratic equation?

+
It often depends on the form of the equation and your comfort with the techniques. Factoring is generally preferred for simple cases, while the quadratic formula works universally.



