Algebra 1 Made Easy: Simplify Expressions Worksheet

Algebra 1 can often feel like a daunting mountain to climb, with variables, expressions, and equations stretching in every direction. However, understanding how to simplify expressions is one of the foundational skills that will pave the way for mastering more complex algebraic concepts. Today, we're going to demystify this process, walking through step-by-step examples to ensure you feel confident in your algebra skills.
Understanding the Basics of Simplifying Expressions

Before diving into the techniques, let’s understand what we mean by simplifying an expression. Simplifying an algebraic expression means reducing it to its most basic form without changing the value it represents. Here are the core elements to focus on:
- Combine Like Terms: Terms that contain the same variables raised to the same power can be combined.
- Distribute: Use the distributive property to remove parentheses.
- Factor: Factor out common factors to simplify.
Step-by-Step Guide to Simplify Expressions

Let’s go through the process of simplifying an expression with an example.
Example 1: Simplifying a Polynomial Expression

Consider the expression:
3x + 5 + 7x - 2
Step 1: Combine Like Terms
First, identify the like terms:
- 3x and 7x are like terms because they both contain x raised to the first power.
- 5 and -2 are also like terms since they are both constants.
The expression now becomes:
(3x + 7x) + (5 - 2)
Combine the coefficients:
10x + 3
Now let's look at a more complex example:
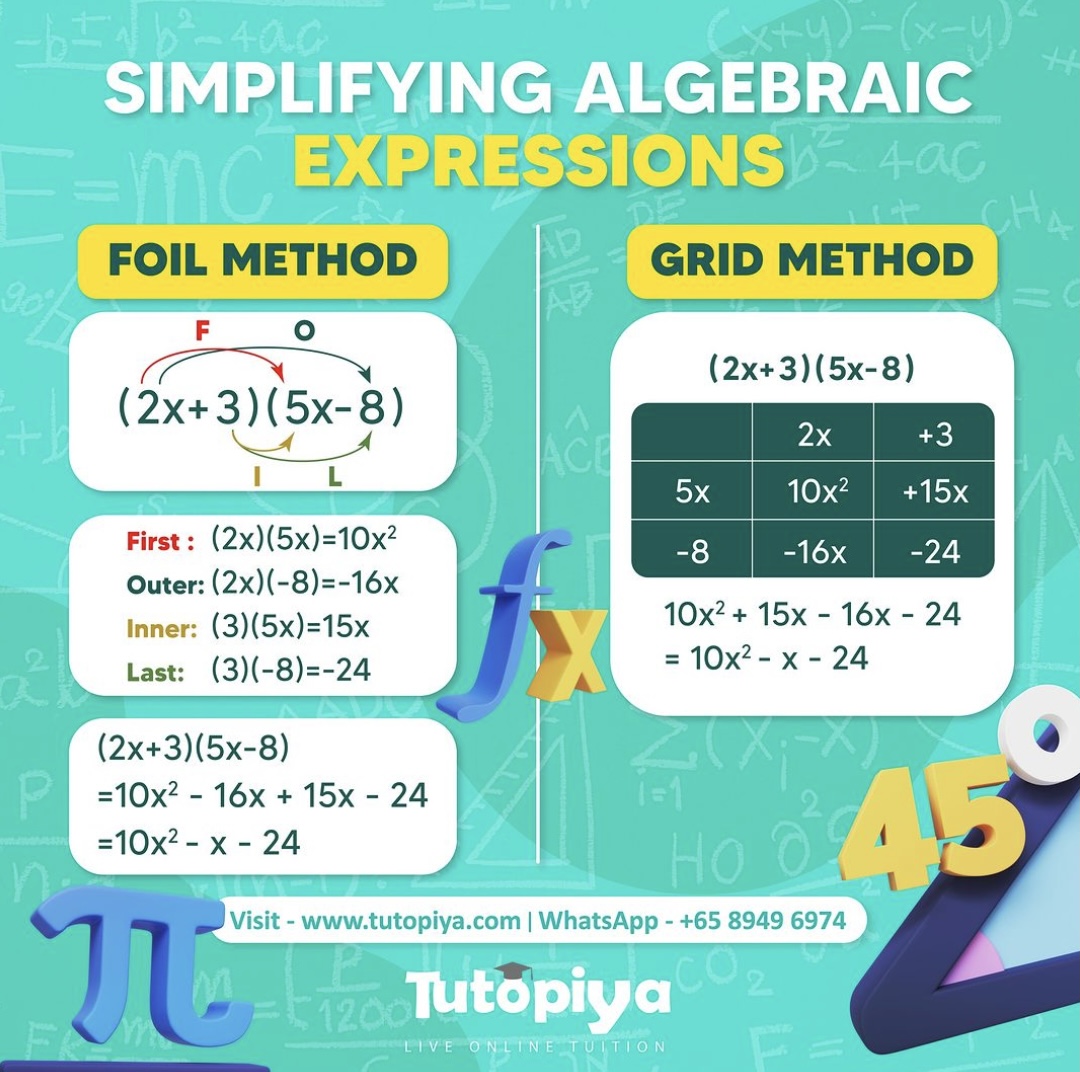
Example 2: Using the Distributive Property

Consider the expression:
2(3x - 4) + 5x
Step 1: Distribute the Coefficient
Use the distributive property to expand:
- 2(3x) - 2(4) gives us 6x - 8.
Step 2: Combine Like Terms
Now, add the term 5x:
6x + 5x - 8 which simplifies to 11x - 8.
Example 3: Factoring to Simplify

Consider the expression:
10x + 15 - 5x - 12
Step 1: Combine Like Terms
Combine the x terms and the constants:
(10x - 5x) + (15 - 12) which simplifies to 5x + 3.
Step 2: Factor Common Factors
Factor out the common factor of 5:
5(x + 1)
Here's a summary table of what we've learned:
| Step | Description |
|---|---|
| Combine Like Terms | Add or subtract coefficients of like terms. |
| Distribute | Multiply terms within parentheses by the external coefficient or variable. |
| Factor | Pull out common factors to simplify the expression. |
🔥 Note: Always check your work by plugging in a value for x if possible. This can confirm the expression has been simplified correctly.
Mastering these techniques is key to simplifying expressions in Algebra 1. Not only does it make problem-solving easier, but it also improves your ability to understand and manipulate equations, which is vital for further studies in algebra and beyond.
Why is it important to simplify algebraic expressions?

+
Simplifying expressions helps in understanding the underlying structure of equations and makes solving problems more manageable. It also reduces the chances of errors when working with complex equations.
What if my expression has variables with different exponents?
+
Terms with different exponents aren’t like terms and can’t be combined. You can only combine terms where both the variable and the exponent are the same.
Can simplifying change the value of an expression?

+
No, simplifying expressions does not change their value, only their appearance. It’s akin to rearranging furniture in a room without altering the room itself.