Simple Harmonic Motion Worksheet Answers: Complete Guide

The exploration of Simple Harmonic Motion (SHM) is fundamental in physics, providing insights into the oscillatory behavior that appears in various natural phenomena, from the motion of a mass on a spring to the behavior of atoms. Here's a comprehensive guide with worksheet answers that will help deepen your understanding of SHM.
Understanding Simple Harmonic Motion

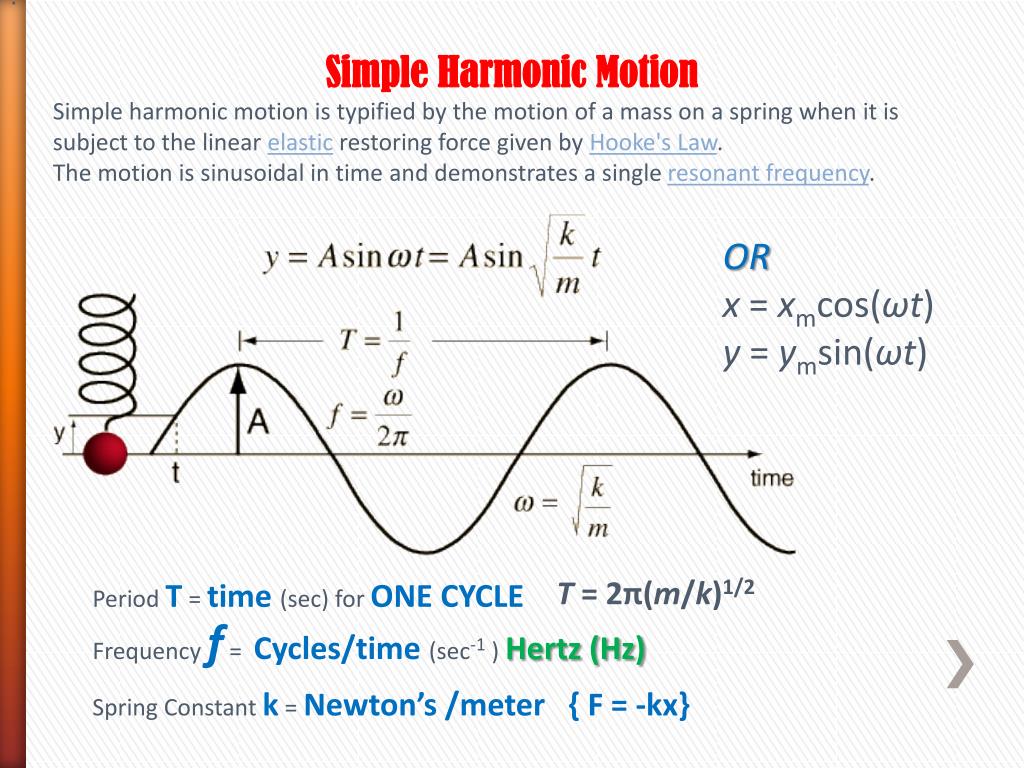
Simple Harmonic Motion occurs when an object oscillates back and forth around an equilibrium position due to a restoring force that's proportional to its displacement from equilibrium. This motion is periodic, and its properties can be expressed mathematically:
- Displacement (x): Measures how far the object is from its equilibrium position.
- Amplitude (A): The maximum displacement from equilibrium.
- Angular Frequency (ω): Determines how fast the object oscillates; in radians per second.
- Period (T): Time taken for one complete oscillation.
- Frequency (f): How many oscillations occur in a second.
The relationship between these quantities can be summarized with the equations:
ω = 2πf and T = 1/f
Worksheet Question 1

Question: A mass is attached to a spring with a spring constant of 80 N/m. If the mass moves through a distance of 0.30 m, what is the amplitude of its motion?
Answer: The amplitude (A) is simply the maximum displacement from the equilibrium position. Hence, the amplitude here is:
A = 0.30 m
Worksheet Question 2

Question: If the angular frequency of this oscillator is found to be 8 rad/s, what is the mass of the object?
Answer: For a mass-spring system in SHM, the angular frequency (ω) is given by:
ω = √(k/m)
Where k is the spring constant and m is the mass. Rearranging to solve for m:
m = k/ω² = 80/64 = 1.25 kg
🔍 Note: Units are crucial. Ensure that the spring constant (N/m) and the angular frequency (rad/s) units are consistent when solving for mass.
Worksheet Question 3

Question: What is the period of oscillation for the above mass-spring system?
Answer: Using the relation T = 2π/ω:
T = 2π/8 ≈ 0.785 s
Worksheet Question 4

Question: If the mass in the previous example were reduced to half, how would this affect the period?
Answer: Since the period T is inversely proportional to the square root of the mass (T ∝ 1/√m), reducing the mass by half would:
T_new = √(m/2) * T_old = 0.5625 * T_old ≈ 0.442 s
Worksheet Question 5

Question: Consider a simple pendulum with a length of 0.6 m. What will be its period of oscillation on Earth (g ≈ 9.8 m/s²)?
Answer: For small oscillations, the period of a simple pendulum is given by:
T = 2π √(L/g)
Substituting the values:
T ≈ 1.56 s
🔍 Note: This equation assumes small angles of oscillation (θ ≤ 15°) where the amplitude doesn't significantly affect the period.
In this comprehensive guide to Simple Harmonic Motion Worksheet Answers, we've covered core concepts, equations, and how to apply them to various physics problems. Understanding SHM not only aids in solving physics problems but also provides a foundation for studying more complex physical phenomena like waves and quantum mechanics.
What is the difference between a period and a frequency?

+
The period (T) is the time for one complete cycle of an oscillation, while the frequency (f) is the number of cycles in a second. They are reciprocals of each other; T = 1/f and f = 1/T.
Can the amplitude of an object in SHM change over time?

+
Yes, due to damping or other energy losses, the amplitude can decrease over time. This process is known as damped harmonic motion.
Why does the period of a pendulum not depend on its amplitude?

+
For small angles, the restoring force is proportional to the sine of the angle, which approximates to the angle itself. This linearity ensures the period remains constant despite changes in amplitude.
How does increasing mass affect the period of a spring-mass oscillator?
+
Increasing mass increases the period since the period T is proportional to the square root of mass (T ∝ √m), making the system oscillate more slowly.
What are real-life applications of SHM?

+
Simple Harmonic Motion appears in clocks (pendulums), shock absorbers in vehicles, tuning forks for sound, and even in biological systems like the heart’s natural rhythm.



