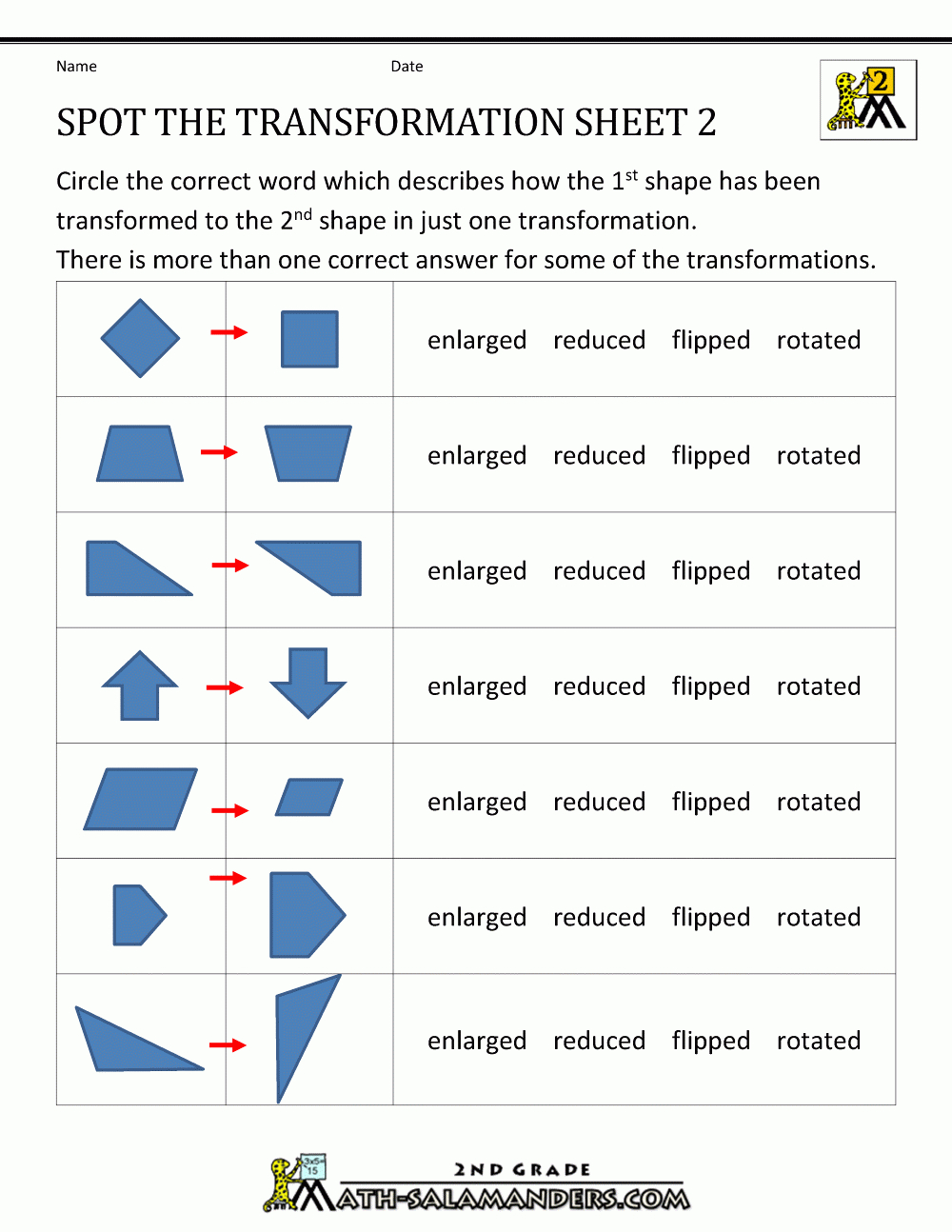
Geometry 1.3 Worksheet Answers: 5 Must-Know Solutions

Geometry, being a core component of mathematical education, often presents challenges to students. In this detailed exploration, we'll delve into the Geometry 1.3 worksheet, highlighting five pivotal solutions. These solutions not only offer answers but also provide insights into the fundamental geometric principles needed for understanding further topics. Let's embark on this journey through the intricacies of geometric problem-solving.
Key Concepts in Geometry 1.3

Geometry 1.3 typically covers:
- Points, Lines, and Planes
- Segments and Distance
- Midpoints
- Perpendicular Lines
- Parallel Lines and Planes
Understanding these concepts is vital as they form the foundation upon which more complex geometrical relationships are built.
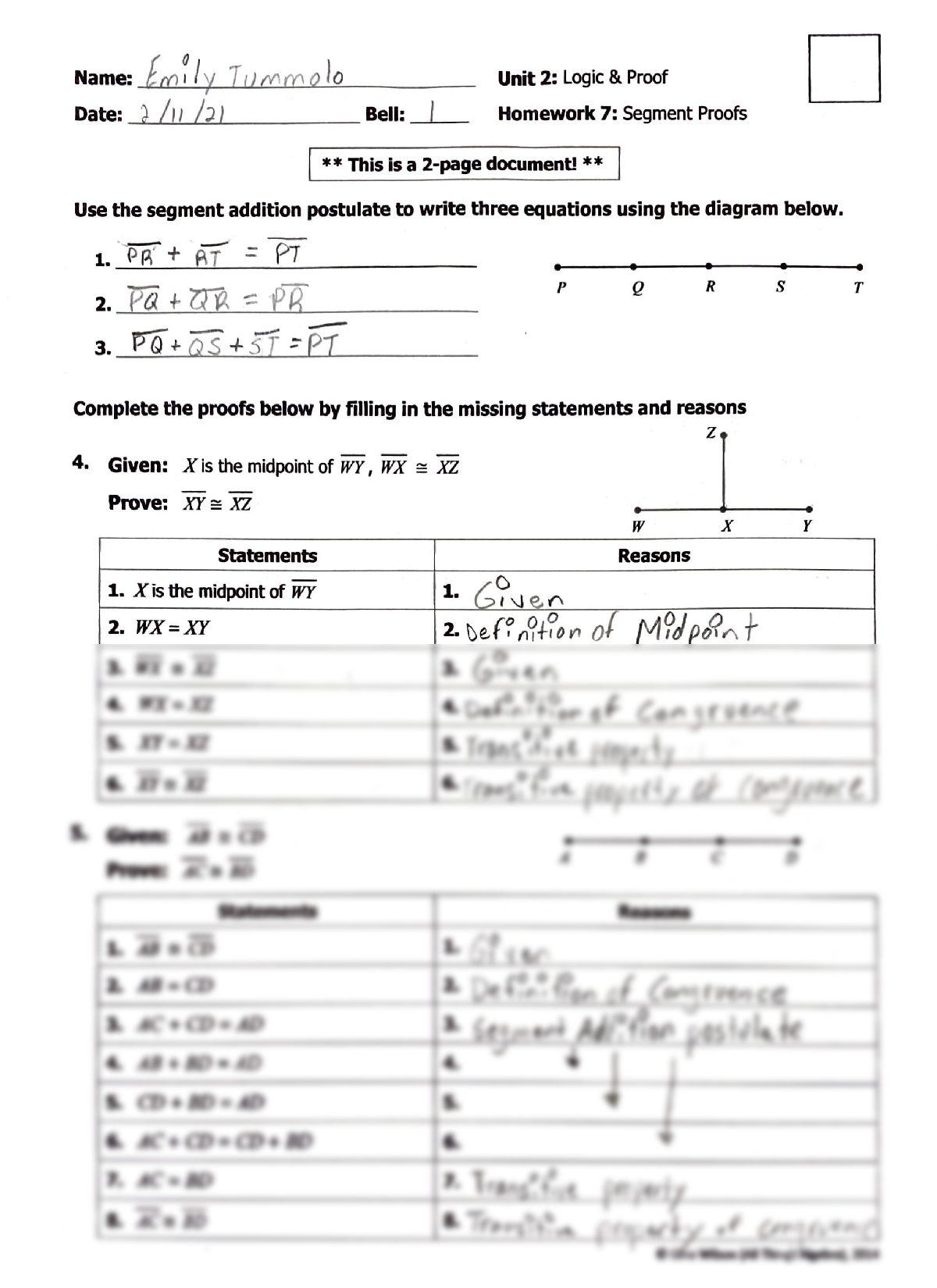
Problem 1: Midpoint Formula

The first problem in this worksheet usually introduces the midpoint formula. Here’s how to approach it:
- The midpoint M of a segment with endpoints A(x1, y1) and B(x2, y2) is calculated using the formula:
M = (\left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right))

Solution:

If you have two points, say A(3, 5) and B(7, 9), the midpoint calculation would be:
M = (\left( \frac{3 + 7}{2}, \frac{5 + 9}{2} \right)) = (5, 7)
📌 Note: Understanding the midpoint formula aids in solving problems related to distances and coordinate geometry.
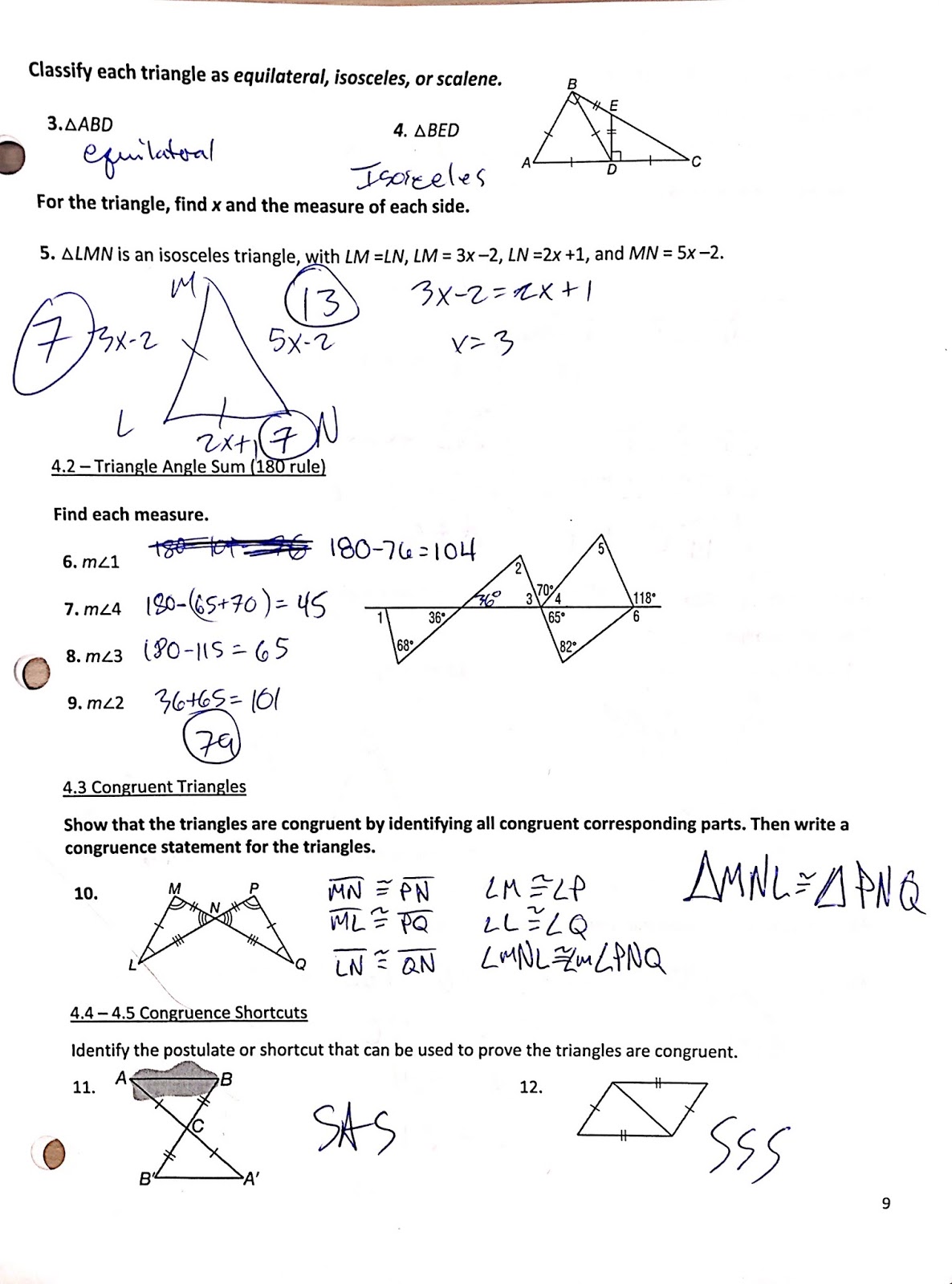
Problem 2: Parallel and Perpendicular Lines

One key question might ask to determine if two lines are parallel or perpendicular. Here are the concepts to grasp:
- Parallel lines have the same slope.
- Perpendicular lines have slopes that are negative reciprocals of each other.
Solution:

Given lines:
- Line 1 with a slope of 2
- Line 2 with a slope of -1⁄2
Since the slope of Line 1 is 2 and Line 2’s slope is -1⁄2, they are perpendicular. The product of their slopes equals -1.
| Line 1 Slope | Line 2 Slope | Relationship |
|---|---|---|
| 2 | -1⁄2 | Perpendicular |

Problem 3: Distance Formula

This problem involves using the distance formula to find the length of a line segment:
Distance = (\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2})
Solution:
If given points A(1, 2) and B(4, 6), you can calculate:
Distance = (\sqrt{(4 - 1)^2 + (6 - 2)^2}) = (\sqrt{9 + 16}) = 5
Problem 4: Slope of a Line

Understanding slope is fundamental in geometry. Here’s how to tackle related problems:
- Slope = (\frac{rise}{run} = \frac{y_2 - y_1}{x_2 - x_1})
Solution:

If you’re given points C(-2, 3) and D(4, 7):
Slope = (\frac{7 - 3}{4 - (-2)}) = (\frac{4}{6}) = (\frac{2}{3})
Problem 5: Equation of a Line

The final problem might involve deriving the equation of a line, often in point-slope or slope-intercept form:
- Point-Slope Form: ( y - y_1 = m(x - x_1) )
- Slope-Intercept Form: ( y = mx + b )
Solution:

If you know the slope ( m = -3 ) and a point (2, 1):
Point-Slope Form: ( y - 1 = -3(x - 2) ) Slope-Intercept Form: ( y = -3x + 7 )
By dissecting these five solutions, we've gained not just answers but a deeper understanding of the core geometric principles necessary for more advanced problems. Each problem sheds light on different aspects of geometry, from coordinate planes to properties of lines. Mastery of these concepts ensures a strong foundation in geometry, facilitating a smoother journey through future mathematical challenges.
Why is the midpoint formula important?
+
The midpoint formula is crucial for calculating the exact middle point between two points, which is vital in tasks involving distances, bisecting lines, and coordinate geometry.
How can I determine if two lines are perpendicular?
+
Two lines are perpendicular if the product of their slopes equals -1. If the slope of one line is (m), then the slope of the perpendicular line should be (-\frac{1}{m}).
What does the slope of a line represent?

+
The slope of a line gives you the inclination or the steepness of the line. A positive slope means the line rises as you move from left to right, a negative slope means it falls, and a zero slope indicates a horizontal line.



