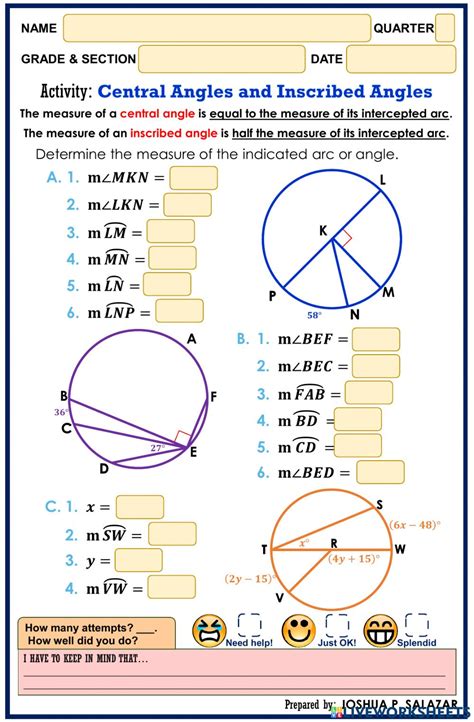
Segment Addition Worksheet: Mastering Geometry Basics

Geometry, the branch of mathematics concerned with shape, size, angles, and properties of space, can be incredibly satisfying once you get the hang of its basics. One fundamental concept that often serves as a stepping stone in understanding more complex geometrical problems is the segment addition postulate. This blog post will delve into the segment addition postulate, guide you through its principles, provide worksheets to practice, and help solidify your understanding of this essential geometry skill.
What is the Segment Addition Postulate?

The segment addition postulate is a basic but crucial geometric principle which states that if point B is on line segment AC, then the distance from A to B plus the distance from B to C will equal the total distance from A to C. Mathematically, this is expressed as:
AB + BC = AC
Here, A, B, and C are collinear points, with B being between A and C. This postulate is intuitive: if you were to walk from A to C, you could take a break at B, and the distance covered would still sum up to the total length of the segment AC.
Practical Applications of Segment Addition

- Road Mapping: When planning routes, knowing the distances between cities can help in calculating the total distance of travel if you were to go through certain landmarks or cities.
- Construction: Architects and builders use segment addition to measure distances and ensure the dimensions of structures are precise.
- Navigation: Pilots, sailors, and even hikers use this principle to determine distances and plan their journeys efficiently.
Steps to Solve Segment Addition Problems

To effectively use the segment addition postulate, follow these steps:
- Identify the points involved, particularly the point B which lies on segment AC.
- Determine the distances AB and BC.
- Apply the formula: AB + BC = AC.
Segment Addition Worksheet

Here’s a simple worksheet to practice segment addition:
| Problem | Given | Find |
|---|---|---|
| 1 | AB = 5 cm, BC = 3 cm | AC |
| 2 | AC = 10 cm, BC = 4 cm | AB |
| 3 | AB = 7 cm, AC = 12 cm | BC |

📝 Note: Ensure that all measurements are in the same unit before adding or subtracting.
Exploring Advanced Segment Addition

Once you’ve grasped the basics, you can dive into more complex scenarios:
- Using segment subtraction if point B is not between A and C but extends past C.
- Incorporating coordinate geometry where distances are calculated based on coordinates rather than given distances.
Concluding Thoughts

The segment addition postulate might seem simple, but it’s a foundational concept that aids in understanding more intricate geometrical problems. By practicing with worksheets and applying this postulate in real-life scenarios, you enhance your spatial reasoning and problem-solving skills. Remember, geometry is not just about formulas; it’s about visualizing space and understanding how shapes and distances interact. This concept will continue to prove useful as you advance in your studies, particularly when tackling more challenging topics like geometric proofs, coordinate geometry, and calculus.
What if Point B lies outside of Segment AC?

+
If B is not between A and C but extends past C, you’re dealing with segment subtraction instead of addition. The formula changes to: AC = BC - AB.
Can the segment addition postulate be applied in three-dimensional space?
+
Yes, the principle can be extended to three dimensions, where segments along different axes are added to find the total length or distance along a path.
What are common mistakes when using segment addition?
+
Common errors include not considering collinearity, misreading the diagram, or mixing up which segments are being added or subtracted.
Related Terms:
- Segment addition worksheet pdf
- Angle Addition Postulate worksheet
- Segment Addition Postulate worksheet pdf
- Segment addition worksheet with answers
- Midpoint Segment Addition Postulate worksheet