5 Tips for Mastering Central and Inscribed Angles
Unlocking the secrets of geometry often begins with understanding the basic concepts of angles, particularly central angles and inscribed angles. These are fundamental in both simple circles and complex geometrical shapes. Let’s dive deep into five tips that can help you master these concepts.
Understand the Basics

Before we delve into advanced techniques, understanding the basic definitions is crucial.
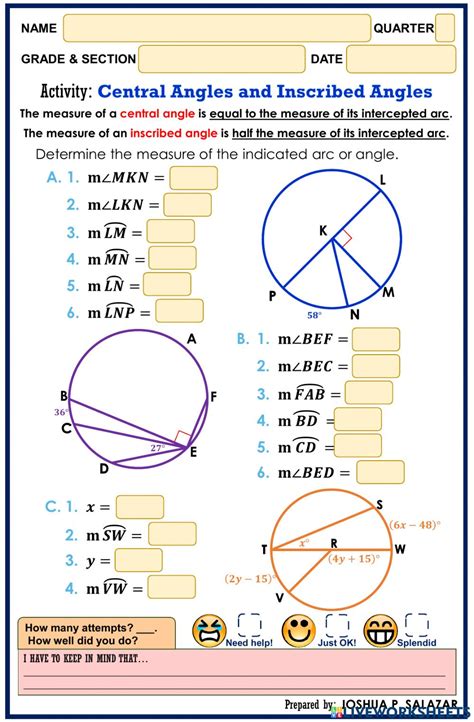
- Central Angles: These are angles whose vertices lie at the center of a circle. The measure of a central angle is equal to the arc it intercepts.
- Inscribed Angles: These angles have their vertices on the circumference of the circle, and the measure of an inscribed angle is half that of the central angle subtending the same arc.
Key Relationships:

- The measure of an inscribed angle is always half the measure of the central angle that cuts off the same arc.
- Angles subtended by the same arc, whether central or inscribed, are congruent.
Tip 1: Use Visual Aids

Geometry is a visual subject, so using diagrams can help significantly:
- Draw a circle and mark the points where the vertices of angles lie.
- Label the arcs and angles to keep track of your work.
- Visualize and compare the lengths of arcs for central and inscribed angles.

🎨 Note: Remember, the more you practice drawing, the better you'll become at spatial recognition and understanding geometrical relationships.
Tip 2: Leverage Theorems

There are several theorems that relate central and inscribed angles. Here’s a quick overview:
| Theorem | Description |
|---|---|
| Inscribed Angle Theorem | An inscribed angle is half the measure of the arc it intercepts. |
| Central Angle Theorem | The measure of a central angle is equal to the arc it intercepts. |
| Arc-Angle Relationship | Angles subtended by the same arc are congruent. |

📚 Note: Knowing these theorems will make solving problems faster and more accurate.
Tip 3: Practice with Different Configurations

Not all central and inscribed angles are straightforward. Here are some configurations to practice:
- Angles that share a common side with the diameter.
- Angles where one side is tangent to the circle.
- Angles formed by intersecting chords, secants, or tangents.

Tip 4: Understand Angle Relationships

The relationships between different types of angles in circles can simplify problem-solving:
- The sum of angles in a triangle inscribed in a semicircle is 90 degrees.
- The angles formed by intersecting chords, secants, or tangents have special properties.
- The angle between a tangent and a radius at the point of tangency is 90 degrees.
Tip 5: Solve Complex Problems

Once you’re comfortable with the basics, tackling more complex problems can solidify your understanding:
- Calculate the angles when given multiple intersecting lines or arcs.
- Determine lengths of chords and arcs based on angle relationships.
- Solve problems involving sectors and the golden ratio.
Applying these tips consistently will not only master your understanding of central and inscribed angles but also enhance your overall geometry skills.
In this journey through mastering central and inscribed angles, we've covered understanding the foundational concepts, leveraging visual aids, applying relevant theorems, exploring different configurations, understanding angle relationships, and tackling complex problems. By implementing these tips, your grasp on these geometric concepts will strengthen, allowing you to solve more intricate problems with confidence.
What is the difference between a central angle and an inscribed angle?

+
A central angle is an angle whose vertex is at the center of the circle, intercepting an arc equal in measure to the angle itself. An inscribed angle, on the other hand, has its vertex on the circumference of the circle, and its measure is half that of the central angle that cuts off the same arc.
How can I remember the theorems related to angles in circles?

+
One effective way is to associate each theorem with a mnemonic or a visual cue. For instance, you can remember the inscribed angle theorem with “Half the arc, half the angle.” Drawing and annotating diagrams also helps in visual learning.
Why are visual aids important in geometry?

+
Visual aids like diagrams make abstract concepts tangible, helping to visualize spatial relationships and understand complex geometrical properties more intuitively.