Ratios and Proportions Worksheet Solutions Revealed
If you're delving into the world of mathematics, understanding ratios and proportions is fundamental. Ratios help us compare quantities, and proportions provide a way to establish the relationships between these quantities. This blog post will guide you through the nuances of ratios and proportions, offering solutions to common problems that you might encounter in your academic journey or daily life.
Understanding Ratios

A ratio compares two or more quantities. Here's how you can identify and work with ratios:
- What is a Ratio? A ratio can be written in several ways:
- Using a colon like 3:1
- As a fraction like \frac{3}{1}
- With "to" such as 3 to 1
- Identifying Ratios: You'll often find ratios in recipes, blueprints, maps, and even in financial analysis. For example:
- A paint mix ratio might be 5 parts yellow to 3 parts blue (5:3)
- The screen resolution might be stated as 1920x1080, where the ratio of width to height is 1920:1080 or 16:9 after simplification.
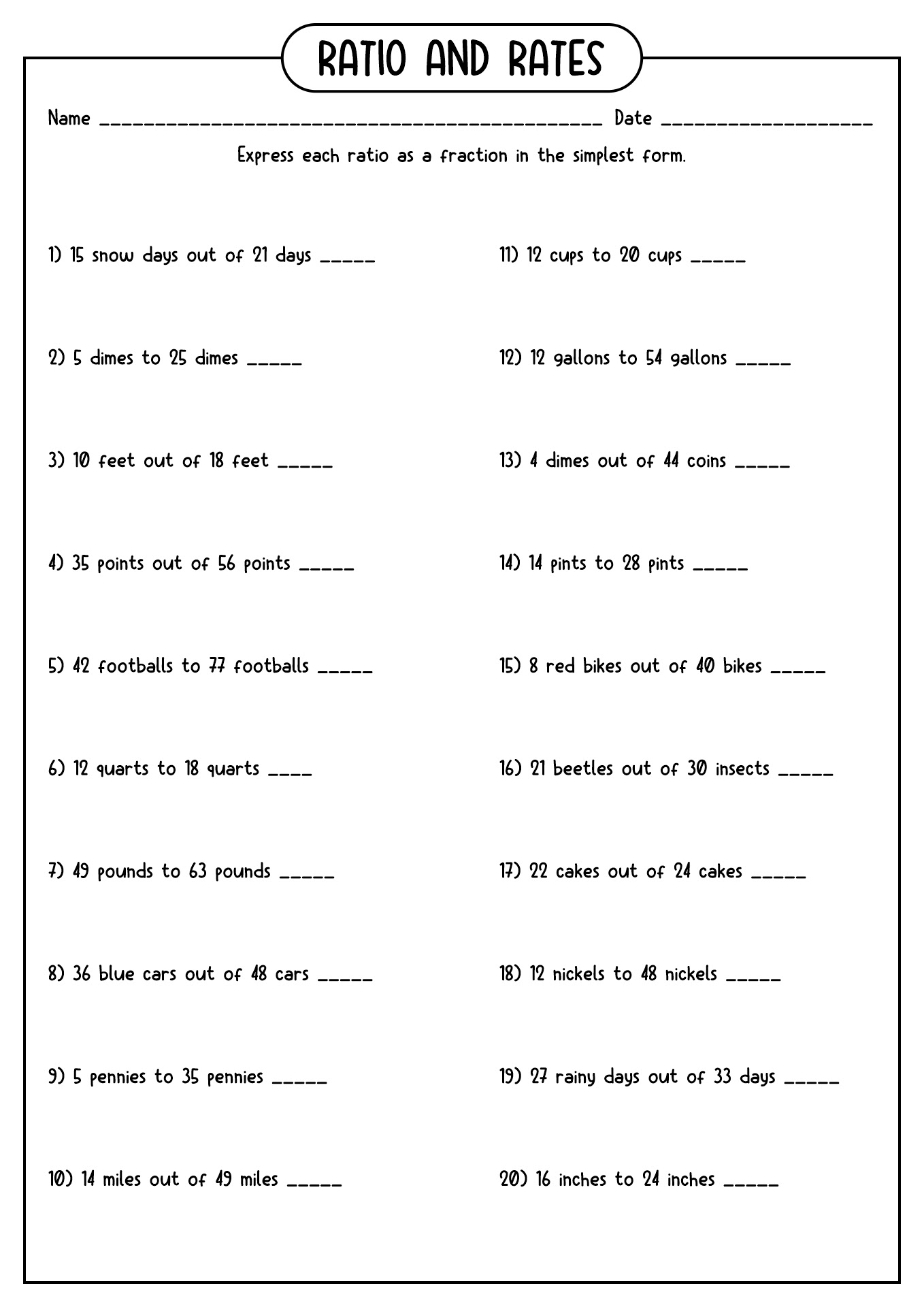
- Solving Ratio Problems: Here are some common problems and their solutions:
- Divide a total amount in a given ratio: If you need to divide 120 into the ratio 3:2, you would first find the total number of parts in the ratio (3+2=5). Then, you divide 120 by 5 to get the value of one part (24). Multiply this by 3 for the first portion (72) and by 2 for the second (48).
🔍 Note: When you simplify a ratio, ensure both quantities are either multiplied or divided by the same factor to keep the relationship intact.
Exploring Proportions

Proportions are equations that show two ratios are equal. Here's what you need to know:
- Setting up Proportions: If you have two ratios, like 2:5 and 4:10, they form a proportion because \frac{2}{5} = \frac{4}{10}.
- Solving for Unknown Values: When one part of a proportion is unknown, you can use cross multiplication. For instance, in \frac{3}{x} = \frac{6}{12}:
- Cross multiply to get 3 \times 12 = 6 \times x
- Which leads to 36 = 6x
- Divide both sides by 6: x = 6
Practical Applications

Understanding ratios and proportions can enhance your ability to deal with real-world scenarios. Here are some applications:
- Mixing Solutions: If you're in chemistry or cooking, knowing how to mix substances in a certain ratio is crucial. For example, if a cleaning solution requires a 1:4 mix of detergent to water, you need to understand how to scale this ratio.
- Scale Drawings: Architects and engineers use scale drawings to represent actual sizes. Understanding ratios allows you to convert these scales into real-life measurements.
- Financial Planning: Ratios are crucial in financial analysis, like debt-to-equity ratios, which help investors understand a company's financial health.
🔢 Note: Always ensure units are consistent when working with ratios for practical applications.
Advanced Ratio and Proportion Problems

Here are some advanced problems to test your understanding:
Problem 1: Ratio of Two Quantities with Variables

Solve for the ratio of quantities where one value depends on another:
- Problem: If the speed of a car increases proportionally to its acceleration, find the ratio of the speed after accelerating for 2 seconds to the speed after accelerating for 4 seconds. Assume acceleration is constant.
- Solution:
- Let initial speed be (S) and acceleration (a).
- After 2 seconds, speed is (S + 2a).
- After 4 seconds, speed is (S + 4a).
- The ratio would be (\frac{S + 2a}{S + 4a}).
- Simplify this: (\frac{S + 2a}{S + 4a} = \frac{S}{S + 4a} + \frac{2a}{S + 4a}).
- To simplify further, cross multiply and solve.
Problem 2: Ratio in Multiplicative Contexts

Solve for the ratio in contexts where multiplication changes one variable:
- Problem: If a population of bacteria triples every hour, find the ratio of the population after 2 hours to the population after 3 hours.
- Solution:
- After 2 hours, population is (P \times 3^2 = 9P).
- After 3 hours, population is (P \times 3^3 = 27P).
- Ratio is (\frac{9P}{27P} = \frac{1}{3}).
As we explore these ratios and proportions, remember that they are not just mathematical tools but are deeply embedded in how we understand and interact with the world. Whether you're adjusting a recipe, resizing an image, or analyzing financial statements, the principles of ratios and proportions are at play.
In summary, ratios and proportions allow us to make comparisons, understand relationships, and solve problems efficiently. By mastering these concepts, you'll be equipped to handle a range of mathematical problems with confidence.
What’s the difference between a ratio and a proportion?

+
A ratio compares two quantities while a proportion is an equation stating that two ratios are equal. Ratios help compare while proportions help in understanding relationships.
How do I solve a ratio word problem?

+
Identify the given ratio, the total number of parts, and the total amount to divide. Divide the total amount by the sum of parts, then multiply this by each part of the ratio to find the specific quantities.
Can ratios and proportions be used in everyday life?

+
Absolutely! They are used in cooking, scaling drawings, financial analysis, and many other practical applications where understanding quantities and their relationships is necessary.