5 Ways to Identify Rational vs. Irrational Numbers Instantly

Mathematics is often considered a universal language, and among its intriguing aspects are numbers and their classifications. A fundamental division in numbers that often comes up in mathematical discussions and analyses is the distinction between rational and irrational numbers. Knowing how to identify these types of numbers can enhance your problem-solving skills and deepen your understanding of mathematical principles.
1. Decimal Expansion

The most straightforward way to differentiate between rational and irrational numbers is by examining their decimal expansion:
- Rational Numbers: The decimal representation of a rational number either terminates (stops at some point) or eventually repeats itself. Examples include 0.5 (terminating) or 0.333... (repeating). The repeating sequence can be very long but will always follow a pattern.
- Irrational Numbers: The decimal expansions of irrational numbers neither terminate nor repeat. These numbers go on infinitely without any discernible repeating pattern, like π (pi) which is approximately 3.14159265358979...
2. Arithmetic Operations

Another method involves understanding how these numbers behave under arithmetic operations:
- Addition: The sum of two rational numbers or a rational and an irrational number is always rational, while the sum of two irrationals can be either rational or irrational.
- Subtraction: Similarly to addition, subtraction yields rational results from two rational numbers or one rational and one irrational. The subtraction of two irrationals can yield an irrational number or occasionally a rational one.
- Multiplication: Multiplying two rationals results in a rational number. However, multiplying two irrationals or a rational and an irrational can result in either a rational or an irrational number, although most often it will be irrational.
- Division: Dividing a rational by a non-zero rational or an irrational number can produce both rational and irrational results. When dividing by zero or by an irrational number, the result can be more complex or undefined.
3. Unique Representation

Here's an interesting trait of rational and irrational numbers:
The decimal expansion of rational numbers has an ending or a repeating sequence, meaning every rational number can be uniquely represented in its decimal form. On the other hand, an irrational number cannot be expressed as a ratio of two integers; thus, it has an infinitely non-repeating, non-terminating decimal expansion which means its value can never be fully captured in a finite or predictable manner.
4. Algebraic vs. Transcendental

There's a nuanced differentiation within irrational numbers:
- Algebraic Numbers: These are irrational numbers that are roots of non-zero polynomials with rational coefficients. For example, the square root of 2 or cube root of 3.
- Transcendental Numbers: These irrationals are not roots of any polynomial equation with rational coefficients, like π and e (Euler's number).
5. Density and Infinitude
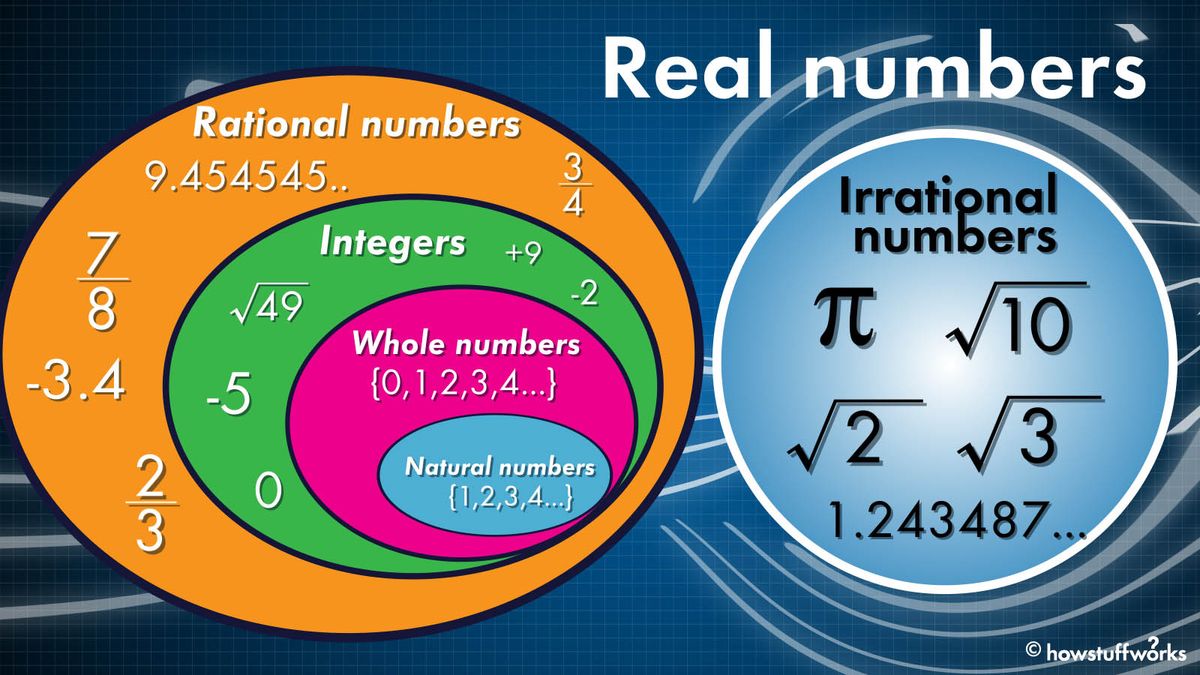
Lastly, we consider the distribution and countability of these numbers:
- Rational Numbers: While there are infinite rational numbers, they are countable, which means they can be put into one-to-one correspondence with the natural numbers. Their distribution is dense on the number line, meaning between any two rational numbers, there are infinitely many other rational numbers.
- Irrational Numbers: Irrationals are not countable; their density is so profound that between any two rational numbers, there exist uncountably many irrational numbers. They form the bulk of real numbers.
By incorporating these five methods into your mathematical toolkit, you'll gain a quick and efficient way to determine whether a number is rational or irrational. This knowledge not only supports advanced mathematical computations but also aids in understanding deeper properties of numbers and their applications in real-world problems, from physics to finance.
💡 Note: Even though π and e are both transcendental numbers, not all irrational numbers are transcendental. Algebraic irrational numbers are not necessarily transcendental.
To wrap things up, understanding the distinction between rational and irrational numbers not only deepens our appreciation for the complexity of numbers but also equips us with a foundation to tackle more advanced mathematical concepts. From financial calculations to solving complex engineering problems, these distinctions play a crucial role in providing precise and accurate results.
Can a fraction represent an irrational number?

+
No, fractions by definition represent rational numbers. An irrational number cannot be expressed as a simple ratio of two integers, and thus cannot be represented as a fraction.
Are all square roots irrational?

+
No, only the square roots of non-perfect squares (numbers that are not the result of multiplying an integer by itself) are irrational. For instance, √4 (2) is rational while √2 is irrational.
Why is π considered irrational?

+
π, or pi, is considered irrational because its decimal representation neither terminates nor enters into a repeating cycle, making it impossible to express as a simple fraction.