Ratio Problems Made Easy: 5 Quick Worksheets with Answers
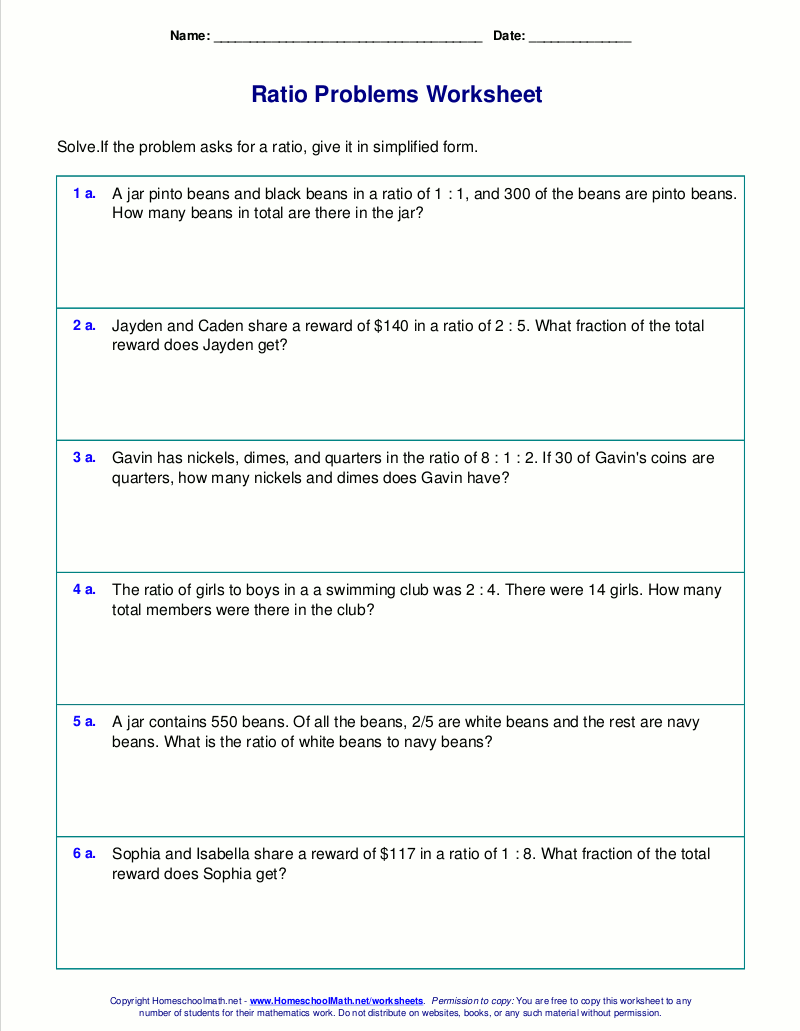
Welcome to our guide on mastering ratio problems! Whether you're a student preparing for exams, a parent helping with homework, or simply someone looking to brush up on mathematical skills, understanding ratios is essential. Ratios appear in various practical scenarios, from comparing quantities in cooking to analyzing financial data. Let's explore how to solve ratio problems effectively with five quick worksheets designed to clarify this concept.
What Are Ratios?

Before diving into the worksheets, let’s understand what ratios are. A ratio expresses the relationship between two quantities. It tells us how much of one thing there is compared to another. For example, if there are 3 apples for every 5 oranges, the ratio of apples to oranges can be written as:
- 3:5 (in numerical form)
- 3 to 5
- 3⁄5 (as a fraction)
🍎 Note: Ratios do not always sum up to 1 or 100; they merely show the proportion of one part to another.
Worksheet 1: Simple Ratios

Here’s your first worksheet focusing on basic ratio problems:
| Question | Answer |
|---|---|
| If the ratio of boys to girls in a class is 4:7, how many girls are there if there are 20 boys? | (20 * 7) / 4 = 35 girls |
| Given that the ratio of red marbles to blue marbles is 2:3, and there are 12 red marbles, how many blue marbles are there? | (12 * 3) / 2 = 18 blue marbles |

📝 Note: Use division to find the unknown part when you have one part and the total ratio.
Worksheet 2: Expanding Ratios

In this worksheet, we’ll expand ratios to understand how they change when quantities increase:
| Question | Answer |
|---|---|
| A mixture of juice and water is in the ratio 2:3. If you want to make 25 glasses of this mixture, how many glasses of juice are needed? | (2 / (2 + 3)) * 25 = 10 glasses of juice |
| If the ratio of sugar to flour in a recipe is 1:4 and you need to make a dough with 400g of flour, how much sugar do you need? | (1 / 4) * 400g = 100g of sugar |
Worksheet 3: Dividing Quantities by Ratios
Learn how to split quantities according to ratios:
| Question | Answer |
|---|---|
| If a sum of 120 is divided in the ratio of 3:2, how much will each share be?</td> <td>3/5 * 120 = 72, 2/5 * 120 = $48 | |
| A cake is shared in the ratio 5:3. If the total weight is 800g, what are the weights of the two pieces? | 5⁄8 * 800g = 500g, 3⁄8 * 800g = 300g |
Worksheet 4: Ratios in Real Life

Let’s look at how ratios apply to everyday situations:
| Question | Answer |
|---|---|
| If a car travels 3 miles on 1 gallon of gas, how many miles can it travel on 5 gallons? | 3 * 5 = 15 miles |
| In a company, the ratio of workers to managers is 10:1. If there are 200 workers, how many managers are there? | 200 / 10 = 20 managers |
Worksheet 5: Mixed Ratio Problems

Combine your learning with some challenging mixed problems:
| Question | Answer |
|---|---|
| If a painter mixes blue and yellow paint in the ratio 3:2 to make 500ml of green paint, how much blue paint is used? | (3 / 5) * 500ml = 300ml of blue paint |
| The ratio of boys to girls in a scout troop is 5:4. If there are 27 boys, how many scouts are in the troop? | 27 + (27 / 5) * 4 = 40 scouts |
These worksheets cover various aspects of ratio problems, helping you to understand not only how to solve them but also how they apply to real-world scenarios. By practicing these problems, you've built a foundation in understanding proportions and ratios, making it easier to tackle more complex mathematical concepts in the future.
What are ratios used for in real life?

+
Ratio are used for comparing quantities, making proportions in recipes, setting business targets, or even in financial calculations like comparing income to expenses.
How can I remember ratio formulas easily?

+
Relate ratios to everyday experiences like dividing a pizza or splitting money. Visual aids, mnemonics, or real-life applications can help with memory retention.
Can ratios be expressed as fractions or percentages?
+
Yes, ratios can be expressed as fractions (e.g., 2⁄3 for 2:3) or percentages (e.g., 66.67% for 2:3 when considering the total as 100%).
How do I solve a ratio problem when both parts are unknown?

+
Set up an equation based on the given ratio, then solve for both parts by defining one part as a variable and using algebra to find the total and divide according to the ratio.
What’s the difference between a ratio and a proportion?

+
A ratio compares quantities, while a proportion establishes equality between two ratios (e.g., 2:3 = 4:6). A proportion is essentially comparing two ratios to see if they are equivalent.