5 Quantum Numbers Practice Problems Explained

In the world of quantum mechanics, understanding the concept of quantum numbers is crucial for explaining the behavior of electrons in atoms. These numbers provide detailed insights into the energy levels and orbitals where electrons reside. This blog post will delve into 5 Quantum Numbers Practice Problems, explaining each step by step, so you can grasp this fundamental aspect of atomic theory better.
Problem 1: Electron Configuration of Hydrogen

Let’s start with the simplest atom, Hydrogen, which has only one electron:
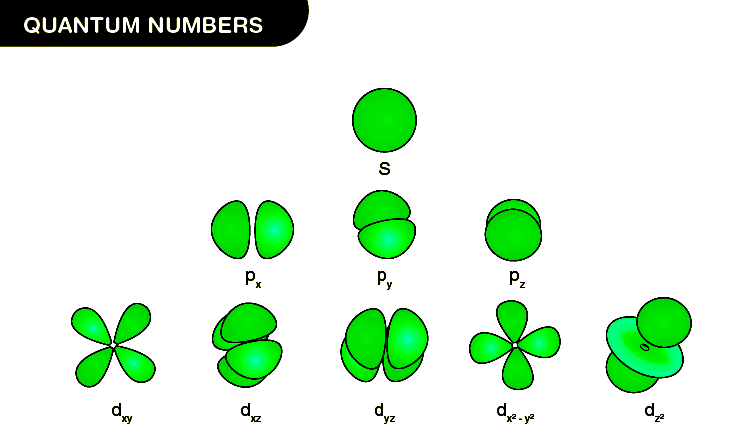
- Principal quantum number (n) - This dictates the energy level or shell in which the electron resides. For Hydrogen’s ground state, n = 1.
- Angular Momentum Quantum Number (l) - This specifies the shape of the orbital. Since there’s only one electron, l = 0 for an s-orbital.
- Magnetic Quantum Number (m_l) - Indicates the orientation of the orbital in space. For l = 0, m_l can only be 0.
- Spin Quantum Number (m_s) - Represents the electron’s spin, which can be either +1⁄2 or -1⁄2. Let’s assume it’s +1⁄2 for simplicity.
🚀 Note: The spin quantum number doesn't change the shape or orientation of the orbital; it determines the electron's intrinsic angular momentum.
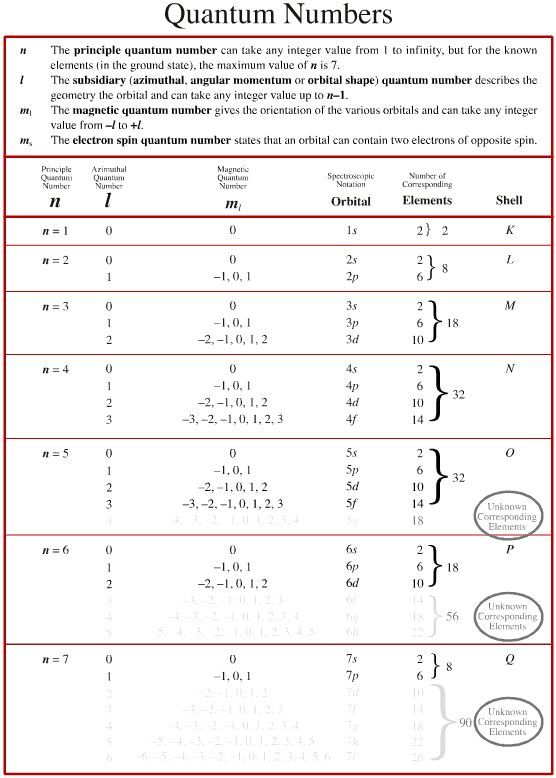
Problem 2: Assign Quantum Numbers for Oxygen

Oxygen has 8 electrons, and we’ll look at one electron in its 2p orbital:
- n - For the second energy level, n = 2.
- l - p-orbitals correspond to l = 1.
- m_l - With l = 1, m_l can be -1, 0, or 1. Let’s choose m_l = -1.
- m_s - Electron spin will be +1⁄2 or -1⁄2. We’ll select -1⁄2.
| Quantum Number | Range | Value |
|---|---|---|
| n | 1, 2, 3... | 2 |
| l | 0 to n-1 | 1 |
| m_l | -l to +l | -1 |
| m_s | +1/2, -1/2 | -1/2 |
Problem 3: Quantum Number Violations
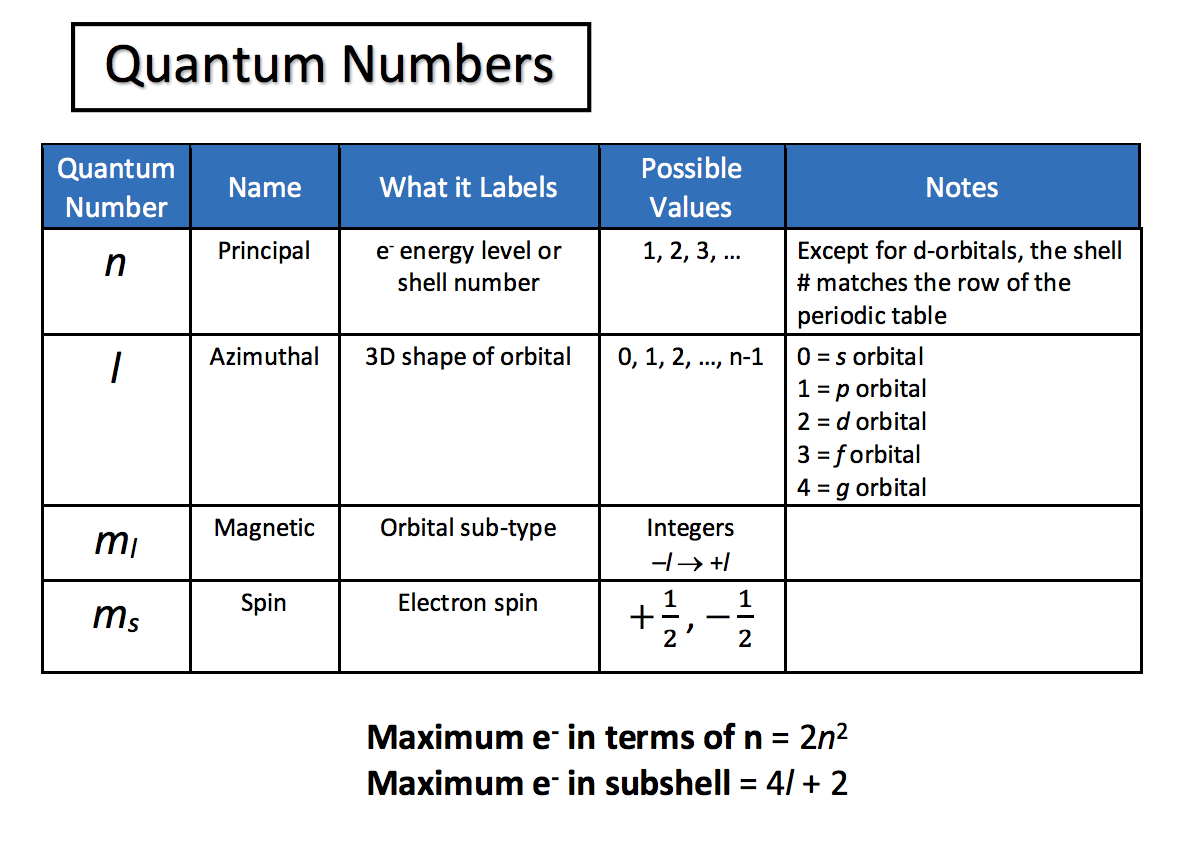
Consider an electron with these quantum numbers: n = 3, l = 4, m_l = -1, and m_s = +1⁄2.
- Violation: l can only go from 0 to n-1, meaning l cannot be 4 when n is 3.
🚨 Note: Invalid quantum number configurations can help in understanding the limits and rules of quantum mechanics.
Problem 4: Chromium Electron Configuration Anomaly

The electron configuration of Chromium (Cr) demonstrates a common anomaly:
- In its ground state, Chromium has an electron configuration [Ar] 3d5 4s1 rather than [Ar] 3d4 4s2 due to:
- Stability from Half-filled Orbitals - Chromium achieves a more stable configuration by having half-filled 3d orbitals.
- Exception Quantum Numbers: In the 3d orbital, n = 3, l = 2, m_l = -2, -1, 0, +1, +2, and m_s = ±1/2 for each electron.
Problem 5: Quantum Numbers for Boron

For Boron (B), which has 5 electrons:
- n = 2 - All valence electrons are in the second energy level.
- l - For its single electron in 2p, l = 1.
- m_l = -1, 0, +1 - for the possible orientations of 2p orbitals, choose m_l = 0.
- m_s = +1⁄2 or -1⁄2 - Select m_s = +1⁄2.
Understanding quantum numbers not only helps in predicting electron configurations but also aids in comprehending the intricate nature of atomic physics. By assigning and understanding these numbers, we can predict the behavior of electrons with high precision, leading to breakthroughs in chemistry and physics.
What are quantum numbers used for?

+
Quantum numbers describe the characteristics of electrons in atoms, detailing their energy, location, and spin. They’re vital for understanding the periodic table, chemical bonding, and atomic theory.
Why do electrons fill orbitals in a specific order?

+
Electrons fill orbitals according to the Aufbau Principle, the Pauli Exclusion Principle, and Hund’s Rule to minimize the total energy of an atom while maintaining stability and symmetry in the electronic structure.
Can electrons ever have the same quantum numbers?

+
No, according to the Pauli Exclusion Principle, no two electrons in the same atom can have the same set of all four quantum numbers. This principle is fundamental to the organization of the electron cloud around the nucleus.
What’s the significance of electron spin?

+
Electron spin contributes to the magnetic properties of atoms and plays a key role in phenomena like electron magnetism, spin resonance, and bonding. It also has applications in quantum computing.
How do quantum numbers relate to electron configuration?

+
Quantum numbers assign specific locations and properties to electrons within an atom’s electron shell, directly influencing the electron configuration that reflects the element’s chemical behavior.



