5 Proven Methods to Solve Triangle Similarity Problems

There are several effective methods for tackling triangle similarity problems, each with its own advantages. Whether you're a student looking to grasp the concept of triangle similarity for an exam or an enthusiast keen on understanding geometric principles, here are five proven techniques to ensure you can solve any triangle similarity problem with confidence.
1. AA (Angle-Angle) Similarity Criterion


The AA criterion is often the first similarity postulate students learn. This method is straightforward:
- If two angles of one triangle are congruent to two angles of another triangle, the triangles are similar by the AA criterion.
- This is because all triangles have angles that sum up to 180°, so if you know two angles are equal, the third angle must also be equal, making the triangles similar.
This method is intuitive because it’s based on the recognition of equal angles, which are easier to identify.
2. SSS (Side-Side-Side) Similarity Theorem


When dealing with sides instead of angles, the SSS method comes into play:
- If the ratios of all corresponding sides of two triangles are equal, the triangles are similar.
- Here’s an example:
- Triangle ABC with sides AB = 3, BC = 4, AC = 5
- Triangle DEF with sides DE = 9, EF = 12, DF = 15
- The ratio of sides AB to DE is 3⁄9 or 1⁄3, BC to EF is 4⁄12 or 1⁄3, and AC to DF is 5⁄15 or 1⁄3. Since all ratios are equal, these triangles are similar.
3. SAS (Side-Angle-Side) Similarity Theorem


The SAS theorem uses both angles and sides:
- If two sides of one triangle are proportional to two sides of another triangle, and the included angles are congruent, the triangles are similar.
- Key steps:
- Identify the two proportional sides.
- Ensure the included angle (the angle formed by these sides) is congruent.
4. Using the Law of Sines
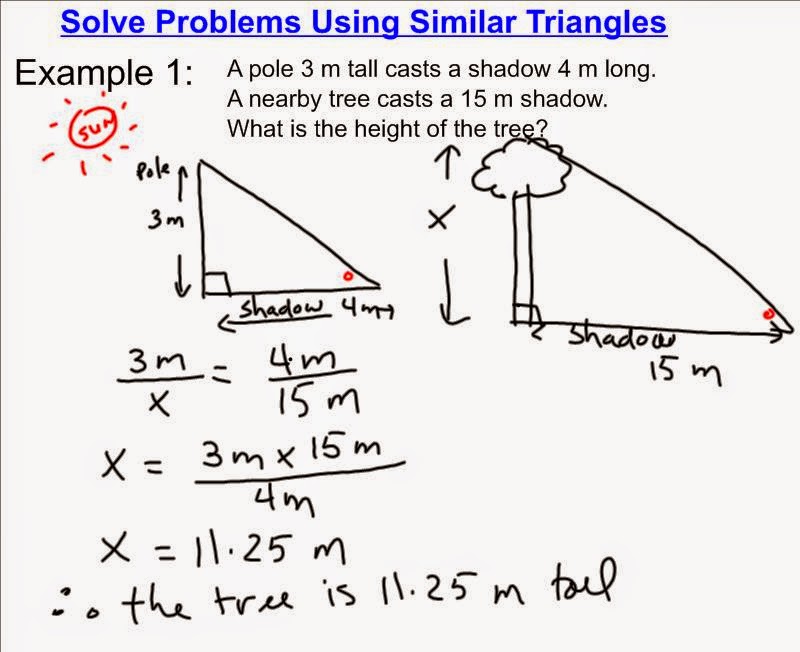

The Law of Sines, which states that for any triangle sin(A)/a = sin(B)/b = sin©/c, can be used to find if triangles are similar:
- If you can establish that the ratios of the sides of two triangles to their corresponding sine angles are equal, then the triangles are similar.
- This method is often useful when angles and sides are given in a less straightforward manner.
5. Proportionality of Segments


Another often overlooked but powerful method:
- If a line is drawn parallel to one side of a triangle, the line divides the other two sides proportionally.
- This method can be used to determine similarity:
- If the segments are cut by parallel lines, they form triangles similar to the original triangle.
- This is due to the Basic Proportionality Theorem (or Thales’ Theorem).
📝 Note: Ensure you check both corresponding angles and sides when verifying similarity. It’s easy to forget to confirm both criteria.
In summary, mastering triangle similarity involves recognizing different criteria for similarity, from angles to sides and even the relationship between lines. Each method has its unique strengths, and often, you might find combining these methods yields quicker results or a more intuitive solution. Whether it’s by recognizing equal angles, using proportional sides, or applying more advanced geometric principles, these five methods provide the tools necessary to solve triangle similarity problems efficiently.
What is the difference between triangle congruence and triangle similarity?

+
Congruent triangles have the same size and shape, with all corresponding sides and angles equal. Similar triangles, on the other hand, only have the same shape; their sides are proportional but not necessarily the same length.
Can triangles be similar if they have different orientations?

+
Yes, orientation does not affect triangle similarity. As long as the corresponding angles are equal and sides are proportional, the triangles are similar regardless of their orientation in space.
How can the AA similarity criterion be applied in real-world scenarios?

+
The AA similarity criterion can be used in applications like engineering, architecture, and surveying to determine the height of buildings or mountains based on angle measurements from different known distances.