5 Tips for Mastering Unlike Fractions Subtraction
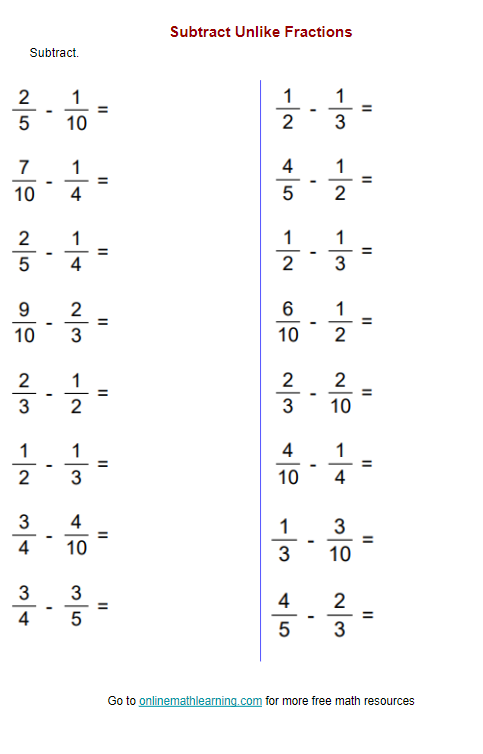
Subtracting unlike fractions can often seem daunting, especially when the denominators are not common. However, with a few strategic tips and tricks, you can master this essential math skill with ease. Whether you're a student brushing up for a test or an adult revisiting your numeracy skills, these five tips will help you navigate through unlike fractions subtraction effortlessly.
Understanding Unlike Fractions


Before diving into the subtraction process, it’s crucial to understand what unlike fractions are. Unlike fractions have different denominators, unlike like fractions which share a common denominator. For example, 1⁄2 and 1⁄3 are unlike fractions because they have different denominators, whereas 2⁄5 and 3⁄5 are like fractions.
Tip 1: Find a Common Denominator

To subtract unlike fractions, you first need a common ground:
- List the Multiples: Find the Least Common Multiple (LCM) of the two denominators. This becomes your new common denominator.
- Convert the Fractions: Adjust both fractions so that they have this common denominator. This often means multiplying both the numerator and the denominator by the same number.
🔢 Note: Remember that you’re not changing the value of the fractions, just their representation.
Tip 2: Use the Cross-Multiplication Technique
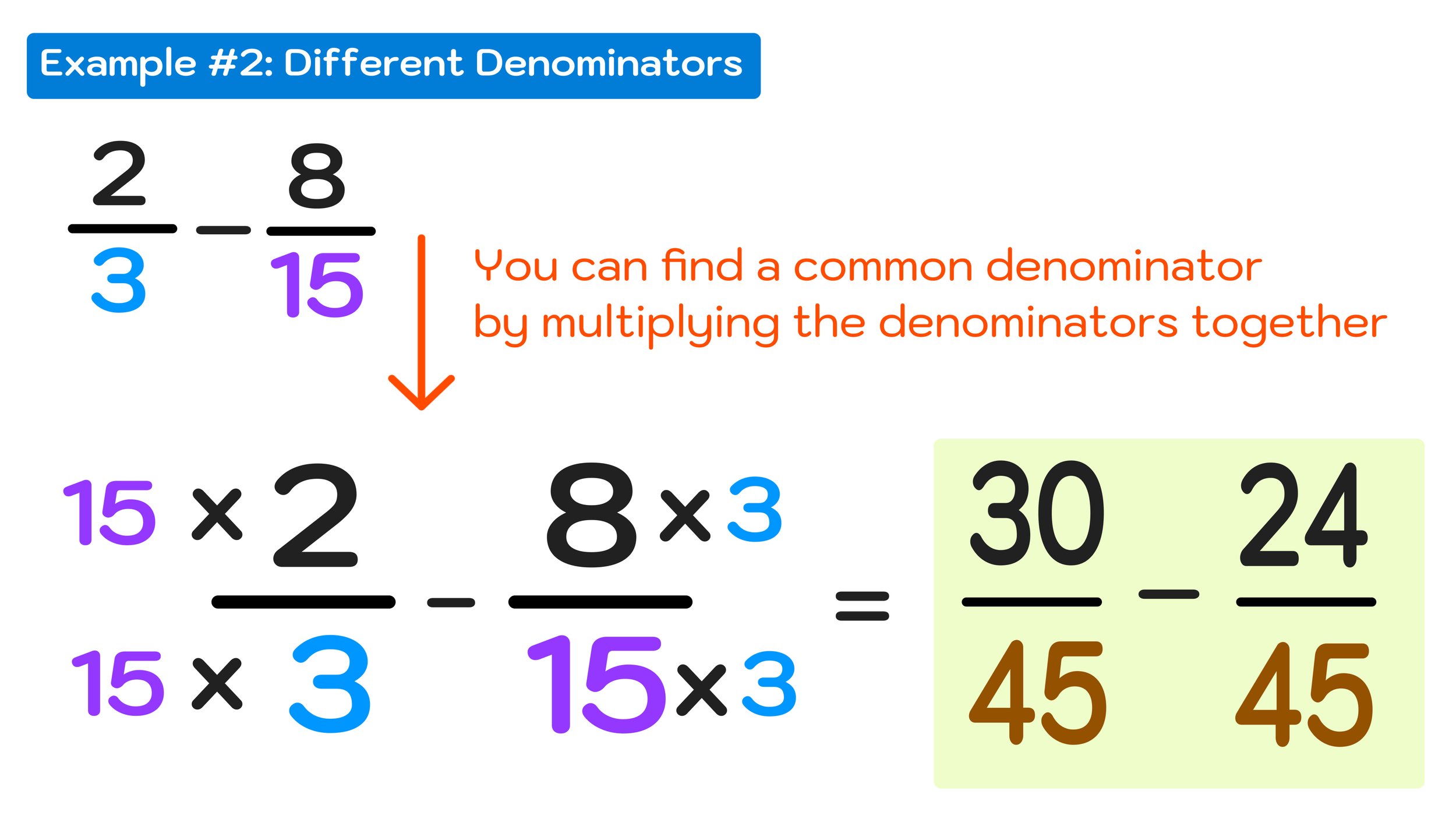
When denominators aren’t immediately recognizable, cross-multiplication can come in handy:
- Multiply: Multiply the numerator of one fraction by the denominator of the other, and vice versa.
- Subtract: Subtract the first result from the second to get the new numerator over the common denominator.
| Example | Operation | Result |
|---|---|---|
| 1⁄2 - 1⁄3 | (1 * 3) - (1 * 2) = 3 - 2 | 1⁄6 |

Tip 3: Simplification After Subtraction

Once you’ve subtracted the fractions, simplification can make the result more manageable:
- Greatest Common Divisor (GCD): Find the GCD of the numerator and the new denominator.
- Divide: Divide both the numerator and the denominator by their GCD to simplify the fraction.
Tip 4: Use Visuals to Understand

Visual aids can significantly enhance your understanding of unlike fractions:
- Use Shapes or Diagrams: Draw or visualize fractions as parts of a whole. This can make the concept of subtraction more tangible.
- Online Tools: There are plenty of digital tools that help visualize fraction subtraction.
📝 Note: Visual aids are especially beneficial for visual learners to grasp the concept of unlike fractions subtraction.
Tip 5: Practice Makes Perfect

Like any skill, proficiency in subtracting unlike fractions comes with practice:
- Regular Drills: Do regular subtraction exercises. Start with easier fractions and move to more complex ones.
- Real-life Applications: Apply this knowledge in everyday scenarios like cooking or DIY projects where measurements are crucial.
In mastering the art of subtracting unlike fractions, you’ve uncovered a blend of patience, strategy, and practice. These five tips not only simplify the process but also build your confidence in handling numerical problems. By understanding the fundamental concepts, finding common denominators, employing cross-multiplication when needed, simplifying results, using visual aids, and regularly practicing, you’re well on your way to becoming a pro in fraction subtraction. The journey of mastering unlike fractions subtraction is indeed a testament to the beauty of math – where numbers dance in harmony, giving us a deeper appreciation for the logic and structure behind the numbers.
Why do I need to find a common denominator for unlike fractions?

+
Finding a common denominator allows you to operate on fractions as if they were parts of the same whole, making addition and subtraction easier and more accurate.
Can I subtract fractions without changing their denominators?

+
Not directly. For unlike fractions, you must first convert them to have a common denominator before performing any arithmetic operation.
How can visual aids help in understanding unlike fractions subtraction?

+
Visual aids help by representing fractions as parts of a shape or diagram, making it easier to understand and calculate the difference between fractions with different denominators.
What are some common mistakes when subtracting unlike fractions?

+
Common mistakes include forgetting to convert both fractions to a common denominator, incorrectly simplifying the result, or not finding the least common denominator, which can make the process unnecessarily complex.
What is the significance of practicing fraction subtraction?

+
Practicing helps in reinforcing the conceptual understanding and procedural knowledge, improving speed and accuracy in real-world applications and academic settings.



