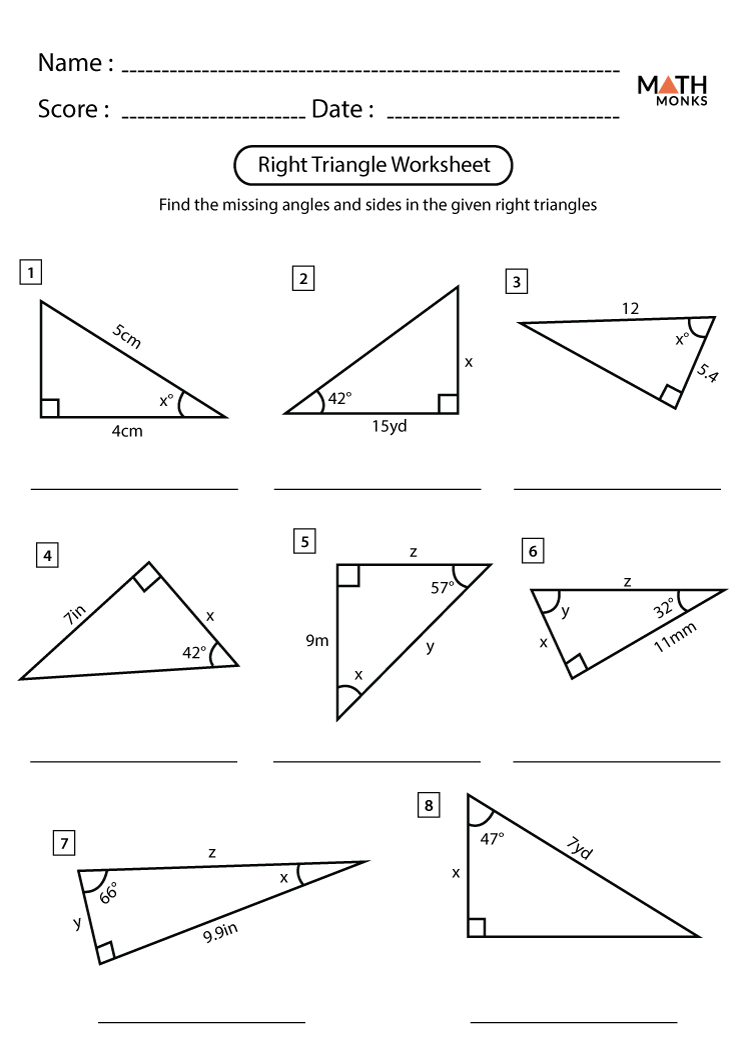
5 Methods to Prove Triangles Congruent Fast

Understanding and proving triangle congruence is a fundamental aspect of geometry that can be both challenging and rewarding. Whether you're preparing for an exam or just brushing up on your mathematical skills, knowing the different methods to prove triangles congruent will make your geometry journey much smoother. In this post, we'll delve into five key methods for proving triangle congruence and discuss how you can quickly determine if two triangles are congruent using these methods.
Method 1: Side-Angle-Side (SAS) Congruence

When proving that two triangles are congruent using the SAS method, you need to show that:
- Two sides of one triangle are equal to two sides of the other triangle.
- The included angle (the angle between the two sides) is also equal.
Why does it work? The SAS postulate works because the two sides and the angle between them essentially lock the triangle into a unique shape, making any two triangles with these congruences identical.
Method 2: Side-Side-Side (SSS) Congruence

If all three sides of one triangle are equal to all three sides of another triangle, then the triangles are congruent by the SSS method. This approach includes:
- Three sides of one triangle being equal to three sides of the other.
When you're proving triangles congruent using SSS, you're essentially saying that the triangles are like rigid bodies that cannot be reshaped unless all corresponding sides match.
Method 3: Angle-Side-Angle (ASA) Congruence

With ASA, you prove congruence by:
- Showing two angles and the included side of one triangle equal to two angles and the included side of another triangle.
The significance of ASA is that once you know two angles, the third is determined, ensuring the triangles have the same shape.
Method 4: Angle-Angle-Side (AAS) Congruence

This method is often confused with ASA, but here's how AAS works:
- Two angles of one triangle are congruent to two angles of another.
- One side (not included between the angles) of one triangle is congruent to the corresponding side of the other.
The AAS method leverages the fact that if two triangles have two angles and one side congruent, the triangles must be congruent because the remaining side will match up due to the parallel postulate.
Method 5: Right Angle-Hypotenuse-Leg (HL) Congruence

Specific to right triangles, the HL congruence method states that:
- If the hypotenuse and one leg of one right triangle are congruent to the hypotenuse and one leg of another right triangle, the triangles are congruent.
This is a handy method because it often simplifies proving right triangle congruence, especially in complex geometric problems where other methods might not apply.
Choosing the Right Method

When faced with a triangle congruence problem, the key is to:
- Identify which information you have available.
- Choose the method that allows you to prove congruence with the least steps possible.
💡 Note: While these methods are definitive ways to prove triangle congruence, some problems might need additional geometric properties or theorems to be fully solved.
Understanding how to prove triangle congruence can vastly simplify solving geometric problems. Here's a summary of what we've learned:
- SAS: Two sides and the included angle must be congruent.
- SSS: All three sides must be congruent.
- ASA: Two angles and the included side must be congruent.
- AAS: Two angles and a non-included side must be congruent.
- HL: For right triangles, the hypotenuse and one leg must be congruent.
These methods provide a quick and reliable path to determine if two triangles are congruent, which in turn helps in proving further geometric theorems and solving complex problems. The journey through geometry isn't just about applying these rules; it's about understanding how shapes relate to each other, which opens up a whole new world of mathematical exploration and appreciation.
Why are triangles considered congruent?

+
Triangles are considered congruent when all corresponding sides and angles are exactly the same, making them identical in shape and size. This concept helps simplify geometric proofs and problem-solving.
Can I use SAS when two angles are congruent?

+
No, SAS requires two sides and the included angle to be congruent. If you have two angles, consider using ASA or AAS.
Is HL method only for right triangles?
+
Yes, the HL method applies only to right triangles, where you compare the hypotenuse and one leg.