Free Place Value Worksheets for Thousands Practice

Place value is a fundamental concept in mathematics that helps students understand the value of digits in different positions within a number. It's crucial for building arithmetic skills, especially when working with thousands, hundreds, tens, and ones. Whether you're a teacher, parent, or student looking to improve your skills or reinforce learning, having access to free place value worksheets can be incredibly beneficial. In this comprehensive guide, we will delve into various activities and resources to help you practice place value focusing on thousands.
Why Is Practicing Place Value Important?

Understanding place value is key for:
- Mastering arithmetic operations like addition, subtraction, multiplication, and division.
- Interpreting larger numbers accurately.
- Estimating and rounding numbers effectively.
- Developing a foundation for more complex mathematical concepts.
Understanding Place Value with Thousands

Here are some core concepts to keep in mind:
- The thousands place is the fourth digit from the right.
- Each digit in a number represents different values depending on its position.
- Zero as a placeholder: It retains the value of higher place values when preceding them.
- The value of a digit can change when it moves from one place to another.

How to Practice Place Value for Thousands
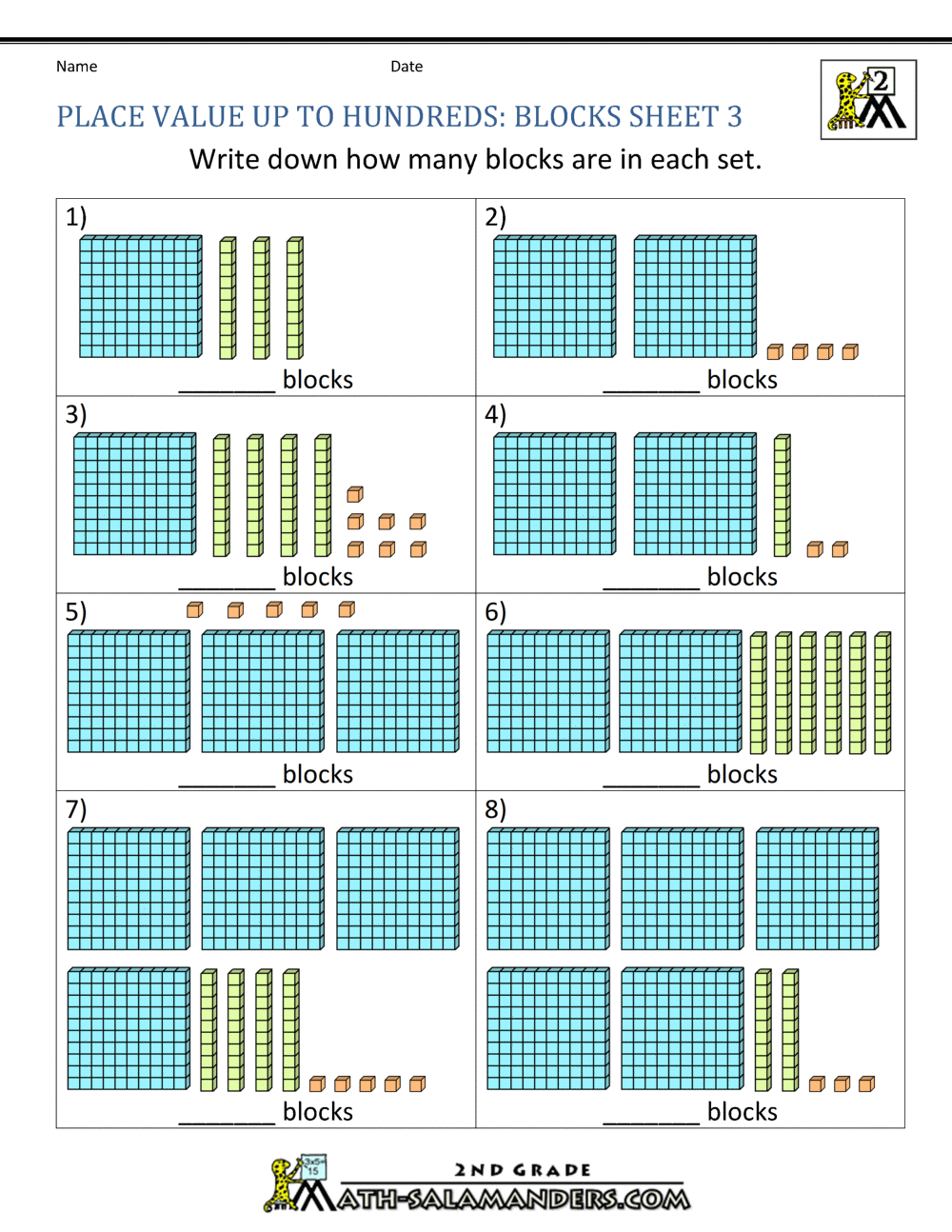
Here are some ways to practice place value with a focus on thousands:
- Place Value Chart - Use a place value chart to visually represent the value of each digit in a number.
- Reading Numbers - Practice reading numbers correctly to understand the importance of each digit’s place.
- Rounding Numbers - Learn to round numbers to the nearest thousand to understand the impact of place value.
- Word Problems - Solve problems where place value knowledge is essential.
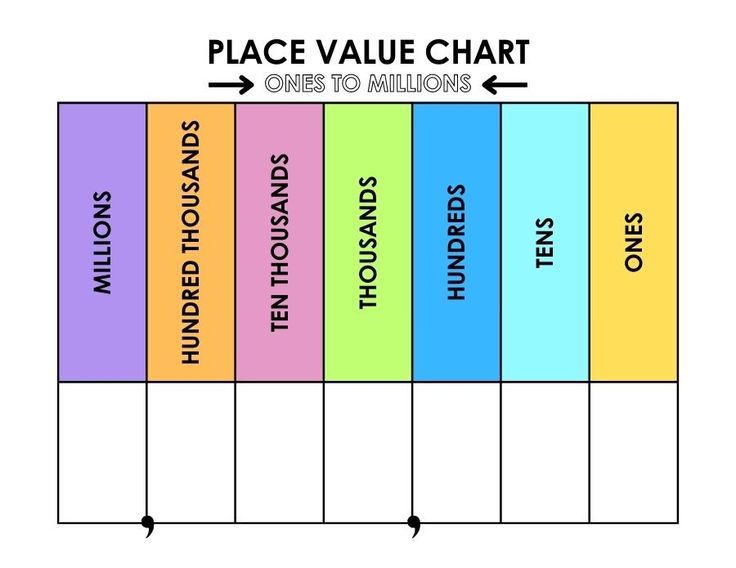
Using a Place Value Chart
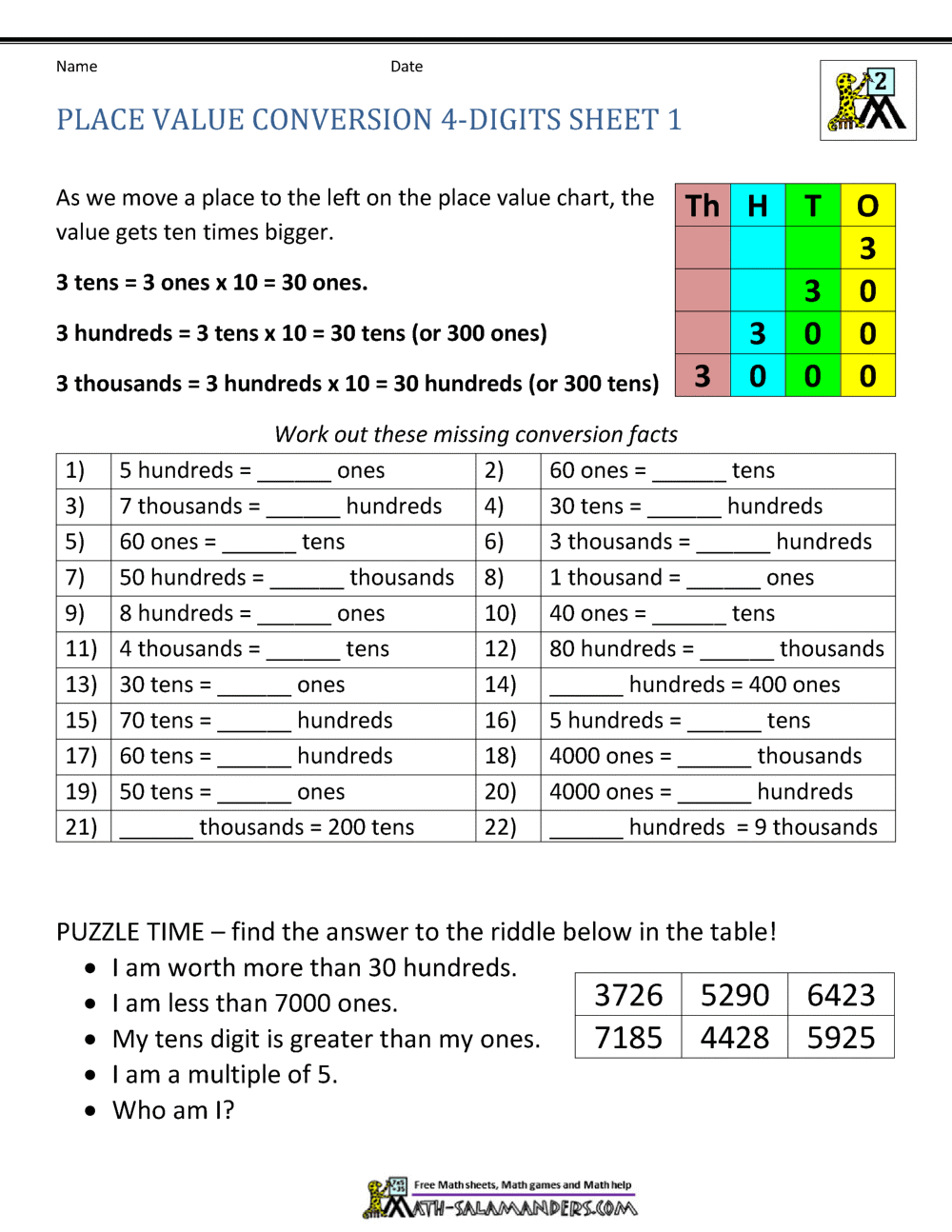
A place value chart visually separates the number into its constituent parts:
| Millions | Hundred Thousands | Ten Thousands | Thousands | Hundreds | Tens | Ones |
|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 2 | 4 | 8 | 3 |

This table shows that the number 2,483 has a 2 in the thousands place, meaning the number is 2 thousand, 4 hundreds, 8 tens, and 3 ones.
📝 Note: While practicing with a chart, ensure that numbers are expanded to emphasize the thousands place, like writing 2,483 as 2000 + 483.
Reading Numbers

Reading numbers correctly involves identifying each digit’s place value:
- The number 1,093 is read as “one thousand ninety-three.”
- The number 13,467 is read as “thirteen thousand four hundred sixty-seven.”
Rounding Numbers to the Nearest Thousand
Here’s how you can round numbers:
- If the hundreds digit is 5 or more, round up the thousands digit.
- If the hundreds digit is less than 5, keep the thousands digit as is.
- Examples:
- 2,483 rounds to 2,000.
- 3,545 rounds to 4,000.
Word Problems

Word problems can be a fun way to apply place value in practical scenarios. Here are some examples:
- John has 3,875 apples. If he gives away 1,000 apples, how many will he have left?
- If a store has 2,300 books in stock and they order an additional 700 books, how many books will be in the store altogether?
📝 Note: Word problems can be scaled for difficulty by changing the numbers involved or introducing multiple-step problems.
By practicing these methods, students will gain a solid understanding of place value, particularly with large numbers involving thousands. Remember, repetition and variety in exercises can solidify this concept over time.
What is the importance of understanding place value in mathematics?

+
Place value is crucial for several reasons. It allows students to comprehend the value of digits based on their position in a number, which is essential for operations like addition, subtraction, multiplication, and division. Additionally, it lays the groundwork for understanding larger numbers, decimals, and other advanced mathematical concepts.
How can I make place value practice engaging for students?
+
To make place value practice engaging, incorporate hands-on activities like using manipulatives, playing math games, and integrating technology. For example, using physical base ten blocks to represent numbers, creating "number stories," or utilizing educational apps that focus on place value can be both fun and educational.
Can you give examples of place value errors?

+
Common place value errors include reading numbers incorrectly (e.g., reading 3,465 as "thirty four thousand six hundred five"), misunderstanding the value of zero in place value (e.g., thinking that 3000 is less than 2999), or not recognizing the shift in value when moving digits (e.g., thinking 41 is greater than 140).
Understanding place value, especially with thousands, is more than just knowing what numbers are; it’s about interpreting their worth within the mathematical system. This understanding underpins various mathematical operations and problem-solving skills. By employing the various techniques and exercises mentioned, students can grasp this vital concept, laying a strong foundation for future mathematical learning.