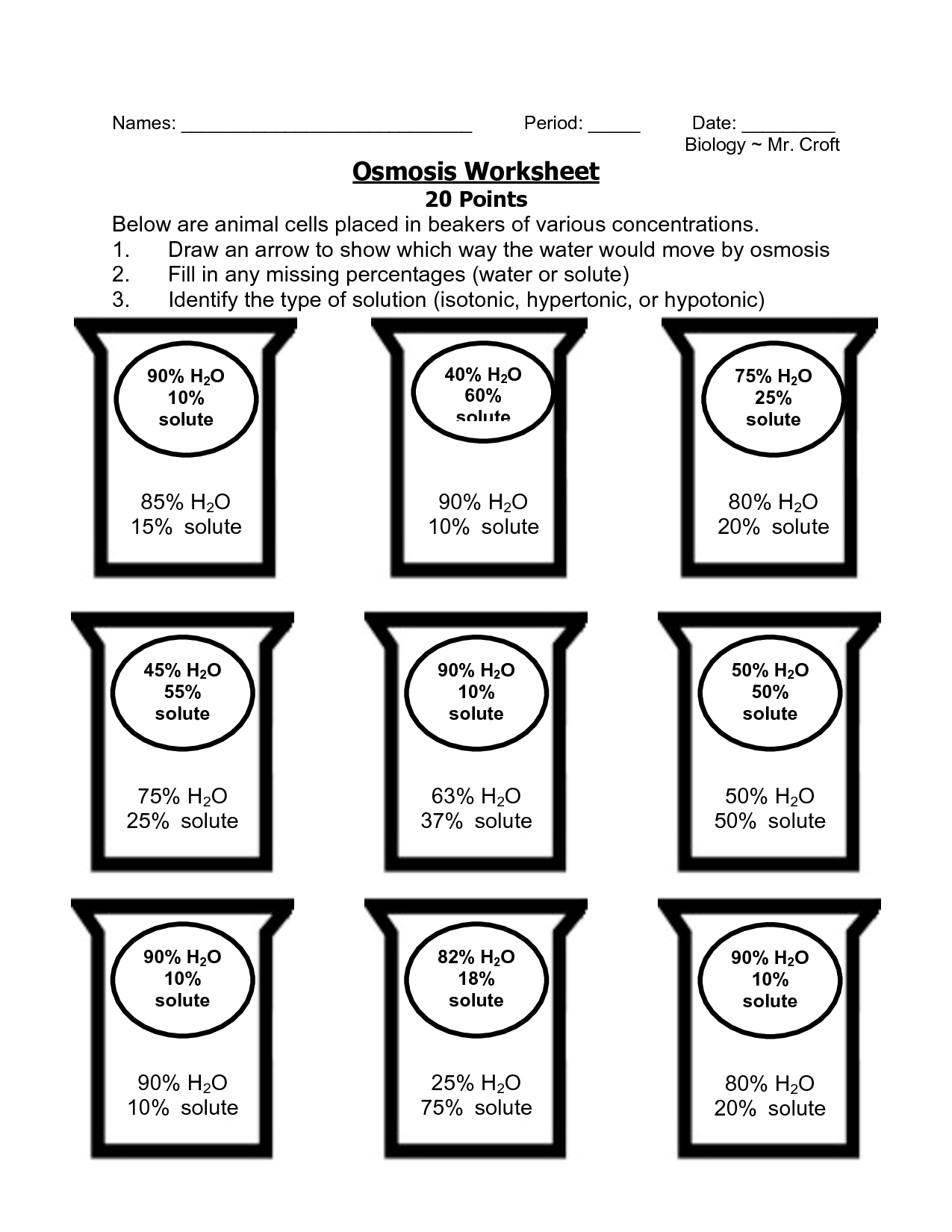
5 Tips for Mastering One-Step and Two-Step Equations

One-step and two-step equations form the foundation of algebra. They are not only essential for academic success in math but also for understanding and solving real-world problems. Whether you're helping someone with homework, catching up on basics, or just wanting to brush up on your math skills, mastering these equations is crucial. Here's how you can become adept at solving them:
Understand the Basics

Before diving into the mechanics of solving equations, it’s imperative to grasp the fundamental concepts:
- Equation: An equation is a statement of equality between two expressions involving variables and constants.
- Variable: A symbol, usually a letter, representing an unknown value or a quantity that can change.
- Constant: A fixed value that does not change.
Isolate the Variable: One-Step Equations

One-step equations involve performing a single operation to isolate the variable. Here are the basic steps:
- If the equation involves addition or subtraction, use inverse operations to move the constant to the opposite side.
- If the equation involves multiplication or division, do the same by dividing or multiplying both sides by the coefficient of the variable.
Example:
- Addition: x + 5 = 10 (subtract 5 from both sides)
- Multiplication: 3x = 27 (divide both sides by 3)
Combine Operations: Two-Step Equations
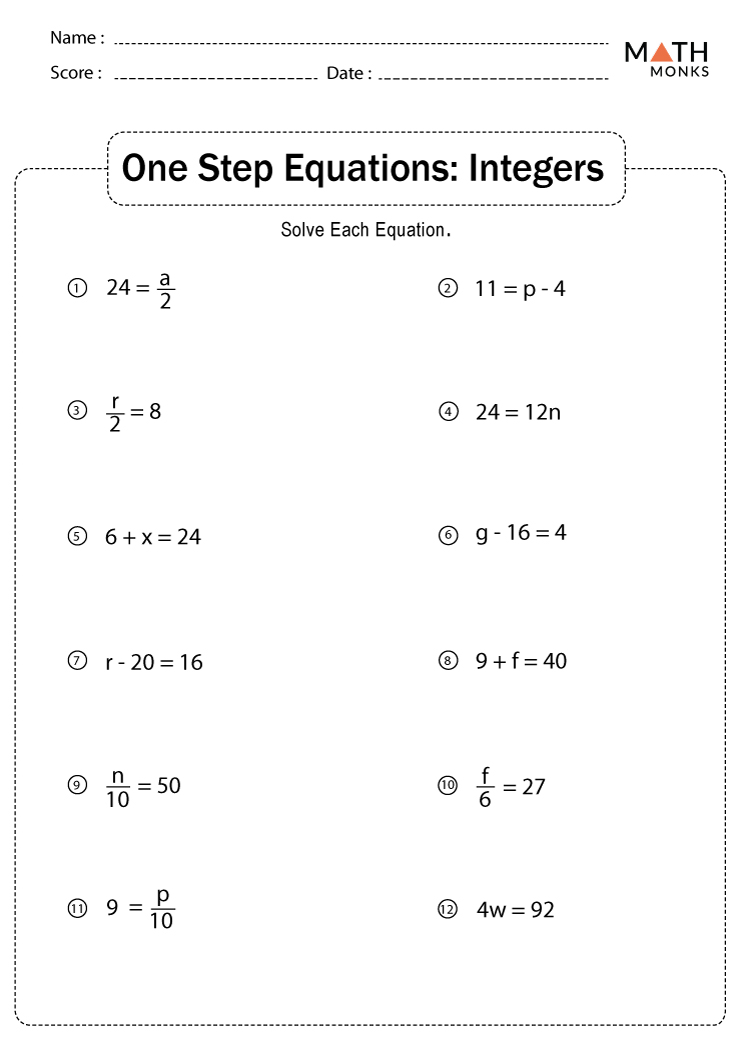
Two-step equations are slightly more complex and require you to apply two operations in sequence:
- First, deal with any addition or subtraction to isolate the term with the variable.
- Then, use multiplication or division to get the variable by itself.
Example:
- Two Steps: 2x + 3 = 9
- Subtract 3 from both sides: 2x + 3 - 3 = 9 - 3
- Divide by 2: (2x/2) = 6/2
Practice Consistency

Consistency in applying steps is key to mastering equations. Here's how you can ensure consistency:
- Always follow the same sequence: For one-step equations, always apply the inverse operation directly. For two-step equations, handle constants first, then coefficients.
- Check your work: Substitute your solution back into the original equation to verify it's correct.
🔎 Note: For more complex equations, the principles of isolating the variable remain the same but involve more steps.
Use Visual Aids and Tools

Visual aids can significantly help in understanding the balance of equations:
- Use balance scales to illustrate how operations affect both sides of an equation.
- Number lines can visually represent the addition or subtraction of values.
Key Strategies for Practice:
- Practice sets of one-step and two-step equations separately to master each type.
- Create flashcards with equations on one side and the steps to solve on the other.
Real-World Applications

Connecting equations to real-life scenarios helps solidify their utility:
- When shopping, think about how much money you'd need if something was discounted by a certain percentage.
- Understand banking by solving simple interest rate problems or determining the necessary budget adjustments.
📚 Note: Real-life problems might not always appear in standard equation form, but the principles learned from one-step and two-step equations can be applied to decode them.
Embrace the Journey of Learning

Learning equations involves:
- Patience: Each equation solved adds to your skillset.
- Persistence: Continue practicing even when it feels challenging.
- Perspective: View mistakes as learning opportunities.
Understanding and mastering one-step and two-step equations is not merely about solving math problems. It's about developing a systematic approach to problem-solving that can be applied in numerous aspects of life. By embracing the foundational techniques, using visual aids, and connecting equations to real-world scenarios, you'll not only enhance your algebra skills but also your ability to think logically and solve problems effectively. Keep in mind, the path to proficiency involves consistency, practice, and a keen eye for detail. Whether it's ensuring you isolate the variable correctly or understanding the underlying principles, the journey of mastering equations is about both the learning and the application of math in everyday life. As you continue this journey, you'll find that equations become second nature, and complex problems become simpler, one step at a time.
Why is isolating the variable important in solving equations?
+
Isolating the variable is crucial because it allows you to find the unknown value that makes the equation true. By isolating the variable on one side of the equation, you can easily solve for it by equating the other side.
How can I remember which operations to use in two-step equations?

+
Always remember to deal with addition/subtraction first to get to the variable. Then, address multiplication/division. A mnemonic you can use is “A-S M-D” where A and S refer to addition and subtraction, and M and D refer to multiplication and division.
What are some common mistakes when solving one-step or two-step equations?

+
Common mistakes include not maintaining balance by applying the same operation to both sides of the equation, confusion with negative signs, or incorrect division/multiplication when isolating the variable. Always check your solution by substituting it back into the original equation.