5 Steps to Master Multiplying Mixed Numbers Easily

Multiplying mixed numbers can seem daunting at first, but with the right approach, it can become second nature. Mastering this operation not only enhances your mathematical skills but also boosts your confidence in tackling complex number problems. Here are five easy steps to ensure you can multiply mixed numbers without breaking a sweat.
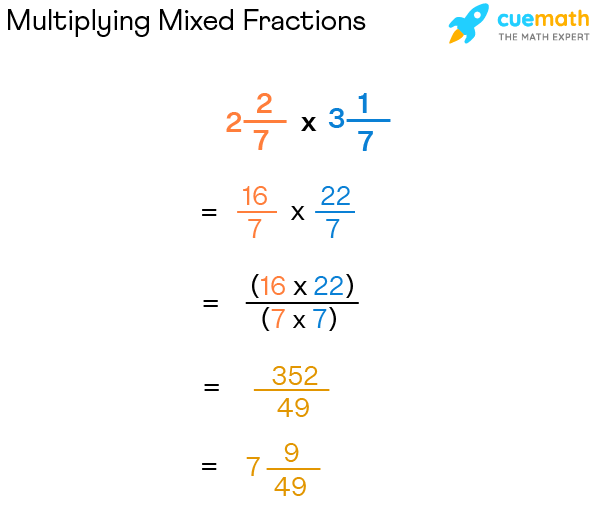
1. Convert Mixed Numbers to Improper Fractions

The first step in multiplying mixed numbers is to convert them into improper fractions. A mixed number is a number that combines a whole number and a fraction. Here’s how to convert:
- Multiply the whole number by the denominator of the fraction.
- Add the numerator to the result from above.
- Place this sum over the original denominator to form an improper fraction.
For example:
- If you have 2 3⁄4, first, multiply 2 by 4 (which gives 8). Add the numerator 3 (which totals 11). So, 2 3⁄4 becomes 11⁄4.
2. Multiply the Improper Fractions

Once you have your improper fractions, you can proceed with the multiplication:
- Multiply the numerators of the two fractions to get the new numerator.
- Multiply the denominators to get the new denominator.
For instance, if you’re multiplying 5⁄2 by 3⁄4:
- The new numerator is 5 * 3 = 15.
- The new denominator is 2 * 4 = 8.
So, 5⁄2 * 3⁄4 = 15⁄8.
3. Simplify the Result if Necessary

After multiplying, your result might be in an improper fraction form. Here’s what you can do:
- If the resulting fraction is improper (top-heavy), convert it back to a mixed number.
- To convert, divide the numerator by the denominator. The quotient will be the whole number, and the remainder will become the new numerator, keeping the original denominator.
For 15⁄8:
- 15 divided by 8 gives 1 as the whole number with a remainder of 7.
- The result is 1 7⁄8.
⚠️ Note: When simplifying, ensure to look for common factors between the numerator and the denominator for the simplest form.
4. Practice with Multiple Examples

Multiplying mixed numbers becomes easier with practice. Here’s how you can practice:
- Start with simple mixed numbers where both the whole part and the fractional part are small.
- Gradually increase the complexity by mixing whole numbers, mixed numbers, and improper fractions in your exercises.
- Use real-life scenarios or story problems to make multiplication more engaging and practical.
5. Review and Understand Errors

Understanding your mistakes is crucial:
- After solving problems, go over them again.
- Identify where you might have gone wrong in conversion or multiplication.
- Learn from these mistakes to prevent them in future calculations.
This step ensures a deeper understanding and better application of the concepts.
By following these steps consistently, you can master multiplying mixed numbers with ease. Each step builds on the previous one, creating a structured approach to problem-solving that not only makes multiplication simpler but also improves your overall mathematical competence. As you refine your skills, you'll find that these operations become more intuitive, allowing you to tackle more complex problems with confidence.
What is the benefit of converting mixed numbers to improper fractions before multiplying?
+
Converting mixed numbers to improper fractions makes multiplication easier because you deal with whole numbers and fractions separately, simplifying the process.
How can I tell if my answer needs simplification?

+
If your result is an improper fraction or if the numerator and denominator share common factors other than 1, simplification is needed.
Can these steps be used for division of mixed numbers?

+
Yes, but for division, you’ll need to convert one fraction into its reciprocal before multiplying.



