Master Multiplying by Multiples of 10 Easily

In the realm of basic arithmetic, multiplying by multiples of 10 stands out as one of the easiest and most intuitive skills to learn. Understanding this concept not only simplifies calculations but also lays the groundwork for mastering higher mathematics. This skill is essential for everyday tasks, from shopping and financial planning to engineering calculations and beyond. Today, we'll explore how you can effortlessly multiply numbers by 10, 100, 1000, and so on, ensuring you feel confident in your mathematical abilities.
Understanding the Concept
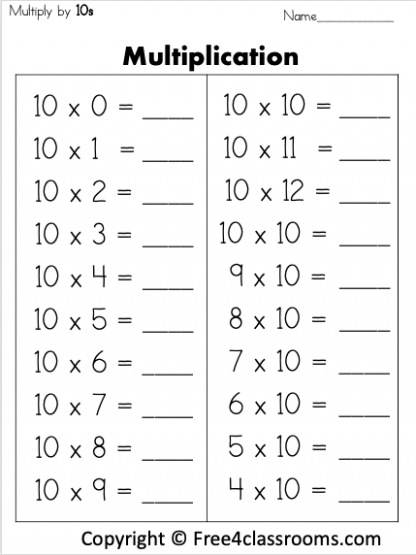
To truly grasp how to multiply by multiples of 10, let’s first understand the basic concept. When you multiply a number by 10, you are essentially adding one zero to the end of that number. This rule applies across the board, whether you’re multiplying by 10, 100, 1000, or any other multiple of 10:
- Multiplying by 10 adds one zero.
- Multiplying by 100 adds two zeros.
- Multiplying by 1000 adds three zeros.
Here’s an example to illustrate:
- 23 x 10 = 230
- 23 x 100 = 2300
- 23 x 1000 = 23000
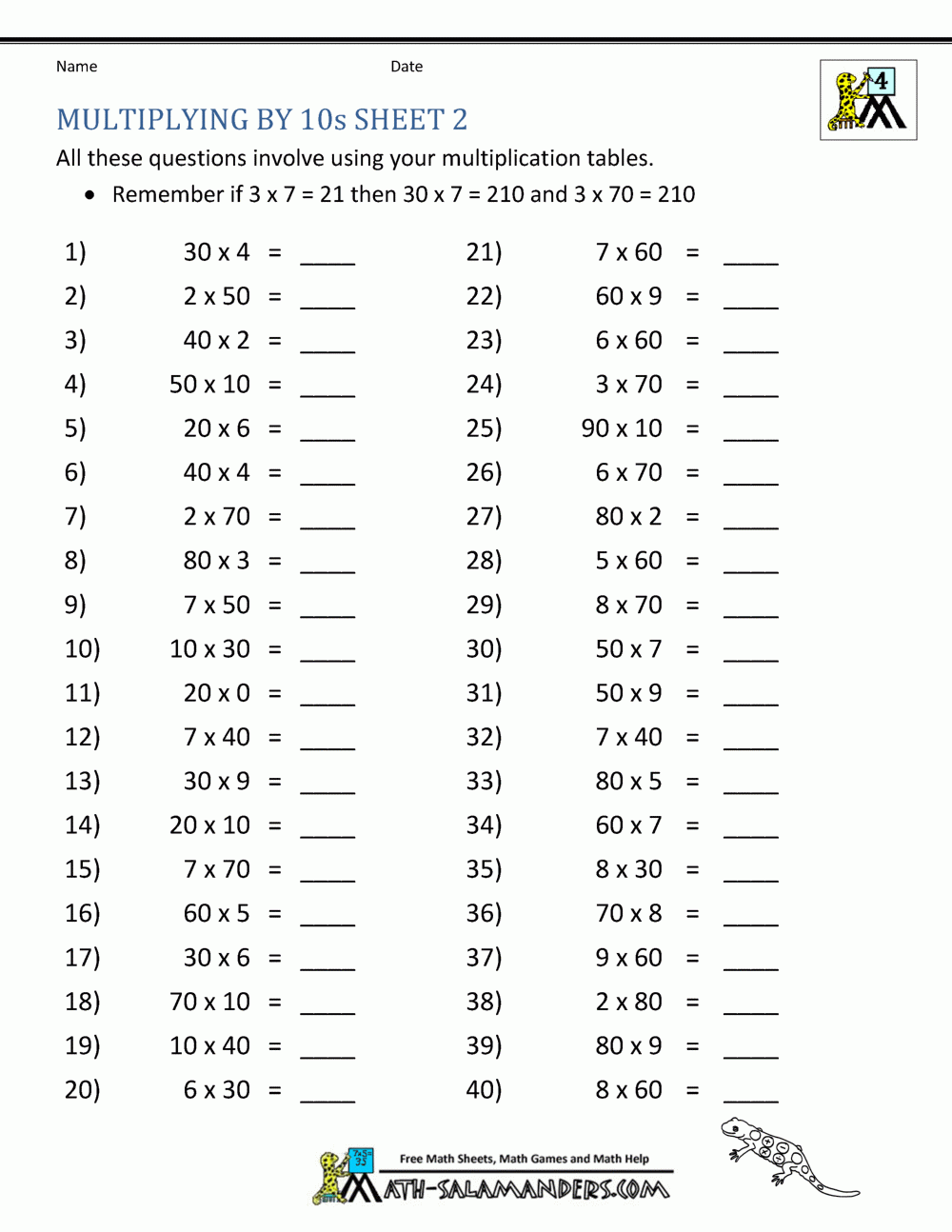
When the number you're multiplying has zeros at the end, you simply shift the digits to the left, then add the appropriate number of zeros:
- 230 x 100 = 23,000 (two shifts to the left, then add two zeros)
Steps to Multiply by Multiples of 10

Follow these steps to ensure you're multiplying correctly:
- Identify the Number of Zeros: Determine how many zeros the multiple of 10 has.
- Shift the Digits: If your base number has zeros, shift the non-zero digits to the left by the number of zeros in your multiplier.
- Add Zeros: Append the same number of zeros to the result as your multiplier has.
- Verify: Double-check your work to ensure accuracy.
Real-World Applications

Understanding this multiplication technique has several practical applications:
- Shopping: When shopping, you often need to calculate the total cost when buying items in bulk. For instance, if you want to buy 3 packs of cookies, each priced at $10, you can quickly determine the cost as $30.
- Financial Calculations: Converting dollars to cents or vice versa becomes a straightforward task. $1 becomes 100 cents, and $10 becomes 1000 cents.
- Measurements: In scientific notation or measurements, you can convert units by multiplying by powers of 10. For example, 1 meter is 100 centimeters, and 1 kilometer is 1000 meters.
Tips for Easy Memorization

To make multiplication by multiples of 10 even easier:
- Practice Regularly: Like any skill, regular practice will enhance your speed and accuracy.
- Use Visual Aids: Consider drawing place value charts or using number blocks to visualize the addition of zeros.
- Relate to Real Life: Link the multiplication to everyday situations, making it more memorable.
- Teach Others: Explaining the concept to someone else can reinforce your understanding.
Now, as we wrap up our journey into the world of multiplying by multiples of 10, we have seen how this fundamental math skill can simplify a wide array of calculations in both personal and professional life. The principle of adding zeros, shifting digits, and applying this technique to real-life scenarios ensures that you can manage numbers effortlessly, making complex problems seem much simpler. This skill, though basic, is the foundation upon which more advanced mathematical concepts are built. So, the next time you encounter a multiplication problem involving tens or hundreds, remember these steps, practice with real-world examples, and let your newfound knowledge shine through your calculations.
What is the difference between multiplying by 10 and 100?

+
The difference is in the number of zeros added to the end of the number you are multiplying. Multiplying by 10 adds one zero, while multiplying by 100 adds two zeros.
Can this method be applied to any number?

+
Yes, this method can be applied to any whole number, decimal, or fraction. However, for decimals, you must ensure the placement of the decimal point is correct after multiplication.
How does this relate to scientific notation?
+
Scientific notation often involves expressing numbers as multiples of 10 raised to some power. Understanding how to multiply by powers of 10 helps you easily convert numbers between standard and scientific notation.