5 Essential Steps to Solving Rational Expressions Easily

Understanding rational expressions can be a daunting task for many students. These expressions, which involve fractions where the numerator and the denominator are polynomials, play a critical role in algebra and beyond. However, with the right strategies and knowledge, solving rational expressions can become straightforward. Here are five essential steps to master solving rational expressions easily.
Identify the Parts

First, identify the key components of the rational expression:
- Numerator: The polynomial above the division line.
- Denominator: The polynomial below the division line.
By recognizing these parts, you can better understand the structure of the rational expression and how to manipulate it.
Simplify the Expression

After identifying the parts, simplify the rational expression:
- Factor both the numerator and denominator. Look for the greatest common factor (GCF) or use methods like grouping or special product forms.
- Cancel out common factors. Any factor that appears in both the numerator and denominator can be canceled out, as long as it does not cancel to zero.
- Combine like terms if possible.
🔍 Note: Remember that when simplifying, you can only cancel factors, not individual terms or constants.
Handle Complex Fractions

If you encounter a complex fraction (a fraction within a fraction), follow these steps:
- Convert each part into its reciprocal if necessary, to make both parts into improper fractions.
- Apply the rule of multiplying by the reciprocal to simplify. For example, if you have (\frac{a}{b} \div \frac{c}{d}), you can simplify it by multiplying (\frac{a}{b}) by (\frac{d}{c}).
- Combine or simplify further if possible.
Perform Operations

Sometimes, you’ll need to perform basic operations like addition, subtraction, multiplication, or division with rational expressions:
- Addition/Subtraction: Find a common denominator, then add or subtract the numerators while keeping the denominator the same.
- Multiplication: Multiply the numerators together and then the denominators together. Simplify the result.
- Division: Divide by the reciprocal, then simplify as with multiplication.
Check for Restrictions

Finally, check for restrictions on the variables in your rational expression:
- Identify the values of the variable that make the denominator zero, as these values are not allowed (the expression is undefined).
- State these restrictions clearly to complete your solution or to avoid errors in further calculations.
These steps provide a structured approach to handling rational expressions, making them less intimidating and more manageable. By focusing on identifying parts, simplifying, handling complex fractions, performing operations, and checking for restrictions, you'll find that you can solve a wide array of problems involving rational expressions with ease.
A Real-World Application

Let’s consider a practical example. Suppose you’re an engineer designing a circuit, and you need to calculate the resistance (R) of two resistors connected in parallel. The formula is:
[ \frac{1}{R} = \frac{1}{R_1} + \frac{1}{R_2} ]
Here's how you would solve this problem using our steps:
- Identify: Numerator = 1, Denominators = R_1 and R_2.
- Simplify: Factor the sum on the right side to get \frac{R_1 + R_2}{R_1R_2}.
- Handle Complex Fractions: Not necessary in this case, but if you were to combine multiple resistors, you'd follow this step.
- Perform Operations: Cross multiply to solve for R, leading to R = \frac{R_1R_2}{R_1 + R_2}.
- Check for Restrictions: R_1 and R_2 cannot be zero.
This example shows how rational expressions are not only relevant in algebra but also have practical applications in fields like engineering, physics, and economics.
Mastering these steps will give you the tools to tackle various mathematical problems and apply them to real-world scenarios. With practice, rational expressions become less about complexity and more about methodical problem-solving.
Why is it important to simplify rational expressions?

+
Simplifying rational expressions helps reduce the complexity of the equation, making it easier to solve and understand. It also reveals the underlying structure of the expression, which can be crucial for further algebraic manipulations.
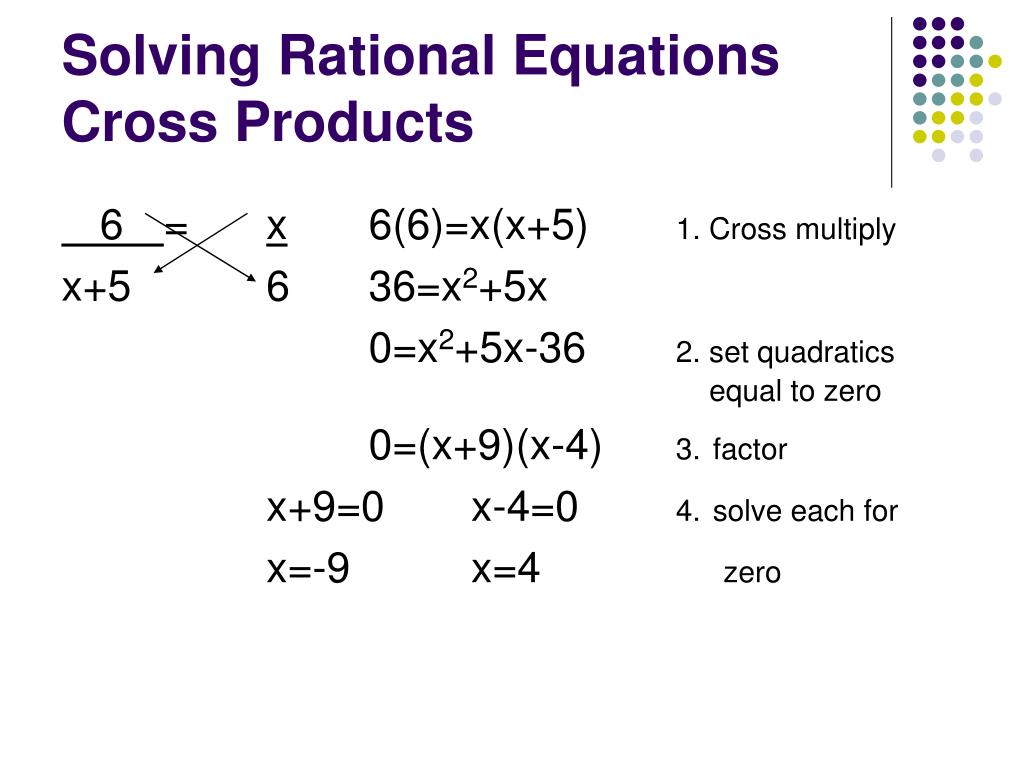
What should I do if I encounter a common factor in both numerator and denominator?
+
You should cancel the common factor. However, ensure that the factor does not lead to a zero in the denominator, which would make the expression undefined for that value of the variable.
How do restrictions on variables affect the solution of a rational expression?

+
Restrictions indicate values that the variable cannot take, as these would make the denominator zero and thus the expression undefined. Understanding these restrictions helps in ensuring the solution is valid for all permissible values of the variable.
Can you perform operations like addition or subtraction with rational expressions?

+
Yes, by finding a common denominator and then combining the numerators. This process often involves factoring and simplifying to maintain the simplest form of the expression.