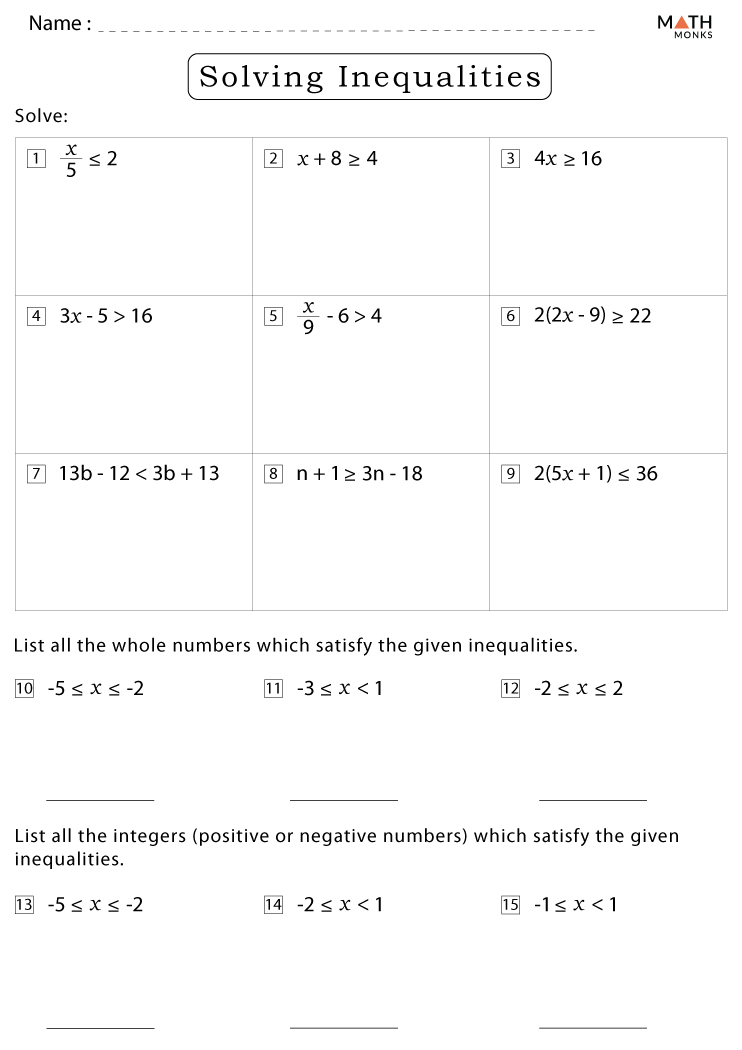
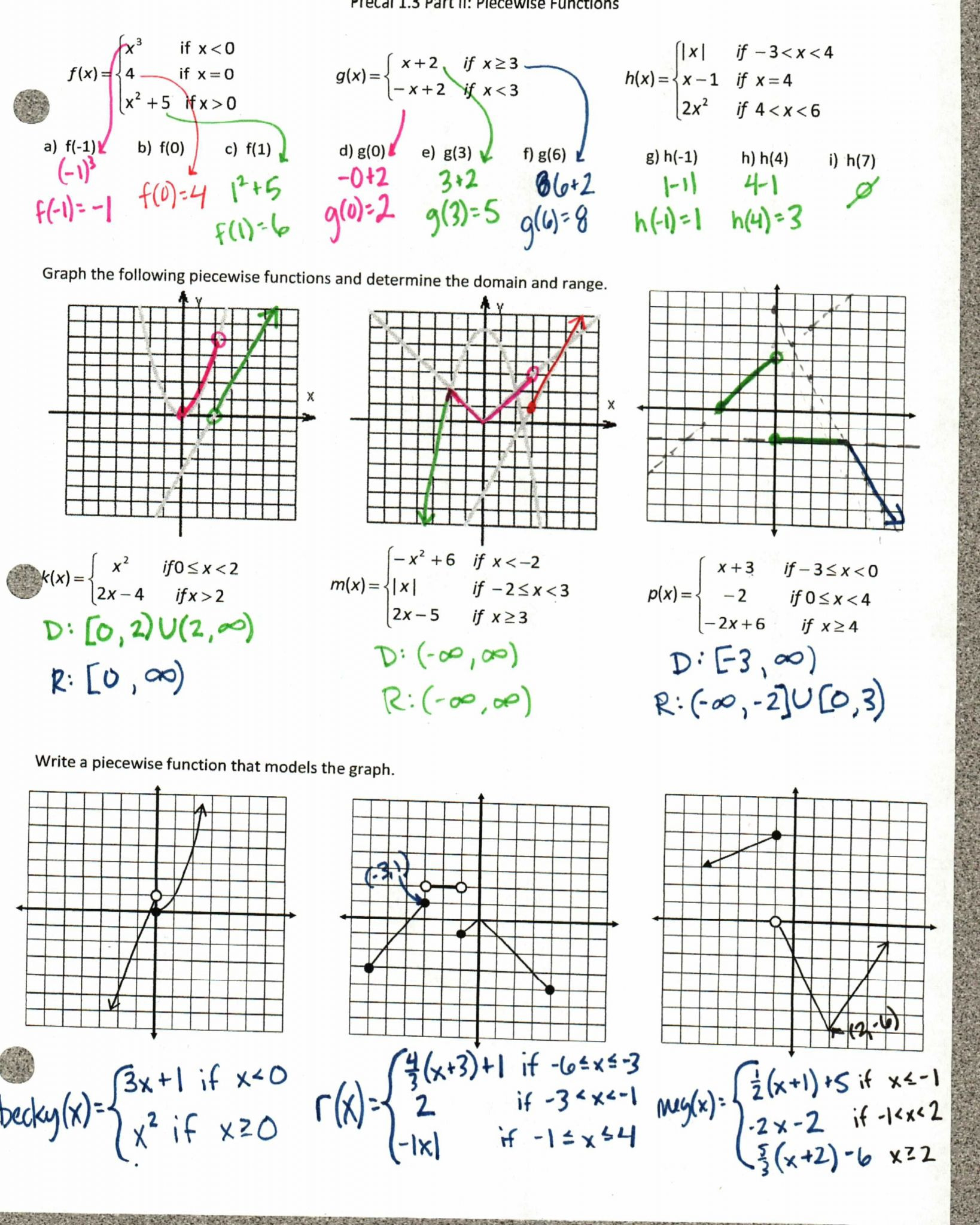
5 Key Features of Function Graphs Worksheet Answers

In today's educational landscape, understanding the intricacies of mathematical functions through graphing is pivotal for student success in advanced math courses. Function graphs provide an intuitive visual representation, making abstract concepts more tangible. Here, we delve into five key features of function graphs worksheet answers, designed to empower students with comprehensive insights into functions and their behaviors.
The Role of Function Graphs in Learning
Function graphs are more than just lines or curves on a grid; they are essential tools for visualizing:
- The relationships between variables.
- The behavior of functions over specific intervals.
- The effects of different transformations and parameter changes on functions.
This understanding equips students with the visual and analytical skills necessary to excel in algebra, calculus, and beyond.

1. Identifying Key Points

Every function has defining points like:
- Intercepts: Where the graph intersects the x-axis or y-axis.
- Turning Points: Local maxima or minima where the slope changes from increasing to decreasing or vice versa.
- Discontinuities: Points or intervals where the function is not continuous.
Correctly identifying these points helps students understand the function’s domain, range, and overall behavior. For instance, if the graph has no turning points, it suggests the function is monotonic over its domain.
🔍 Note: Always check for symmetry in functions; symmetry about the y-axis or origin can simplify understanding and working with the function.
2. Analyzing Function Transformations

The manipulation of function graphs through transformations is a fundamental concept:
- Horizontal Shifts: ( f(x) \rightarrow f(x + c) ) where ( c ) shifts the function left or right.
- Vertical Shifts: ( f(x) \rightarrow f(x) + c ) where ( c ) shifts the function up or down.
- Reflections: Across the x-axis or y-axis.
- Stretches/Compressions: Vertical or horizontal scaling of the graph.
Understanding these transformations helps students predict how different modifications to the function’s formula will affect the graph. This predictive understanding is crucial for modeling real-world phenomena.
3. Determining Function Continuity

The continuity of a function graph can significantly impact its usability in mathematical and scientific applications:
- Continuous functions have no gaps or jumps.
- Discontinuous functions can exhibit jumps, holes, or vertical asymptotes.
Worksheet answers should explain why a function is or isn’t continuous at certain points, offering insights into potential modeling limitations or areas where the function might require piecewise definitions.
4. Asymptotes and End Behavior

As students delve into more complex functions, understanding asymptotes and end behavior becomes essential:
- Vertical Asymptotes: Where the function approaches positive or negative infinity at specific x-values.
- Horizontal Asymptotes: Where the function approaches a specific y-value as ( x ) approaches positive or negative infinity.
- End Behavior: The function’s behavior as ( x ) moves toward the extremes of the number line.
These features indicate where functions might be undefined, how they relate to real-world scenarios, and how they behave as inputs increase or decrease indefinitely.
📚 Note: Understanding the relationship between polynomial degrees and the number of turning points can help predict the function's behavior at extremes.
5. Relating Graph Features to Function Characteristics

Connecting the visual representation of a function to its algebraic definition requires:
- Domain and Range: Identifying the set of all possible inputs and outputs.
- Rate of Change: Analyzing how the function changes over specific intervals.
- Zeros or Roots: Points where the function equals zero, corresponding to x-intercepts.
This synthesis allows for deeper insight into the function’s nature, making it easier to solve equations, inequalities, and interpret real-life scenarios involving those functions.
To wrap up our exploration of function graphs worksheet answers, these five key features offer students a comprehensive approach to analyzing and understanding functions. By mastering these aspects, students not only excel in their current studies but also develop a toolkit that will be invaluable in higher mathematics, science, and engineering disciplines. Function graphs are not merely visual aids but powerful tools for problem-solving and critical thinking, fostering a deeper appreciation for the intricate beauty of mathematics.
What are the different types of functions commonly graphed?

+
Commonly graphed functions include linear, quadratic, polynomial, rational, exponential, logarithmic, and trigonometric functions. Each type has unique characteristics in terms of shape, symmetry, and growth patterns.
Why is understanding function graphs important?

+
Function graphs provide a visual representation that helps understand the behavior of variables, model real-world scenarios, solve equations, and develop intuition about complex mathematical concepts.
How can students practice identifying function transformations?

+
Students can practice by systematically altering the basic forms of functions (like ( f(x) )) through shifts, reflections, and stretches, then observing the changes on the graph. Interactive software or graphing calculators are great tools for this practice.
What’s the importance of knowing the function’s continuity?

+
Continuity informs where the function can be applied in mathematical modeling, how it behaves near certain points, and if it has a continuous derivative. Understanding discontinuities helps students deal with real-world problems where phenomena can have abrupt changes or limitations.
Can graphing functions help with understanding calculus?

+
Absolutely. Graphing functions directly relates to concepts like derivatives, integrals, limits, and optimization problems. It provides a visual context that supports the mathematical abstraction found in calculus.