Graphing Lines vs Zombies: A Math Challenge
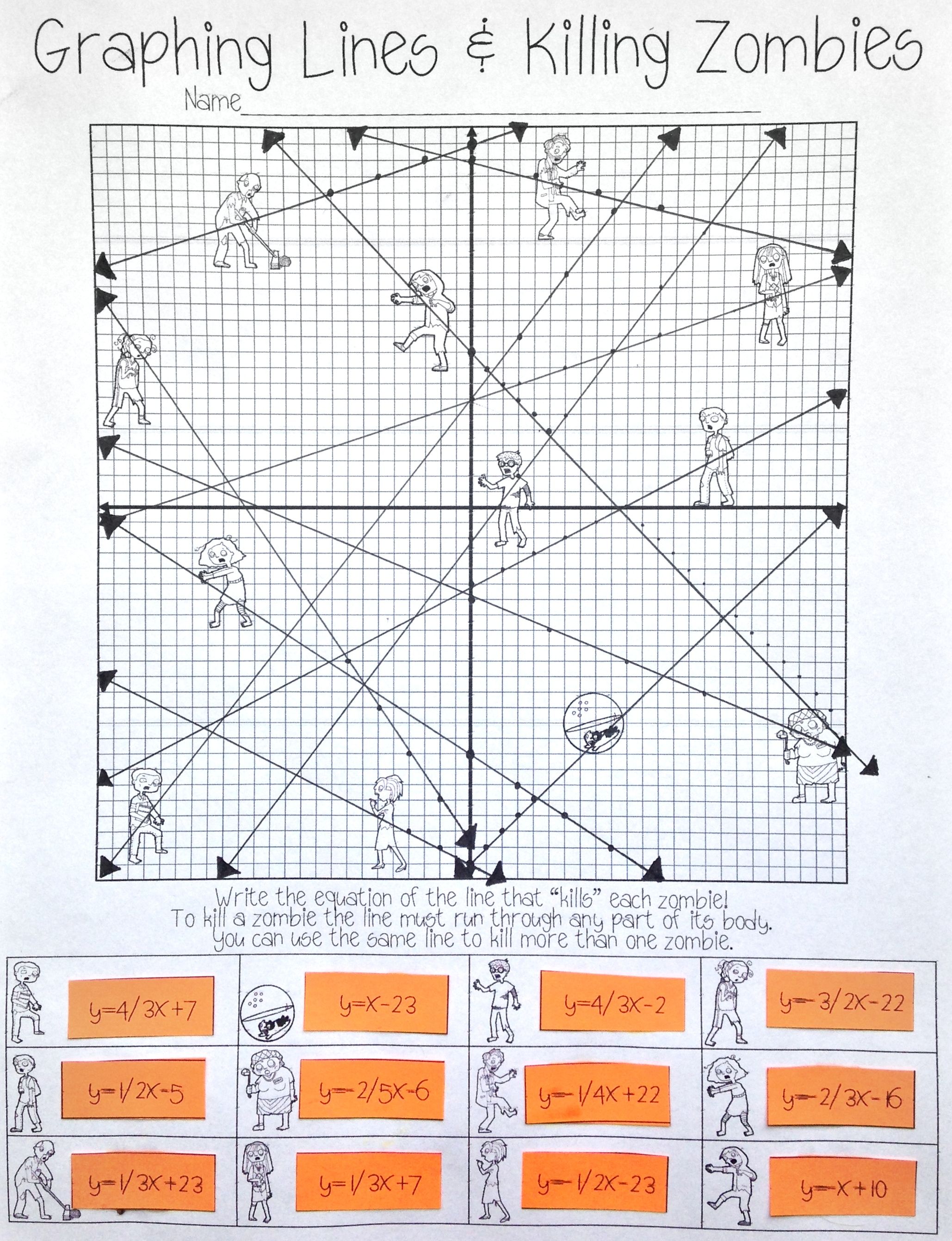
In the world of mathematics, there's always a creative way to make learning fun. One such engaging method is turning mathematical challenges into games. Today, we delve into the mathematical game known as "Graphing Lines vs Zombies," where players use their understanding of linear equations to keep the zombies at bay. Here's an in-depth look at this unique math challenge.
What is “Graphing Lines vs Zombies”?

Graphing Lines vs Zombies is an educational game that uses the concept of graphing linear equations to engage students in mathematics. The premise is simple: you must graph lines to protect a house from an incoming horde of zombies. Each line you graph serves as a barrier, and if your math is correct, you’ll keep the undead from reaching their target.
- Gameplay: Players enter equations, and the lines they form create barriers.
- Goal: Survive as long as possible by preventing zombies from crossing your lines.
- Learning Objective: Understand the relationship between slope, intercept, and equation form.

Why This Game?
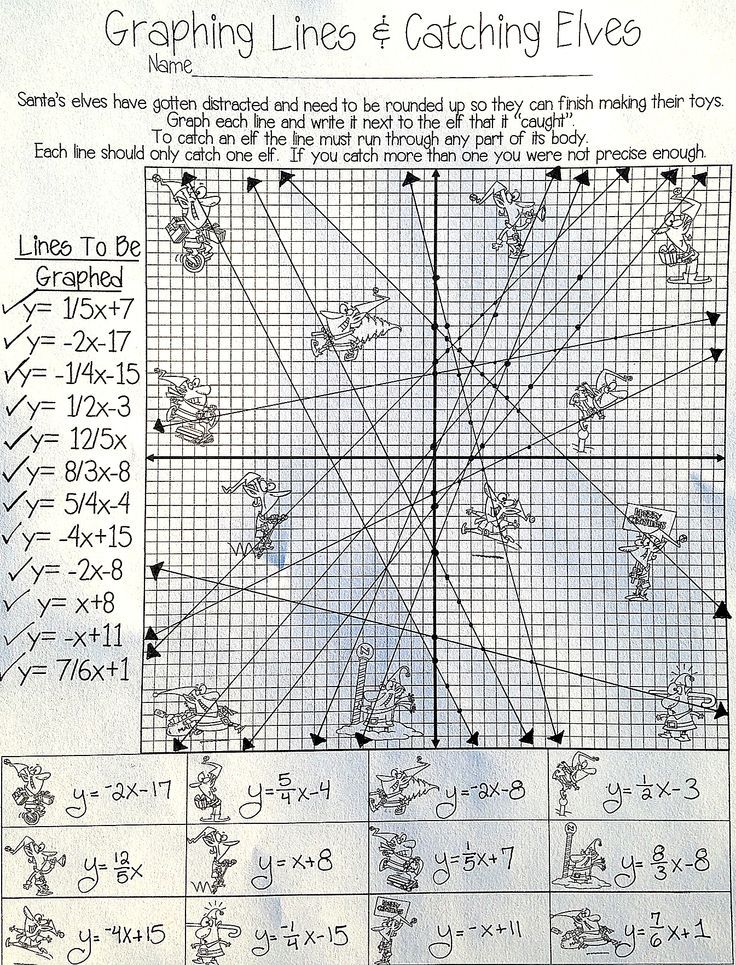
There are several compelling reasons why teachers and students should consider playing “Graphing Lines vs Zombies”:
- Real-World Application: It demonstrates how math can be used in a fun, game-like setting, showing that numbers and equations have practical uses.
- Interactive Learning: The game encourages students to actively think about the results of their equations rather than passively learn formulas.
- Critical Thinking: Players need to strategize which lines will stop the most zombies, thus fostering problem-solving skills.
Understanding the Mathematics Behind the Game
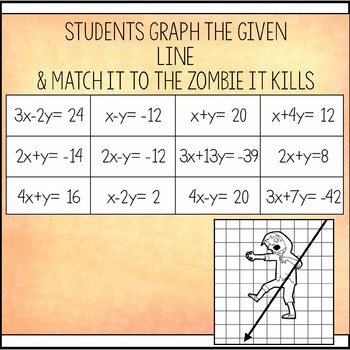
Let’s explore the core mathematical concepts players engage with during the game:
- Slope-Intercept Form: The most common form of linear equation, y = mx + b, where:
- m is the slope, which determines the angle of the line
- b is the y-intercept, where the line crosses the y-axis
- Intercepts: Understanding how to find x and y intercepts helps in deciding where to place lines for maximum protection.
- Parallel and Perpendicular Lines: Players must understand that parallel lines have equal slopes, and perpendicular lines have negative reciprocal slopes.
| Equation Type | Example | Description |
|---|---|---|
| Vertical Line | x = 2 | A line with undefined slope |
| Horizontal Line | y = -3 | A line with a slope of 0 |
| Standard Line | y = 2x + 3 | A typical linear equation in slope-intercept form |

📚 Note: The game allows for more complex equations once players master the basics, encouraging exploration of other forms like point-slope or standard form.
Strategies for Winning at “Graphing Lines vs Zombies”
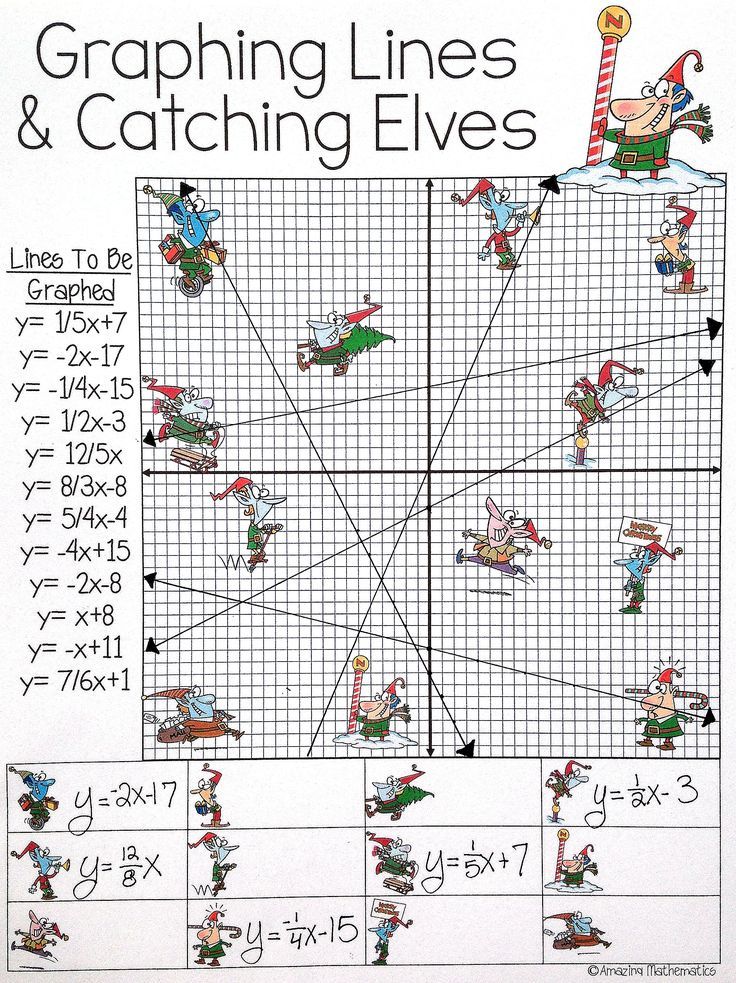
Here are some tactics to consider when playing:
- Strategic Placement: Position your lines to block the most zombies with the least number of equations. Think about which directions the zombies are moving.
- Intersecting Points: Consider the coordinates where your lines should intersect to maximize coverage.
- Varied Slopes: Use different slopes to create multiple barriers at varying angles, making it harder for zombies to bypass your defense.
- Zombie Movement: Anticipate the zombies’ movement patterns. Zombies often follow certain paths or have predictable speeds, which can be used to your advantage.
Enhancing Gameplay with Advanced Concepts
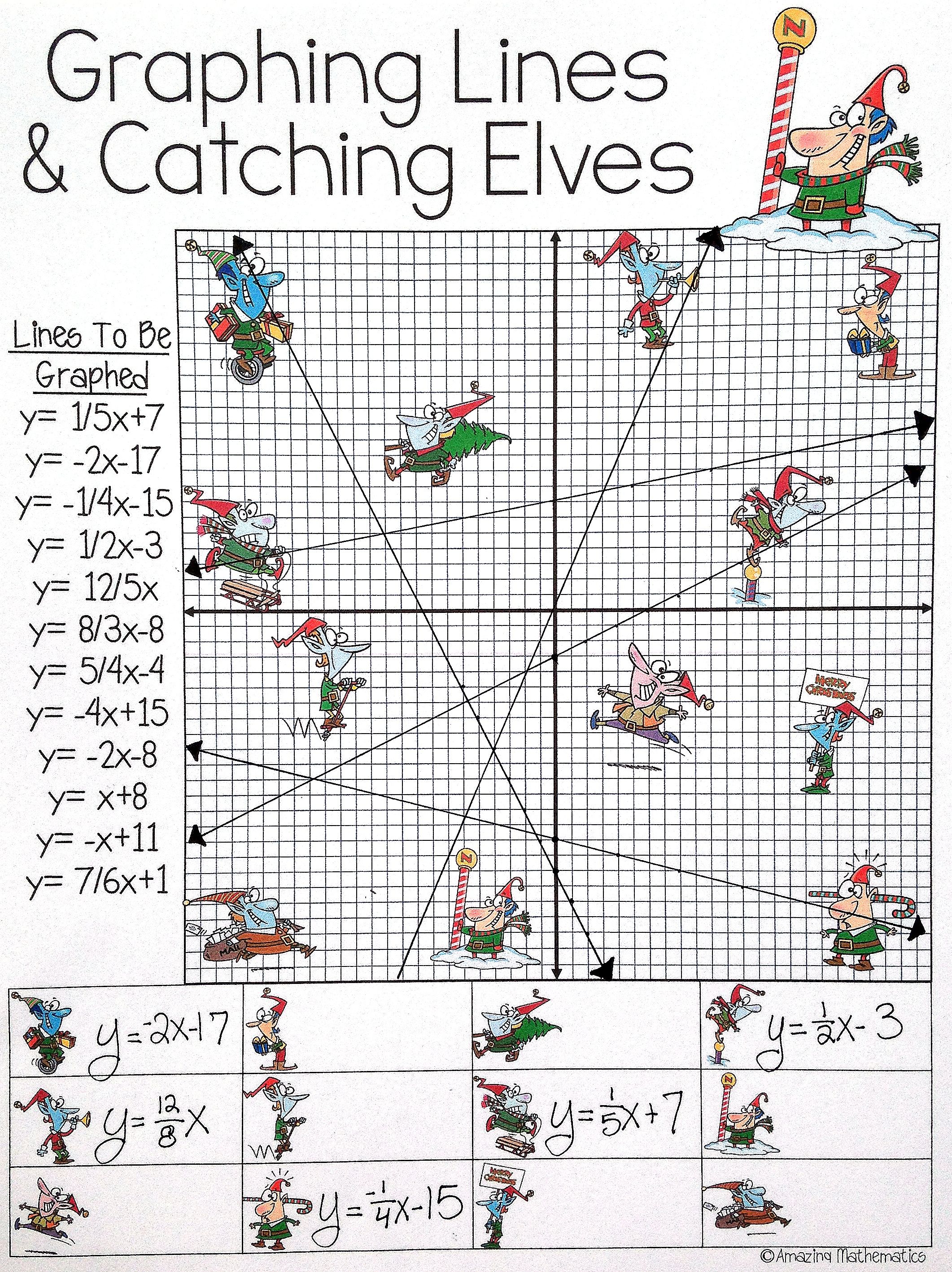
As players become more adept at the game, here are some ways to incorporate more advanced math:
- Systems of Equations: Use intersecting lines to create ‘funnels’ that force zombies into kill zones.
- Dynamic Equations: Change the equation parameters to adjust your lines in real-time, responding to incoming threats.
- Trigonometry: If zombies follow an arc, understanding sine and cosine could help in creating barriers that match their trajectories.
Impact on Math Education

“Graphing Lines vs Zombies” isn’t just a game; it’s a tool for enhancing math education:
- Student Engagement: Games like this can make learning feel less like work and more like play, improving retention.
- Visual Learning: Seeing the equations in action helps visual learners grasp abstract concepts.
- Immediate Feedback: Correct answers fortify the house, while incorrect ones lead to zombie invasion, providing instant feedback on understanding.
To sum up, "Graphing Lines vs Zombies" is an innovative way to engage students with math, proving that even the most abstract of subjects can be made exciting and tangible. By transforming equations into zombie-blocking barriers, this game turns the sometimes dull task of learning algebra into an engaging challenge that involves strategy, foresight, and quick mathematical thinking. Through this unique method, we can see how interactive and dynamic approaches to education can significantly enhance the learning experience, making abstract concepts not only understandable but also enjoyable to explore.
Can this game be used for teaching older students?

+
Yes, the game can be scaled up to include more advanced math concepts, suitable for high school or even college-level math courses.
Is this game available online or for download?

+
Information on availability can be found by searching “Graphing Lines vs Zombies” on educational game platforms. It’s often part of math resource libraries accessible through schools or educational subscriptions.
What age group is this game designed for?

+
The game is primarily aimed at middle school students, but with different levels, it can be adjusted for younger or older learners.
How can teachers incorporate this into their curriculum?

+
Teachers can incorporate the game into units on linear equations, using it as a practical application activity to reinforce learned concepts. It can also be part of math game days or used as a reward or incentive for completing math assignments.
Does this game support multiplayer or cooperative play?
+
The current versions are mainly single-player focused, but some educational platforms might offer multiplayer versions or challenges for collaborative learning.