Geometry Trig Word Problems: Worksheet Answers Explained
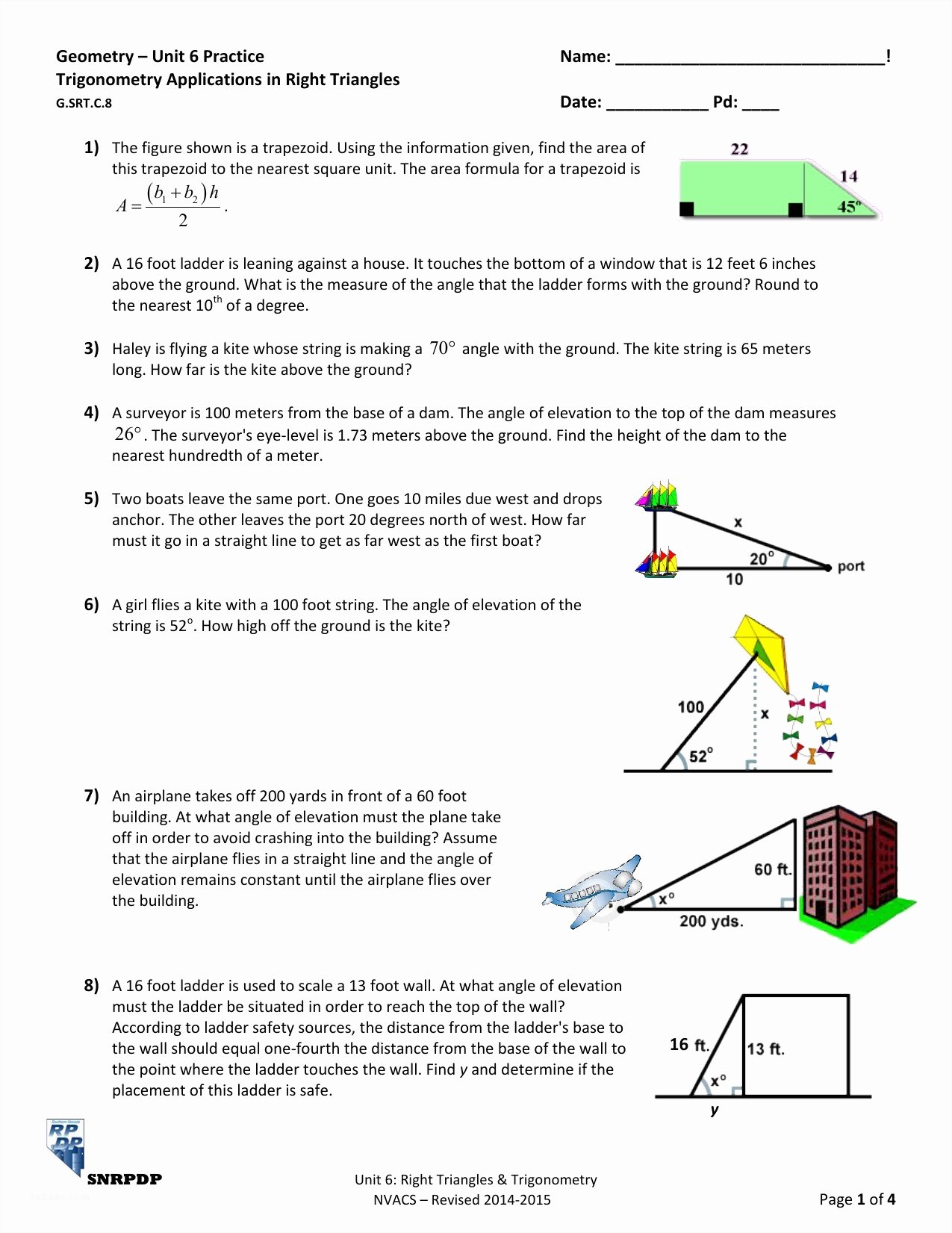
The realm of trigonometry offers a unique challenge and satisfaction, especially when applied to real-life scenarios. Geometry and trigonometry together can help you solve complex problems involving angles, distances, and heights. This comprehensive guide will walk you through some typical word problems, providing detailed solutions and insights into the Geometry Trig Word Problems. Whether you're a student looking to enhance your problem-solving skills or an enthusiast keen on understanding how trigonometry shapes our world, let's dive into the applications of trigonometry in geometric contexts.
Understanding Trigonometric Ratios

Before we delve into the problems, it's crucial to understand the three basic trigonometric ratios:
- Sine (sin): Ratio of the length of the side opposite an angle to the length of the hypotenuse in a right-angled triangle.
- Cosine (cos): Ratio of the length of the adjacent side to the hypotenuse in a right-angled triangle.
- Tangent (tan): Ratio of the length of the side opposite an angle to the length of the adjacent side in a right-angled triangle.
With these ratios in mind, let's explore how they're used in practical scenarios.
Problem 1: Finding the Height of a Building

Imagine you're standing some distance away from a building, measuring the angle of elevation to the top of the building to be 30 degrees. If your eye level is 1.5 meters from the ground and you are 10 meters from the base of the building, how tall is the building?

To solve this:
- We denote the height we're looking for as h.
- The height from the eye level to the ground can be added to our calculated height.
- We use the tangent ratio:
tan(30°) = opposite / adjacent tan(30°) = (h - 1.5m) / 10m h - 1.5 = 10m * tan(30°) h = 10 * (1/√3) + 1.5 ≈ 15.85 meters
📌 Note: Here, we use the value of tan(30°) = 1/√3 ≈ 0.577
Problem 2: Bridge Length Calculation
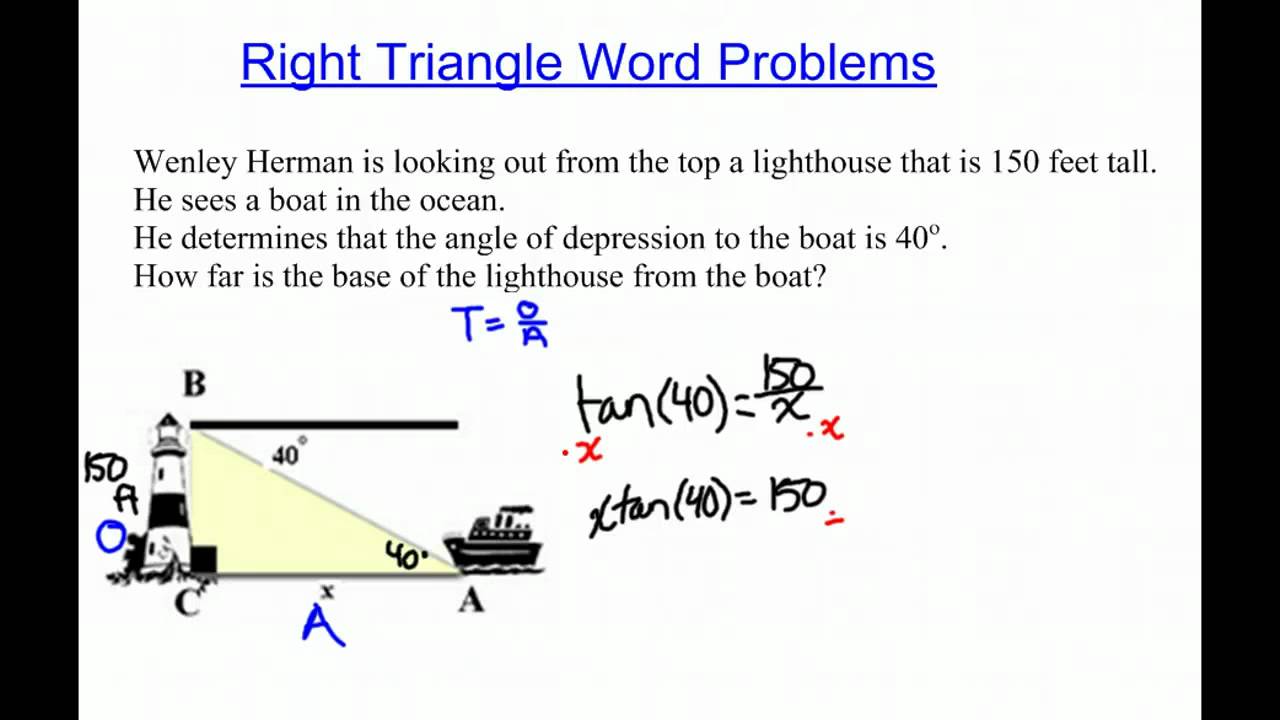
Consider a bridge that spans a river. From one end of the bridge, the angle of elevation to the top of a hill across the river is 25 degrees. If the bridge is 50 meters long, what is the vertical distance from the bridge to the hill?

To find the vertical distance:
- We denote the vertical distance as v.
- Using the sine ratio:
sin(25°) = v / 50 v = 50 * sin(25°) ≈ 20.74 meters
Problem 3: Ship Navigation

A ship departs from a port and sails north for 10 km before turning west at an angle of 60 degrees. How far is the ship from the port after its journey?

To solve for the distance from the port:
- Using the law of cosines:
c² = a² + b² - 2ab * cos(C) c² = 10² + (10 * cos(60°))² - 2 * 10 * (10 * cos(60°)) * cos(60°) c² = 100 + 25 - 50 c = √75 ≈ 8.66 km
📌 Note: Here, a and b are both 10 km, and C is the 60-degree angle between them.
Problem 4: Lighthouse Visibility

A lighthouse stands at the edge of a cliff. From a boat 30 meters away, the angle of elevation to the top of the lighthouse is 20 degrees. The cliff is 5 meters high. How tall is the lighthouse?

To find the height:
- We denote the lighthouse height above the cliff as h'.
- We use the tangent ratio:
tan(20°) = h' / 30 h' = 30 * tan(20°) ≈ 10.98 meters
The total height of the lighthouse is then h' + 5 ≈ 15.98 meters.
Final Thoughts on Applying Trigonometry

We've explored several scenarios where trigonometry aids in solving real-world problems. From calculating the height of structures to determining distances over land and sea, trigonometry provides the tools to make these calculations manageable. Understanding and applying these concepts not only strengthens your problem-solving skills but also enhances your appreciation for the mathematical beauty and utility in everyday life.
Why is trigonometry useful in geometry word problems?

+
Trigonometry helps solve problems involving angles, heights, distances, and direction, which are common in real-world applications. By understanding these relationships, one can calculate unknown lengths, heights, or directions, making it a powerful tool in geometry.
Can I use other methods to solve these problems?

+
Yes, problems in trigonometry can often be approached using different mathematical techniques like the Law of Sines, Law of Cosines, or even algebra, depending on the setup and known variables.
How do I choose which trigonometric ratio to use?

+
Choosing the right ratio depends on the given information. Use sine for sides opposite and adjacent, cosine for sides adjacent and hypotenuse, and tangent for opposite and adjacent sides. Sketching the triangle usually clarifies which ratio to apply.



