Day 1 Answer Key: X and Y Intercept Worksheet
Understanding x and y intercepts is crucial for visualizing and solving linear equations. Intercepts are the points where a line touches the x-axis and y-axis respectively. These points provide valuable insights into the behavior of lines and functions. In this detailed guide, we will explore how to find x and y intercepts, why they're important, and work through practical examples from a typical first-day worksheet on the subject.
What Are X and Y Intercepts?

The x-intercept of a line or curve is the point where it intersects the x-axis, which means the y-coordinate is zero. Conversely, the y-intercept is where the line or curve touches the y-axis, where the x-coordinate equals zero. Here’s how we can define them:
- X-intercept: This is found when y=0. You solve for x in the equation when y equals 0.
- Y-intercept: This is found when x=0. You solve for y in the equation when x equals 0.
How to Find X and Y Intercepts

Let’s break down the process into simple, manageable steps:
- Identify the Equation: Understand the equation you’re working with, usually in the form of y = mx + b or a linear equation like ax + by = c.
- Find the X-Intercept:
- Set y = 0 in the equation.
- Solve for x.
- Find the Y-Intercept:
- Set x = 0 in the equation.
- Solve for y.
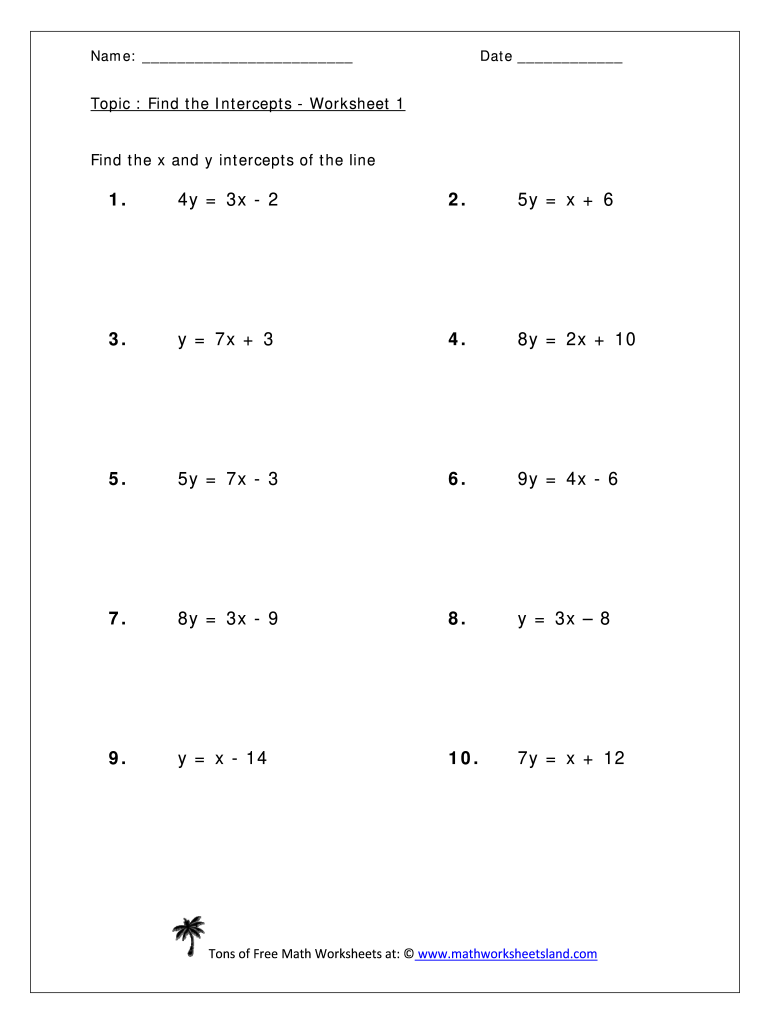
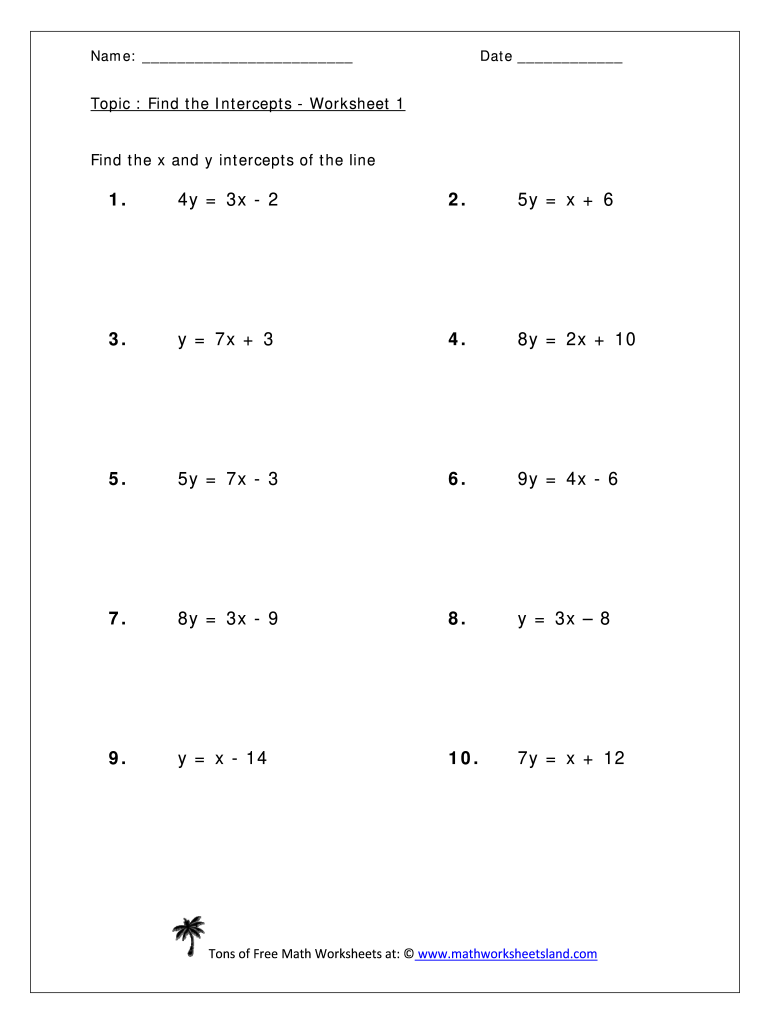
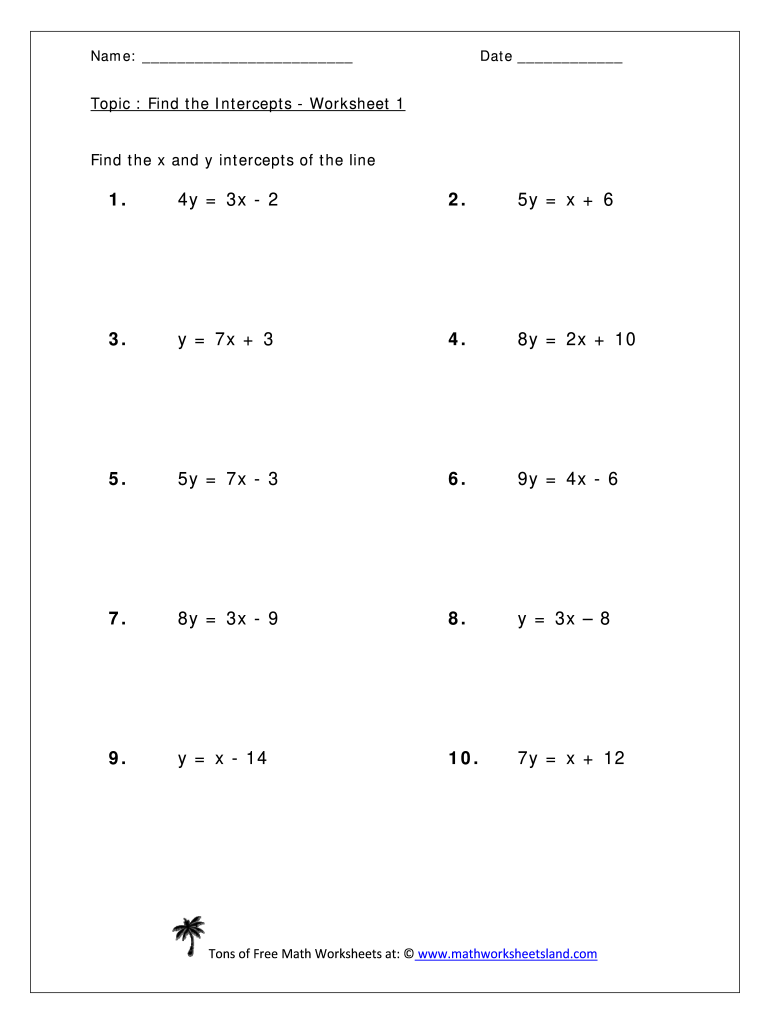
Example from Worksheet

Consider the following linear equation from a typical first-day worksheet:
- 2x + 3y = 6
To find the x-intercept:
- Set y = 0:
-
2x + 3(0) = 6
2x = 6
x = 3
The x-intercept is at (3, 0).
To find the y-intercept:
- Set x = 0:
-
2(0) + 3y = 6
3y = 6
y = 2
The y-intercept is at (0, 2).
💡 Note: This example assumes the line crosses both axes, which is not always the case for all linear equations.
Why Intercepts Are Important

- Graphing: Intercepts give you key points to start plotting a line or curve. Drawing a straight line through these points, along with others, helps visualize the equation graphically.
- Understanding Function Behavior: They help us understand where the function starts on the y-axis or where it touches the x-axis, which can indicate roots, solutions, or even the domain and range of functions.
- Solving Systems: When solving systems of linear equations, intercepts can give us insight into where lines might intersect or be parallel, helping solve problems visually or through substitution methods.
🚦 Note: Intercepts can indicate the direction of the line too, with the slope determining which quadrant the line points towards.
Additional Worksheet Examples

Here are a few more examples from the same worksheet to practice finding intercepts:
| Equation | X-Intercept | Y-Intercept |
|---|---|---|
| 5x + 2y = 10 | (2, 0) | (0, 5) |
| y = 4x + 4 | (-1, 0) | (0, 4) |
| 3x + 6 = 0 | (-2, 0) | (0, -6) |

Each equation provides its own unique challenges and showcases how diverse linear equations can appear, yet the process of finding intercepts remains consistent.
Summing Up
Understanding and calculating x and y intercepts are foundational skills in algebra, useful in graphing, problem-solving, and interpreting equations. They allow us to visualize how equations interact with the coordinate plane, providing immediate insight into the behavior of functions. This guide has provided you with the necessary tools to find these intercepts, explained their importance, and worked through several practical examples from a typical worksheet. Whether you’re sketching graphs, solving systems of equations, or preparing for more complex mathematical concepts, mastering intercepts is key.
Why do we need to find the intercepts of a line?
+
Intercepts help in quickly graphing the line, understanding the behavior of the function, and solving systems of equations. They provide key points for plotting and interpreting equations visually.
Can a line have no x or y-intercepts?

+
Yes, lines that are vertical or horizontal and do not intersect with the respective axes, or lines that lie entirely above or below one of the axes, can have no x or y-intercepts.
How do intercepts help in solving systems of equations?
+
By knowing the intercepts, we can quickly sketch the lines involved in the system and visually estimate or pinpoint where they might intersect, which helps in using methods like substitution or elimination to find solutions.
What if an equation has infinite intercepts?

+
In some special cases, particularly with equations of circles or parabolas, every point on the axis can be considered an intercept, which implies an infinite number of intercepts.



