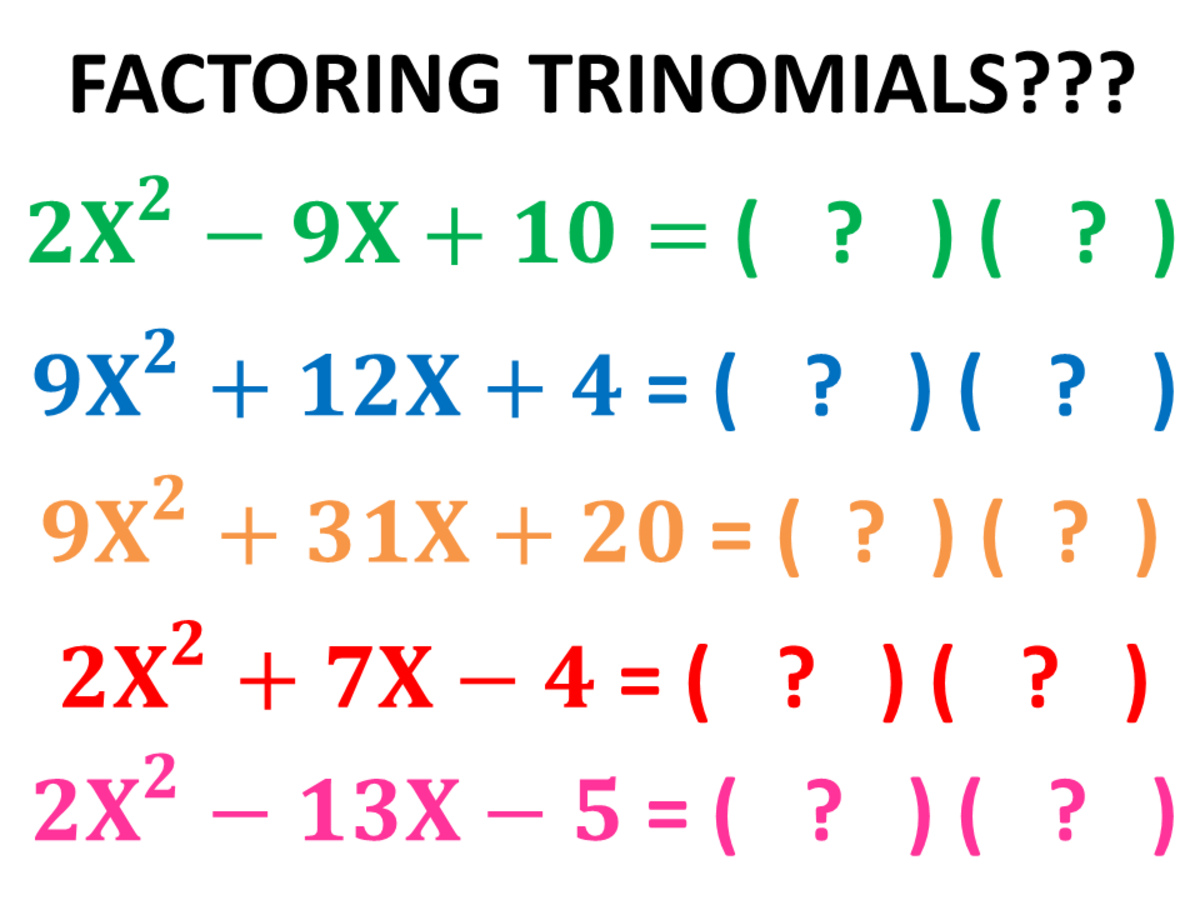
5 Tips for Factoring Quadratics with a Not 1

Solving quadratic equations is a fundamental skill in algebra, often required in mathematics, physics, and engineering courses. While the quadratic formula provides a universal solution, understanding how to factor quadratics where the coefficient of x2 is not equal to 1 can be particularly challenging yet rewarding. Here are five key tips to master this technique effectively.
Understand the Basics of Factoring

Before diving into complex factoring, ensure you have a solid grasp of the fundamental steps:
- Identify a (the coefficient of x2), b (the coefficient of x), and c (the constant term).
- Remember the general form: ax2 + bx + c = 0.
- Factor by grouping: Look for pairs that can be factored.
Tip 1: Use the “AC” Method

The AC method helps in factoring when a is not equal to 1:
- Multiply a and c.
- Find two numbers whose product is a * c and whose sum is b.
- Replace the middle term bx with the sum of these two numbers factored out.
📌 Note: This method transforms the quadratic into a form where factoring by grouping becomes straightforward.
Tip 2: Employ the Quadratic Formula

When other methods fail, the quadratic formula always works:
- The formula is: x = [-b ± √(b²-4ac)] / 2a.
- Use this when factoring seems too cumbersome.
- Once roots are found, express the quadratic as the product of binomials.
Tip 3: Use the Difference of Squares

If your quadratic expression can be written as the difference of squares, this method simplifies the factoring process:
- Identify if the quadratic can be expressed in the form a² - b².
- Factor it into (a + b)(a - b).
- Check if both factors can be further factored.
Tip 4: Factoring By Trial and Error

Although not the most systematic approach, trial and error can be effective:
- List possible factors of a and c.
- Try combinations to see which ones multiply to give ax2 and add/subtract to give bx + c.
- Keep track of successful pairs to avoid repetitive work.
Tip 5: Special Case - Perfect Square Trinomials
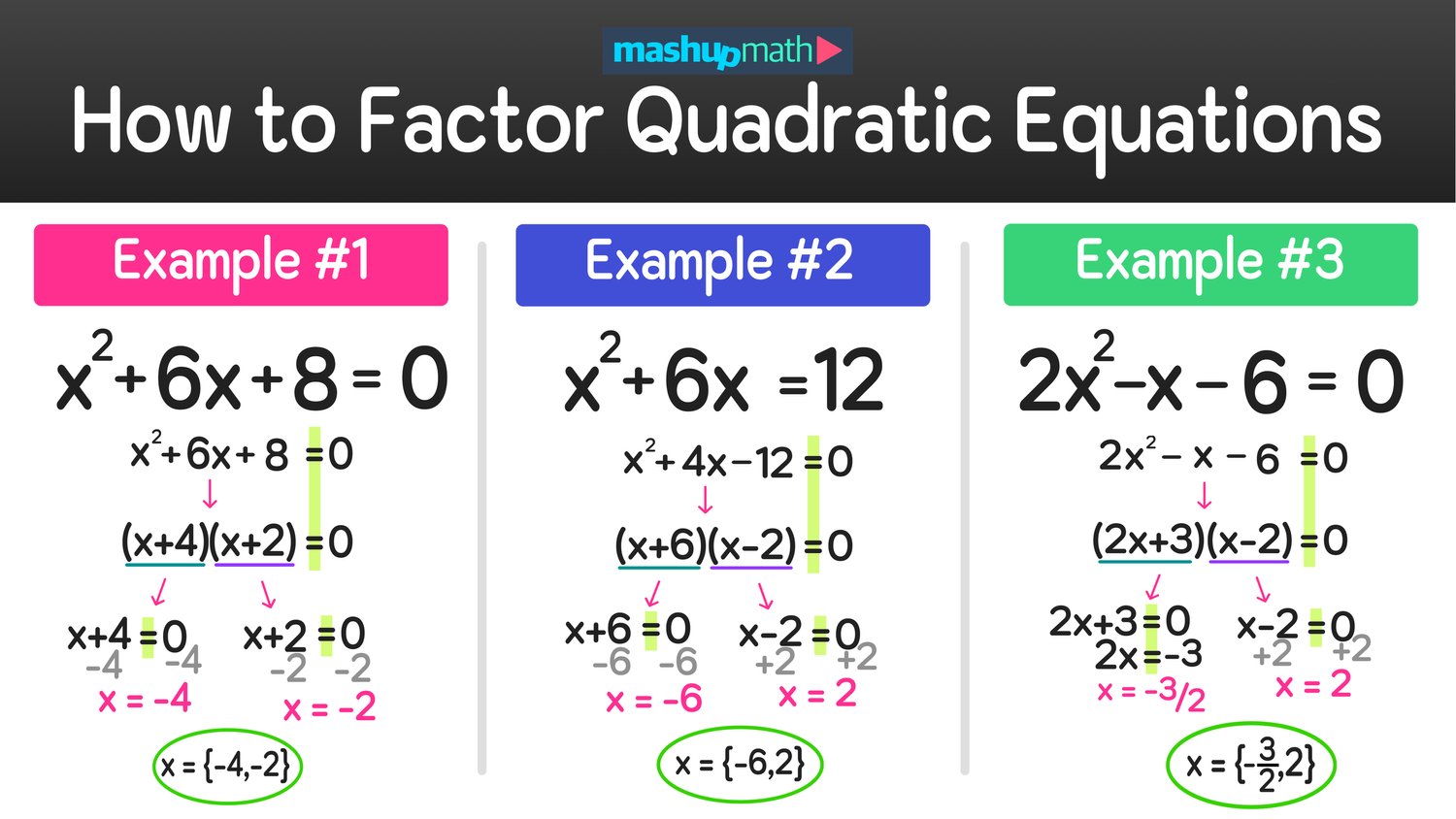
Identifying perfect square trinomials speeds up the factoring process:
- A perfect square trinomial is in the form of (a + b)² or (a - b)².
- Check if the quadratic follows this pattern.
- Factor it into (a + b)² or (a - b)².
To conclude, factoring quadratics when the leading coefficient is not 1 might seem daunting at first, but with these tips, you can master this skill. Remember, practice is key. The more you practice, the easier it will be to recognize which method suits each problem best. Factoring not only simplifies equations but also provides insights into the behavior of functions, which is invaluable in fields beyond mathematics.
What if the quadratic formula gives complex roots?
+
Complex roots in the quadratic equation indicate that the quadratic function does not cross the x-axis. However, you can still factor the quadratic over the complex numbers as (ax + (b+√(b²-4ac))/2a)(ax + (b-√(b²-4ac))/2a).
Why is it useful to factor quadratics?

+
Factoring allows you to find the roots of the equation, understand the equation’s graph, and simplify solving other mathematical problems that involve quadratics.
How do I know which method to use when factoring?

+
Experience will guide your choice, but here are some general guidelines:
- Use the AC method if the quadratic can be grouped easily.
- Consider the quadratic formula for complex problems or if other methods fail.
- Look for patterns like difference of squares or perfect square trinomials.