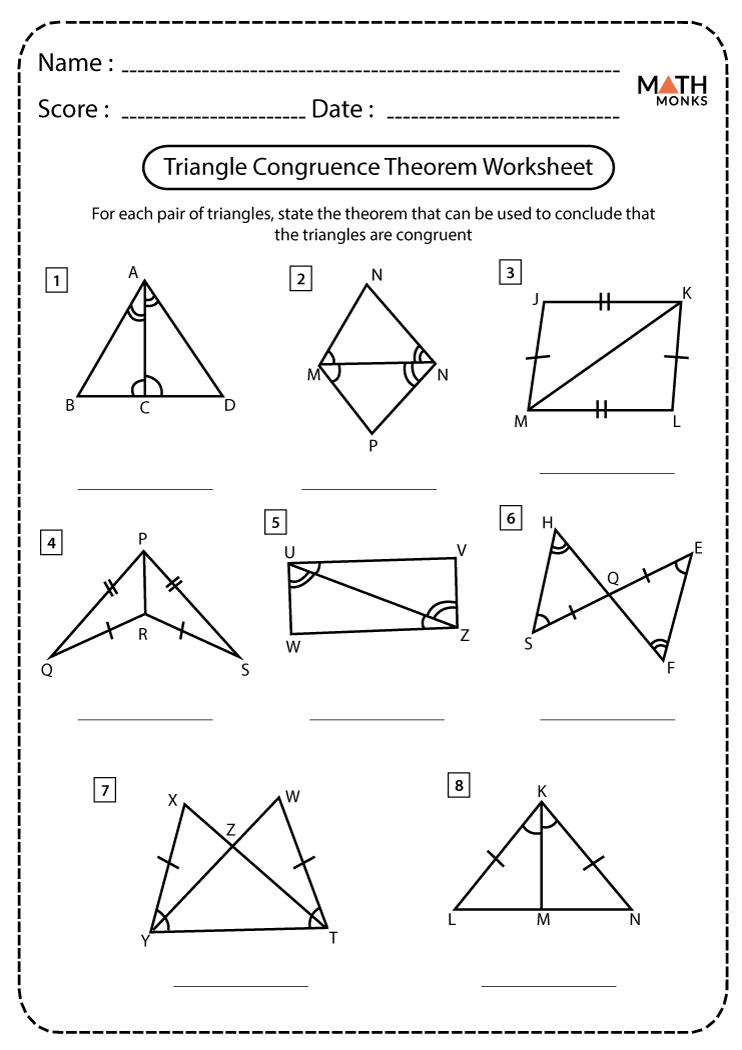
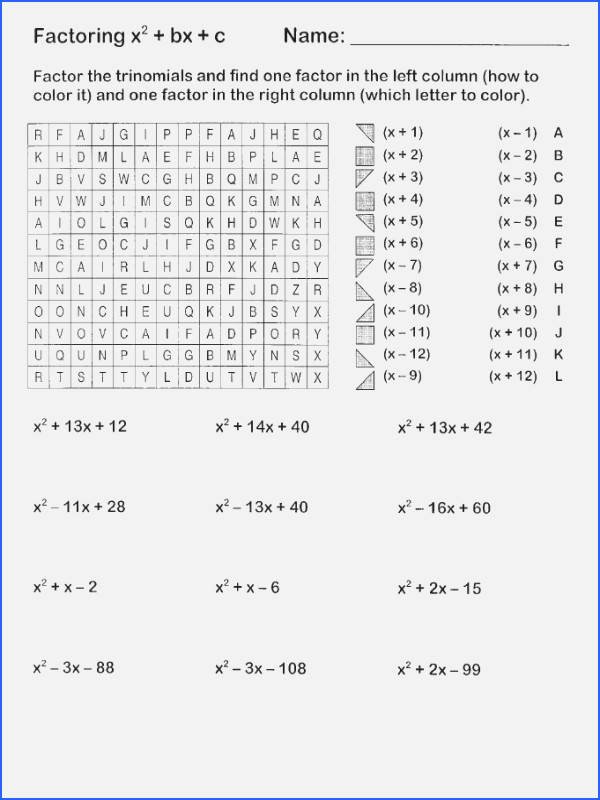
Factoring Polynomials GCF: Ultimate Worksheet Guide
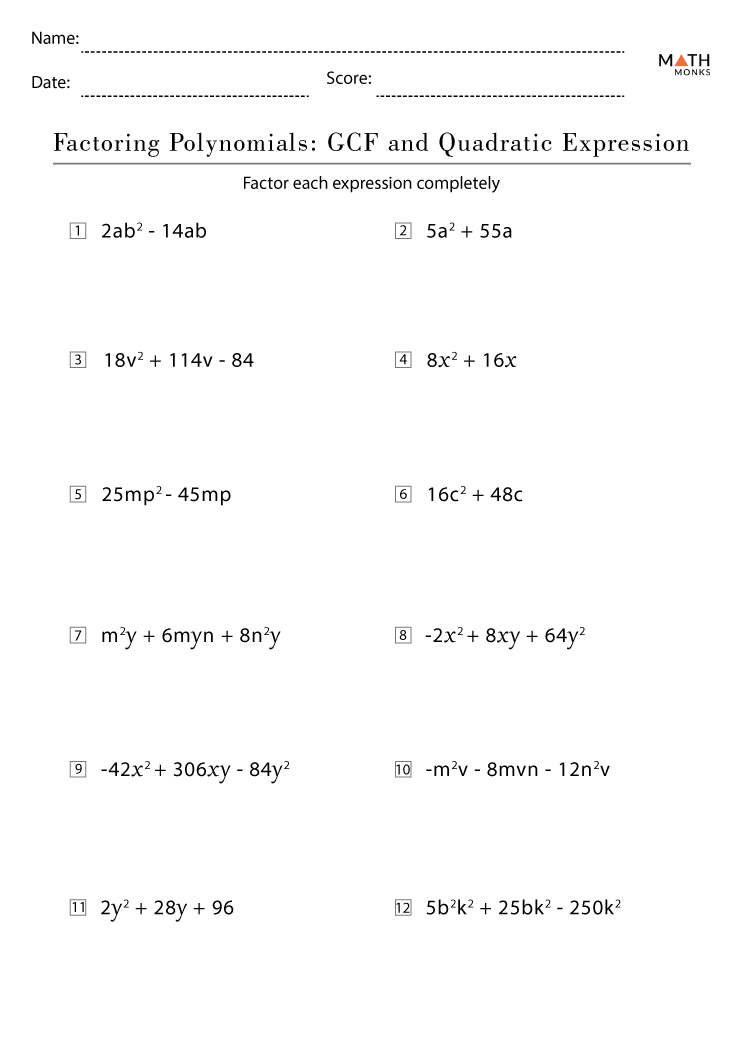
In the realm of algebra, understanding how to factor polynomials using the greatest common factor (GCF) method is a foundational skill. This guide will take you through the steps of factoring polynomials with the GCF, offering detailed explanations, examples, and practice worksheets to solidify your grasp on this crucial technique.
Understanding Factoring with GCF
Factoring involves breaking down a polynomial into simpler expressions that, when multiplied together, give the original polynomial. The GCF method focuses on extracting the largest factor common to all terms in the polynomial. Here’s how you can proceed:
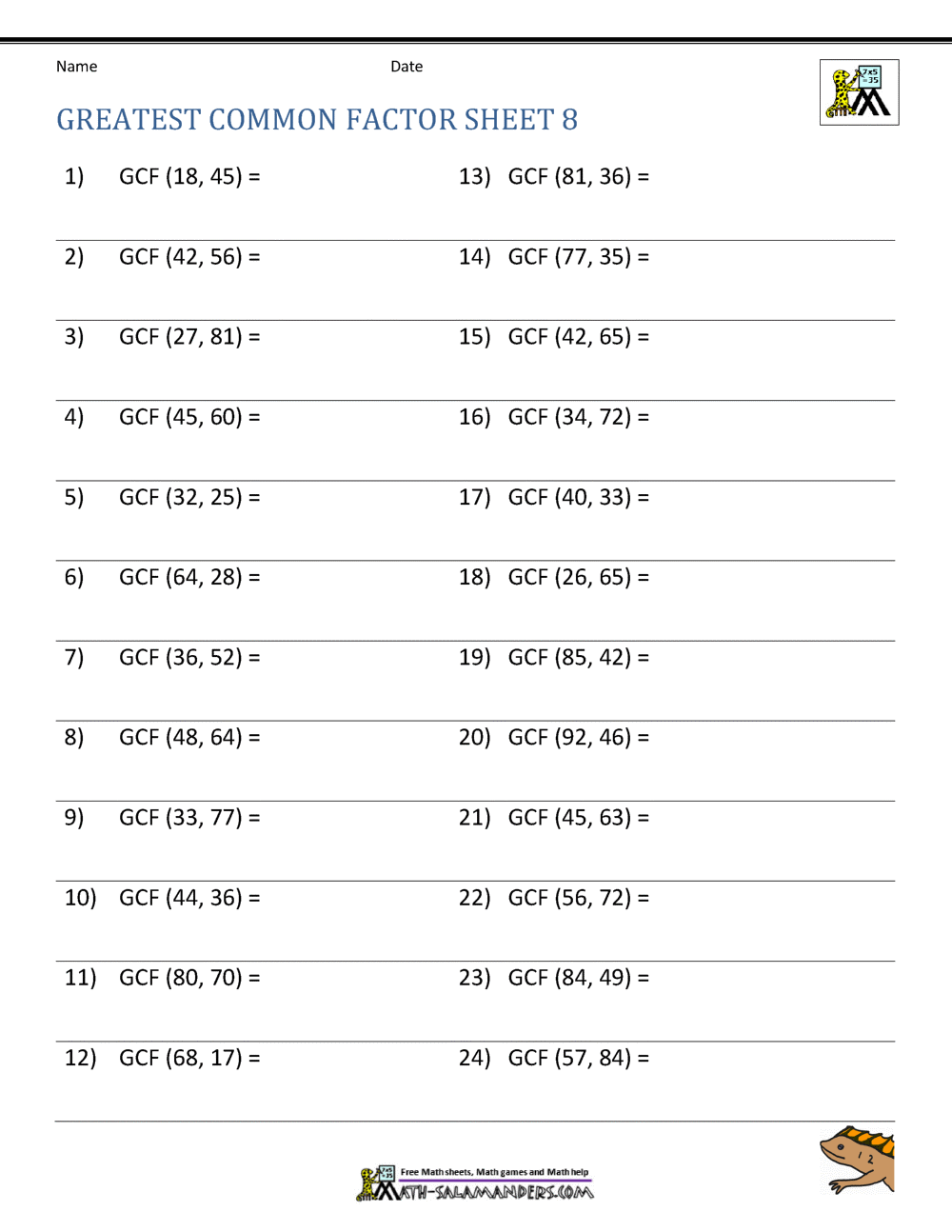
- Identify the GCF: Begin by finding the GCF of all the terms in the polynomial. This includes numbers, variables, and exponents.
- Factor Out the GCF: Divide each term in the polynomial by the GCF and write it outside the parentheses. The remaining terms go inside.
- Multiply: Ensure the factored form can be multiplied back to yield the original polynomial.
Steps for Factoring Polynomials Using GCF
Step 1: Identify the GCF
To find the GCF:
- List all factors of the coefficients.
- Identify the common prime factors.
- Use the lowest power of common variable factors.

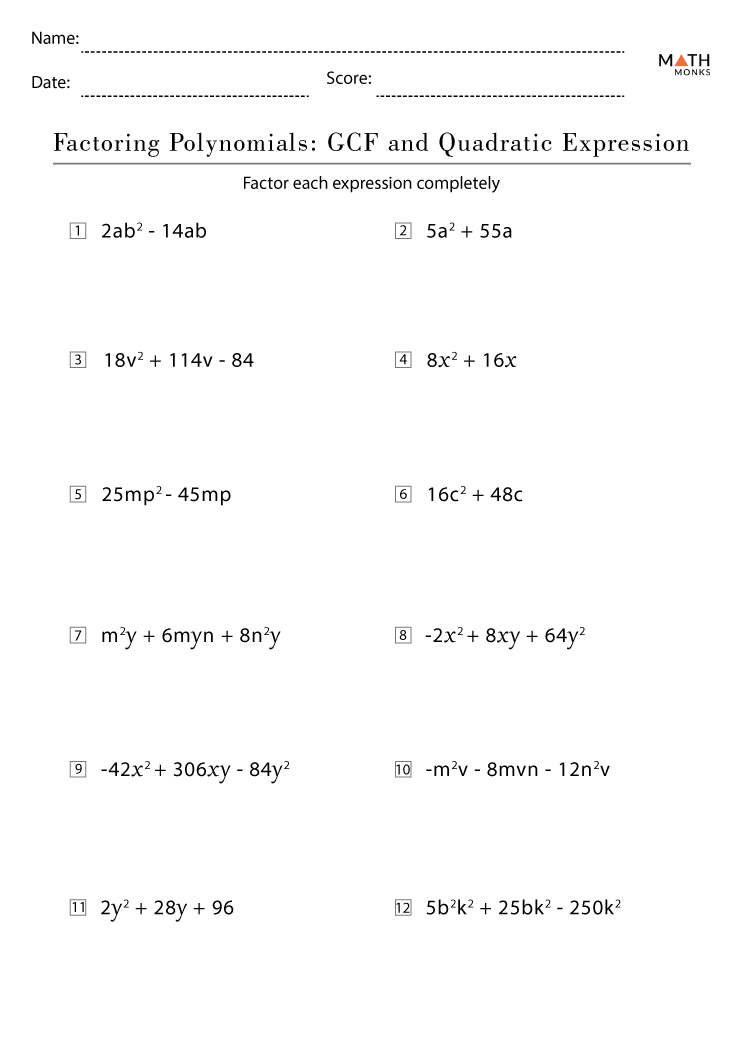
Step 2: Factor Out the GCF
Once you have the GCF:
- Divide each term in the polynomial by the GCF.
- Place the GCF outside the parentheses and the resulting polynomial inside.
🔍 Note: Remember to write the polynomial in its simplest form inside the parentheses.
Step 3: Verify Your Factorization
To ensure accuracy:
- Multiply the factored expression back to check if it matches the original polynomial.

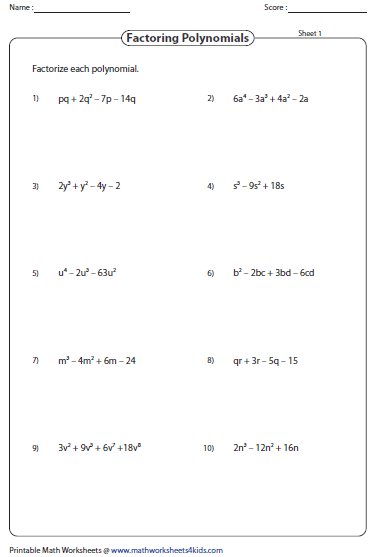
Worksheet for Practicing Factoring with GCF

To master factoring polynomials, here is a worksheet to practice:
| Polynomial | Factored Form |
|---|---|
| 2x2 + 8x | 2x(x + 4) |
| 15x3 - 35x2 | 5x2(3x - 7) |
| 12a3b2 - 4ab | 4ab(3a2b - 1) |
| 7x4 - 14x3 + 21x2 | 7x2(x2 - 2x + 3) |
| 3y5 - 6y4 + 12y3 | 3y3(y2 - 2y + 4) |

Advanced Techniques

As you progress, you might encounter polynomials where factoring by GCF isn’t immediately obvious. Here are some advanced tips:
- Factoring by Grouping: Sometimes, rearranging terms can make factoring easier.
- Use of Substitution: Replacing complex parts with a single variable can simplify the process.
- Recognizing Special Products: Patterns like perfect squares or cubes can be quickly factored.
The mastery of factoring polynomials with the GCF method is not just about solving equations; it’s a key to unlocking further algebraic skills like simplifying rational expressions or solving equations with multiple terms. By understanding how to extract common factors, you open up the door to a deeper understanding of algebraic structure.
What is the Greatest Common Factor (GCF)?

+
The Greatest Common Factor (GCF) is the largest factor that divides two or more numbers or terms without leaving a remainder. In polynomials, this applies to both coefficients and variable terms.
Why is factoring polynomials important?

+
Factoring polynomials helps in simplifying complex expressions, solving equations, and understanding the structure of polynomials, which is crucial for advanced algebraic operations.
Can all polynomials be factored using GCF?
+
Not all polynomials can be factored using GCF; some require different methods like grouping, difference of squares, or synthetic division. GCF is just one of many factoring strategies.